Help w/ Subspaces Questions for Assignment Due Monday

[mmmboh]

Subspaces Questions Help Please!

Hi I have an assignment due Monday morning and there are a few questions I am not sure about or if I proved them properly:

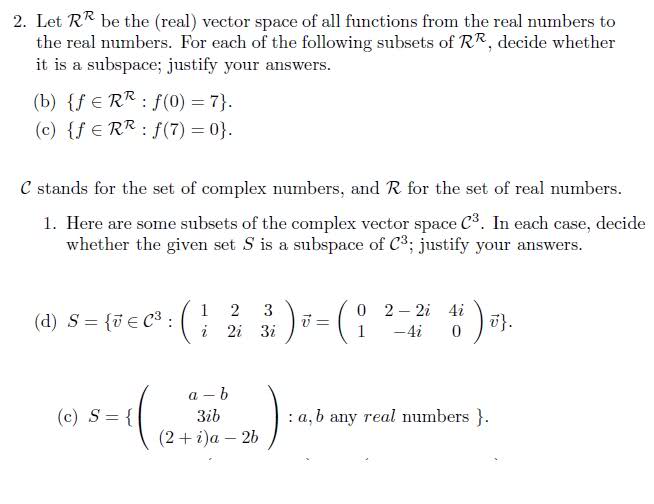
Ok so for 2b) I said that it is not a subspace because f(x)=7 when x=0, and this function never equals zero, and since this is included in the vector space the subset is not a subspace.

For 2c) I wrote it clearly has the zero vector because f(7)=0. and (f+g)(x)=f(x)+g(x), and so the subset is closed under addition, and that (Kf)(x)=k(f(x)), so it is closed under scalar multiplication and thus the subset is a subspace.

For the third question,I wrote it is closed under scalar multiplication because AKV1=AKV2 (K is a scalar), and i wrote that A(V1+V2)=AV1+AV2=BV1+BV2 and is thus closed under addition and so the subset is a subspace. (and I wrote it clearly has the zero vector).

For the fourth one I wrote that the subset is not a subspace because it is not closed under scalar multiplication because you can multiply it by i and then you get complex numbers.

Please help, it is very appreciated :)

 

[vela]

Ok so for 2b) I said that it is not a subspace because f(x)=7 when x=0, and this function never equals zero, and since this is included in the vector space the subset is not a subspace.

I think you're not clear on what the zero vector is in this case. Remember the vectors are the functions from R to R. The zero vector is the function 0(x)=0, not x=0.

 

[mmmboh]

Yeah we haven't done any examples with functions in class, I am pretty confused by it :S...so that means the zero vector is included right? and by the same reasoning it is included in the second question too?

 

[VeeEight]

You should write out your answers more neatly and in proper notation since it's hard to understand some times. You need to write out the criteria for being a subspace and for each question, show these 3 criteria hold or where one of them fails. For example, for the first question, if for f, g functions on R f(0)=7 and g(0)=7, does it follow that , (f+g)(0)=7?

 

[mmmboh]

Hm..I think (f+g)(0)=f(0)+g(0)=7+7=14, and so it is not a subspace. Is this correct?

Sorry about the notation, I am at the library and it is closing now but when I get home I will try to write it better.

 

[vela]

Yeah we haven't done any examples with functions in class, I am pretty confused by it :S...so that means the zero vector is included right? and by the same reasoning it is included in the second question too?

No, it's not in the first set because the zero function evaluated at x=0 is 0, but the set only contains functions that equal 7 at x=0. On the other hand, the second set contains functions that equal 0 when x=7. The zero function obviously satisfies that criterion, so it is in the second set.

To show that the first set isn't closed under scalar multiplication, note that for k \ne 1

(kf)(0) = k[f(0)] = k7 \ne 7

Because kf evaluated at x=0 is not equal to 7, it's not in the set.

Your explanation for the second set needs to be cleaned up a bit. You should explain explicitly why f+g and kf are in the set.

 

[vela]

Hm..I think (f+g)(0)=f(0)+g(0)=7+7=14, and so it is not a subspace. Is this correct?

Yes, though I would say f+g is not in the set, rather than referring to it as a subspace, because the set is apparently not a subspace.

 

[mmmboh]

No, it's not in the first set because the zero function evaluated at x=0 is 0, but the set only contains functions that equal 7 at x=0.

What if the function was f(x)=7-x, and so at x=0 f=7, but when x=7 f=0, wouldn't this include a zero vector?

And are the 3rd and fourth ones right?

Thanks for the help guys.

 

[vela]

What if the function was f(x)=7-x, and so at x=0 f=7, but when x=7 f=0, wouldn't this include a zero vector?

No, you're not understanding what the zero vector is in this problem. The zero vector is the function that maps all real numbers to 0. It's the additive identity element, the function you can add to any other function that leaves it unchanged, i.e. (f+0)(x) = (0+f)(x) = f(x).