- #1
fvalentin
- 1
- 0
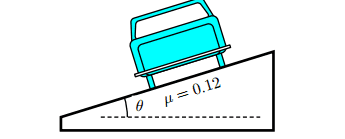
So I'm having a hard time understanding baked turns involving roads. Mainly I'm confused on how to derive the friction force into the x and y components and why it turns be like so. I also am confused about when i encounter a problem that involves friction in banked turns like in the picture below. Ignore the coefficient of friction; I'm just trying to understand the physics of it.