Help with My Physics Test: Find Velocity, Distance & Acceleration

[Obelix]

I understand that I should be following a prompt, but I feel my explanations and questions are clear and concise (enough).

On Friday, I had a physics test that was hard as _____. The teacher ended up letting us take it home but even with that I don't have any new magical knowledge on how to figure these problems out. I'm here to once again ask you smarter minds for help. I've tackled ALL of these problems in a 1 hour 46 minute period and I am not confident with ANY of my answers on ANY problem.

Help please.

Number 1
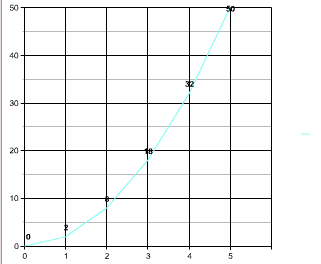
You observe a gooey object falling from a 55 meter high tower (a big turkey nests on the tower) toward a hapless old woman feeding the pigeons from a park bench at the base of the tower. The tower has meter marks on it and you record the time of the falling object as it falls. You make the following data table.

Time (s) Distance (m)
0 0
1 2
2 8
3 18
4 32
5 50

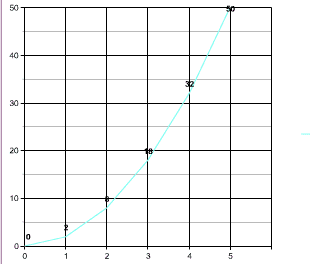
Plot this data - like you know how to (graph above). Find the instantaneous velocity of the object at the time of 0 seconds, 1 second, and 4 seconds. (below this paragraph) Plot the instantaneous velocities on a new graph. Calculate acceleration. Plot acceleration on a new graph. Determine a general formula for velocity. Determine a general formula for distance.

These are the numbers I got for instantaneous velocity.

0 seconds = 0 m/s
1 second = 4.67 m/s
4 seconds = 14 m/s

They seem a bit off, but I guess they're okay.

I don't understand how to plot the instantaneous velocities. I got something like this:

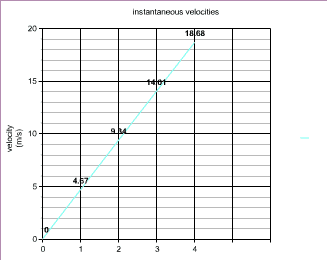
It's wrong though huh?

Acceleration out of nowhere I got 7.5 m/s^2

For my V average formula, I got v = at
for my d formula, I got d = 1/2 at^2
Number 2:

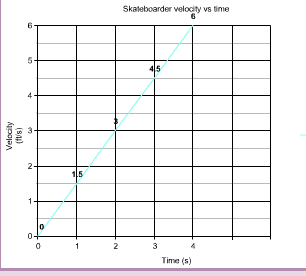
Your friend skateboards straight down Twin Pillar Way. Your friend is wealthy and has a speedometer. He records this graph above. Using the graph, determine acceleration, plot the acceleration, determine an equation for velocity, and determine an equation for distance.

For acceleration, I got 1.5 ft/s^2 and I plotted it. If that's wrong I may as well jump off my roof now.

The determining equations part is eluding me. Also, are formulas the same things as equations? <_<Number 3:

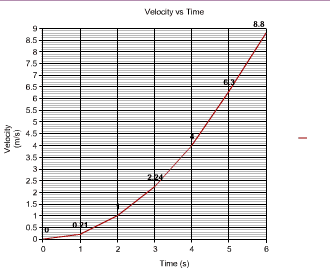
A rocket is launched and its velocity is measured at over 6 one-second time intervals. What are the instantaneous accelerations at t = 0, t = 2 seconds, and t = 4 seconds? Plot the acceleration vs time. What is the rate of change of acceleration? Determine a general formula for the acceleration. Don't forget to include proper units.

Here are my instantaneous accelerations

T = 0: 0 m/s^2
T = 2: 1 1/10 m/s^2
T = 4: 6 m/s^2

Here's my acceleration vs time graph, which is horribly deformed.

What's wrong with it?

This also makes finding the rate of change of acceleration an abnormal task, as well as finding a general formula for acceleration D:

Number 4

You had to resort to passing an elderly Sunday Driver while you were riding your bicycle. What was your acceleration over the 6 seconds? Plot acceleration. What is your overall distance you traveled during your passing the Sunday Driver (over 6 seconds)? Determine a velocity over time equation. Determine a distance over time equation.

Acceleration is 1 1/2 m/s^2...hopefully?
Acceleration is easy to plot, thank god.
I said that the overall distance he traveled was 9 feet...right?

My mind exploded when I had to determine more equations, so those I didn't do @_@

Number 5

You shoot a marble with a slingshot straight up. The marble has an initial velocity of 125 m/s up. What is the velocity of the marble at its highest point? How high does the marble travel? What is the acceleration of the marble at its highest point? What is the distance the marble travels at 15 seconds?

My mind again shat itself to death so this problem was not even attempted.


Number 6

You have a friend who you consider to be not very smart; and he wants to base jump off of a 400 meter high building today. He says his parachute will open in 10 seconds. What is the distance the base-jumper will free fall without an open parachute. What will his speed be at 5 seconds. How far will he have fallen after 2 seconds? What is his acceleration? Will he live?

Assuming gravity = 10 m/s^2, I said he would fall 100 meters before opening his parachute.

At 5 seconds, his speed will still be 10 m/s^2. (Or is it 50 m/s^2)

2 seconds = 20 meters fallen (<_<). Acceleration = 20 m/s^2

Yes, he'll live.Again, I am not asking anybody to do all of these, are even do them at all. Any helpful guidance, maybe a link that will prove useful, ANYTHING is appreciated. If I get 70% right I'll be happy.

Merci beaucoup.

 

[lewando]

Have you familiarized yourself with the basic kinematic equations? Is there a particular promblem that you would like us to focus on first? One way to become good at solving these types of problems is to do a lot of them--starting with lots of easy ones and then taking on more challenging ones. Sometimes you may have to go into a sort of "double- full monk mode" to be able to focus your mind enough to attempt these types of problems. When is this take home test due?

 

[Delphi51]

Your #1 looks pretty good! You don't say how you are getting the velocities. Are you finding the slopes of tangents on the distance graph?
The acceleration will be the slope on the velocity graph - easy because it is a straight line: a = rise/run = 18.7/4 = 4.7

For #2, your acceleration is correct! To get the equation for velocity you could just remember the formula: V = Vi + at = 0 + 1.5*t.
Or you could recall that the velocity is the area under the acceleration graph. That graph is a rectangle because the acceleration is constant at 1.5 for all times. Thus the area up to time t would be
A = L*W = 1.5*t.

In #3, your accelerations don't seem to be right. I'm imagining a tangent line at time 4 and it is going from about 2 m/s to 6 m/s in 2 seconds from time 3 to 5, which would give a slope (acceleration) of
slope = rise/run = (6-2)/(5-3) = 2 m/s^2. The acceleration graph should work out to be a straight line.

#4 - acceleration is correct! The distance is not. Using the method where distance is the area under the velocity graph, you get a trapezoid with average height (2 + 11)/ 2 = 6.5 and width 6. That is an area of 6.5*6 = 39. Using the formula d = Vi*t + .5*a*t^2
= 2*6 + .5*1.5*6^2 = 12 + 27 = 39.

In #5, you know the acceleration is -9.81, so you can use the
d = Vi*t + .5*a*t^2 and V = Vi + at formulas to find out the
velocity and distance at various times to get an overall picture of what happens.

 

[Obelix]

It's due on the 12th - Monday.

Number one is a particularly hard problem, especially graphing instantaneous velocity as well as finding the formula for velocity and distance.

I'm fairly familiar with the kinematic equations, though I still haven't memorized any besides v = vi + at.

 

[Obelix]

Your #1 looks pretty good! You don't say how you are getting the velocities. Are you finding the slopes of tangents on the distance graph?
The acceleration will be the slope on the velocity graph - easy because it is a straight line: a = rise/run = 18.7/4 = 4.7

Yes, I found the velocities by looking at the slopes of the tangents.

For #2, your acceleration is correct! To get the equation for velocity you could just remember the formula: V = Vi + at = 0 + 1.5*t.
Or you could recall that the velocity is the area under the acceleration graph. That graph is a rectangle because the acceleration is constant at 1.5 for all times. Thus the area up to time t would be
A = L*W = 1.5*t.

In #3, your accelerations don't seem to be right. I'm imagining a tangent line at time 4 and it is going from about 2 m/s to 6 m/s in 2 seconds from time 3 to 5, which would give a slope (acceleration) of
slope = rise/run = (6-2)/(5-3) = 2 m/s^2. The acceleration graph should work out to be a straight line.

#4 - acceleration is correct! The distance is not. Using the method where distance is the area under the velocity graph, you get a trapezoid with average height (2 + 11)/ 2 = 6.5 and width 6. That is an area of 6.5*6 = 39. Using the formula d = Vi*t + .5*a*t^2
= 2*6 + .5*1.5*6^2 = 12 + 27 = 39.

In #5, you know the acceleration is -9.81, so you can use the
d = Vi*t + .5*a*t^2 and V = Vi + at formulas to find out the
velocity and distance at various times to get an overall picture of what happens.

Thanks a lot for the help as well as the optimism, it really makes me feel like I can work these out (as corny as that sounds).

I'll tackle the problems tomorrow morning and post my results. I feel that sleeping early might be more helpful than staying up late and getting frustrated from exhaustion. ;x

 

[Delphi51]

Good luck, Obelix. There aren't all that many techniques going on here:
acceleration
velocity
distance
Have to find the slope on the graph to go up on the chart.
Find the area under the graph to go down on the chart.
If you don't know how to draw the tangent line, get some help.