Hi determine the height of the mountain (using atmospheric pressure)

[Krokodrile]

Homework Statement

determine the height of the mountain from the data shown. Consider the average air density of 0.074 lbm and g= 32 ft/s^2

Relevant Equations

p = p*g*h

i have the first convertions of the atmospheric pressure:
1872 lbf/ft^2 = 89,632.6 Pa
2016 lbm/ft^2 = 96,325.4 Pa
g= 9.72 m/s^2

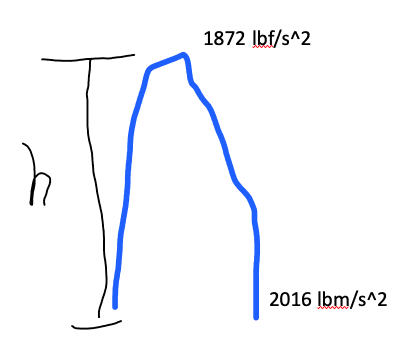
But, i don't have idea how pass the air density of "lbm" to international units ;,(. And other cuestion: its fine pass lbm/ft^2 and lbf/ft^2 to Pa simirlarly?

 

[Orodruin]

The units are all over the place here. Is this the actual problem statement word for word and is the image from the problem?

• Density should have units of mass/length^3, but lbm is a unit of mass only.
• The units in the picture show lbf/s^2 and lbm/s^2 for the pressures. These are wrong as the dimensions of pressure are force/area while lbf/s^2 is force/time^2 and lbm/s^2 is mass/time^2.

I'd really recommend getting the input units correct before doing anything else.

 

[Krokodrile]

The units are all over the place here. Is this the actual problem statement word for word and is the image from the problem?

• Density should have units of mass/length^3, but lbm is a unit of mass only.
• The units in the picture show lbf/s^2 and lbm/s^2 for the pressures. These are wrong as the dimensions of pressure are force/area while lbf/s^2 is force/time^2 and lbm/s^2 is mass/time^2.

I'd really recommend getting the input units correct before doing anything else.

yes, i wrong in the densty, its lbm/ft^3. yes, the units of pressures its lbf/ft^2 (top) and lbm/ft^2(bottom) (my mistake, sorry). But, i too have the question about these units: why lbf and lbm?

 

[Orodruin]

Ok, the lbm/ft^2 is probably a mistake on the problem writer's part. It should likely be lbf/ft^2, which is the correct units for pressure (force/area).

Regarding your question on lbm, 1 lbm is defined as the mass for which the gravitational force in standard Earth gravity is 1 lbf. Since F = mg, 1 lbf = (1 lbm)g or in other words: 1 lbm = (1 lbf)/g. If you convert the 1 lbf to N and insert the appropriate value for g you will get the numerical value for 1 lbm expressed in kg. In order to get the SI units of kg/m^3 for the density, you will of course also have to convert the ft^3 to m^3.

 

[Krokodrile]

Ok, the lbm/ft^2 is probably a mistake on the problem writer's part. It should likely be lbf/ft^2, which is the correct units for pressure (force/area).

Regarding your question on lbm, 1 lbm is defined as the mass for which the gravitational force in standard Earth gravity is 1 lbf. Since F = mg, 1 lbf = (1 lbm)g or in other words: 1 lbm = (1 lbf)/g. If you convert the 1 lbf to N and insert the appropriate value for g you will get the numerical value for 1 lbm expressed in kg. In order to get the SI units of kg/m^3 for the density, you will of course also have to convert the ft^3 to m^3.

I have all the units in international system, but i can't eliminate the s^2 of gravity and obtain m:

 

[Orodruin]

The unit Pa is not kg/m^2 [mass/area], it is N/m^2 [force/area], which equates to (kg m/s^2)/m^2 = kg/(m s^2).

 

[Krokodrile]

The unit Pa is not kg/m^2 [mass/area], it is N/m^2 [force/area], which equates to (kg m/s^2)/m^2 = kg/(m s^2).

Oohh, thanks for that, now i can eliminate the units, i don't know if i was bad because i got 1/m in the result:

 

[Chestermiller]

I would do this differently. 2016 - 1872 = 144 psf

144/0.084= 1714 ft

 

[Krokodrile]

I would do this differently. 2016 - 1872 = 144 psf

144/0.084= 1714 ft

in this case why the gravity was omited? and why 0.084 and not 0.074?

 

[Chestermiller]

in this case why the gravity was omited? and why 0.084 and not 0.074?

I misread the 0.074 as 0.084.
In imperial units, 1 lbm weighs 1 lbf. In these units, F = ma/g

 

[Krokodrile]

I misread the 0.074 as 0.084.
In imperial units, 1 lbm weighs 1 lbf.

I have difference of 11.92 m with my answer, i must check it again. The gravity was omited because the mountain its not afected by her? i don't understand why in 144/0.074 the gravity does``````nt appears :(

 

[Chestermiller]

I have difference of 11.92 m with my answer, i must check it again. The gravity was omited because the mountain its not afected by her? i don't understand why in 144/0.074 the gravity does``````nt appears :(

lbm is not the proper unit to use with such units . The correct unit to use is the slug, which is lbm/32.2. In this way, the 32.2’s cancel out.

 

[Chestermiller]

Suppose I defined 1 kgf as the weight of a 1 kg mass. How many Newton’s would that be?

 

[Krokodrile]

Suppose I defined 1 kgf as the weight of a 1 kg mass. How many Newton’s would that be?

9.80? as 1 kgf = 9.80N

 

[Krokodrile]

lbm is not the proper unit to use with such units . The correct unit to use is the slug, which is lbm/32.2. In this way, the 32.2’s cancel out.

if the slug 32.2s, its fine to cancel it with 32.0?

 

[Chestermiller]

if the slug 32.2s, its fine to cancel it with 32.0?

That was just rounded off to 32.

 

[Krokodrile]

That was just rounded off to 32.

then it would be something like this?:

 

[Gordianus]

Ah...The Imperial System...What was the name of that doomed satellite?

 

[Krokodrile]

Ah...The Imperial System...What was the name of that doomed satellite?

Mars Global Surveyor satellite?

 

[Chestermiller]

then it would be something like this?:
View attachment 280215

It should be $$\frac{144\ lb_f/ft^2}{0.074 \frac{lb_m}{ft^3}(32.2\ \frac{ft}{s^2})\left(\frac{1}{32.2\ \frac{lb_mft}{lb_fs^2}}\right)}$$

 

[Krokodrile]

It should be $$\frac{144\ lb_f/ft^2}{0.074 \frac{lb_m}{ft^3}(32.2\ \frac{ft}{s^2})\left(\frac{1}{32.2\ \frac{lb_mft}{lb_fs^2}}\right)}$$

thank you so much. I understand all the process, except for the 1/32: where does it come lbm/lb?

 

[Chestermiller]

thank you so much. I understand all the process, except for the 1/32: where does it come lbm/lb?

It is the factor that converts lbm to slugs. Those units are the same as lbm/slug

 

[Krokodrile]

It is the factor that converts lbm to slugs. Those units are the same as lb/slug

so, the ft/s^2 its the slug units and lbm/lb is the lfbm to slug factor?

 

[Chestermiller]

so, the ft/s^2 its the slug units and lbm/lb is the lfbm to slug factor?

$$32.2 lb_m=1\ slug= 1 \frac{lb_f s^2}{ft}$$

 

[Krokodrile]

$$32.2 lb_m=1\ slug= 1 \frac{lb_f s^2}{ft}$$

oooohhh, and 1/32 its because we can eliminate with the other 32. Ok, i get it. Thank you so much again for your time, Sir.

 

[Orodruin]

Oohh, thanks for that, now i can eliminate the units, i don't know if i was bad because i got 1/m in the result:
View attachment 280209

You just did the cancellation of the length units wrong. Nominator has one negative power of length, denominator has three negative and one positive power of length so two negative powers overall, thus the power of length in the final result is one positive power of length, i.e., meters.