Home project: Kilogram Force on wheel bearings

[DC81]

TL;DR Summary

Weight triangulation to work out load ratings for bearings

Hi all. I'm designing a custom spare wheel carrier for my 4wd and currently seeking knowledge on which bearings to use, so I need to work out what force on the bearings will be when it's open (closed will be locked and fully supported!)

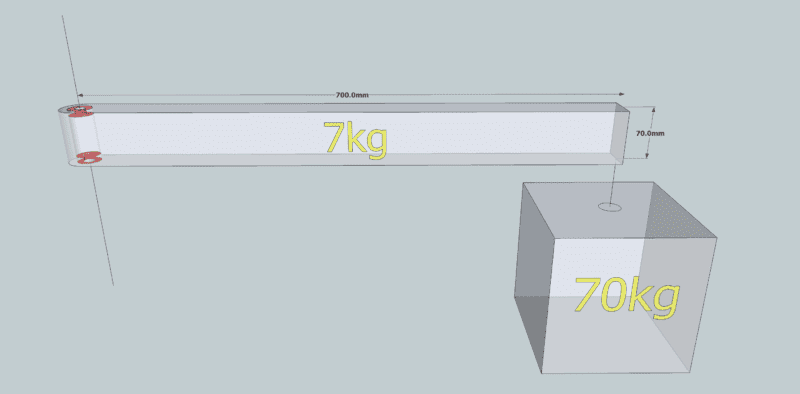
The example image is simplified for calculation. Close enough is good enough because the wheel itself would be no more than 25kg and I'm allowing 70kg to play it safe.

See below for details...

Thanks in advance folks, much appreciated!

 

[BvU]

Clear first post ! My compliments !

Here's what I make of the situation:

$F_1$ is the force that the upper bearing exerts on the beam. $F_2$ idem, lower.
Vertical and horizontal components are $F_{1y},\ F_{1x},\ F_{2y},\ F_{2x}$ .
These four are your unknowns. Magnitude of $F_1$ and $F_2$ follows from $F_1^2 = F_{1y}^2+ F_{1x}^2$. Similar for $F_2$.

In equilibrium there is no acceleration of the whole lot, so the sum of forces is zero.
In equilibrium there is no rotation of the whole lot, so the sum of torques is zero.

Forces:
In horizontal direction: $$F_{1x}+F_{2x}=0$$
In vertical direction: $$F_{1y}+F_{2y}+Mg + mg=0$$

Torque, e.g. about point O:$$F_{1x}\times L_3 + mg\times L_1+Mg\times L_2 = 0$$

That's three equations for four unknowns.
A sensible fourth equation is to assume $F_{1y}=F_{2y}$.


[edit] $g$ is $-9.8$ m/s² in the upward direction and I updated(*) the equations accordingly. Hence the $+$ signs.

(*) i.e. corrected the torque which now has signs consistent with the signs in the sum of force equations...

$\$

 

[DC81]

Thanks for the reply but I don't understand a single piece of it

Was that copied and pasted from another post or was it specific to this post, I don't see any kgf, Newton or kg's and now more confused.

I thought it would be a simply triangulation of weights.


Here's a simplified example

The top circle would have a pull force from the right, the bottom circle would have push force to the left.

Would I be safe to say this is correct?

I'm trying to understand the basics first

 

[Baluncore]

Welcome to PF.
Is the axis through the two hinges vertical or horizontal?

If vertical, the bearings will have an axial force equal to 70 + 7 = 77 kg = 770 N.
Ignoring the arm mass, the torque at the bearings will be;
( 700 mm / 70 mm ) * 70 kg = 700 kg force = 7 kN.
That force will be positive on one bearing, negative on the other.

Would I be safe to say this is correct?

I do not understand that last diagram with angles, 35 kg and 105 kg.

 

[BvU]

Was that copied and pasted from another post or was it specific to this post

Specific !

but I don't understand a single piece of it

I'm trying to understand the basics first

I am sorry. Let me try to make it simpler. But it's difficult to know where to start.

Would I be safe to say this is correct?

No.
Left picture: The Earth is pulling down the 70 kg with a force of $F= Mg$. The load M will not fall down if the beam exerts a force that is the same magnitude, but in the opposite direction. If we take $g = -10$ m/s² (negative to indicate it goes in the negative y direction) that means the beam exerts a force of 700 N upwards. And the force exerted by the mass M ON the beam is 700 N downwards. The drawing in post #2 concerns forces on the beam.

Right picture: There is no relationship with the diagram in your post #1, so it's hard to comment. E.g. I don't see a 45 degree angle.

Clear so far ?

Here's a simplified example

To me it looks exactly like the situation in post #1 and 2

If we take a step back and ignore the mass of the beam itself, we get the following:

Imagine the red circle (I adapted to your color scheme ) as a hinge and concentrate on the torque that acts on that hinge (in particular the forces that cause the torque):

We have the 700 N with an arm of 0.7 m that tries to turn clockwise, and the only force that can counteract that is the horizontal component of the top bearing. The lever arm for that one is 0.07 m and we have a torque balance $$F_{1x} \times L_3 + Mg \times L_1 = 0\ \ .$$ Put in the numbers and the result is $F_{1x} = 7$ kN.

At this point we have already a fairly good estimate of the force on the bearings.
Before going into refinements: Is this understandable ?

$\$

 

[DC81]

Welcome to PF.
Is the axis through the two hinges vertical or horizontal?

If vertical, the bearings will have an axial force equal to 70 + 7 = 77 kg = 770 N.
Ignoring the arm mass, the torque at the bearings will be;
( 700 mm / 70 mm ) * 70 kg = 700 kg force = 7 kN.
That force will be positive on one bearing, negative on the other.


I do not understand that last diagram with angles, 35 kg and 105 kg.

700 kgf is what I estimated it to be but I worked it out differently. Thank you for confirming this and in a clear manner!

The last diagram was me trying to understand from a different perspective on how a vertical force affects sideward force at different angles. If you hang a 70kg weight from rope, grab the rope halfway between the hang point and the weight, now push it until the top half is at 45 degrees. Would I be safe to say that the sideward force applied (in my simpleman way of thinking) would be half of the 70kg weight = 35kg?

This aside, thanks for confirming and taking the time to explain your method

 

[DC81]

Specific !



I am sorry. Let me try to make it simpler. But it's difficult to know where to start.



View attachment 302513

No.
Left picture: The Earth is pulling down the 70 kg with a force of $F= Mg$. The load M will not fall down if the beam exerts a force that is the same magnitude, but in the opposite direction. If we take $g = -10$ m/s² (negative to indicate it goes in the negative y direction) that means the beam exerts a force of 700 N upwards. And the force exerted by the mass M ON the beam is 700 N downwards. The drawing in post #2 concerns forces on the beam.

Right picture: There is no relationship with the diagram in your post #1, so it's hard to comment. E.g. I don't see a 45 degree angle.

Clear so far ?


To me it looks exactly like the situation in post #1 and 2

If we take a step back and ignore the mass of the beam itself, we get the following:

View attachment 302515

Imagine the red circle (I adapted to your color scheme ) as a hinge and concentrate on the torque that acts on that hinge (in particular the forces that cause the torque):


View attachment 302516

We have the 700 N with an arm of 0.7 m that tries to turn clockwise, and the only force that can counteract that is the horizontal component of the top bearing. The lever arm for that one is 0.07 m and we have a torque balance $$F_{1x} \times L_3 + Mg \times L_1 = 0\ \ .$$ Put in the numbers and the result is $F_{1x} = 7$ kN.

At this point we have already a fairly good estimate of the force on the bearings.
Before going into refinements: Is this understandable ?

$\$

Thank you for explaining this in simpleman terms, I understand now. Thanks heaps folks, I appreciate all your efforts to get through my thick head haha! Now I can aim for bearings that are rated at or above 700kgf and know that they won't collapse.

 

[Baluncore]

The last diagram was me trying to understand from a different perspective on how a vertical force affects sideward force at different angles.

If the beam is not horizontal, then the effective torque arm will be less than the beam length. The torque at the hinge points will be proportional to the horizontal distance between two vertical lines, the hinge axis, and the axis of the rope that supports (and projects through) the centre of mass. The path of the beam is not important.

 

[Lnewqban]

Welcome!
How do you plan on anchoring the bearings housing onto the vertical surface, and what type of surface it is?
What the material and wall thickness of the arm will be?

 

[BvU]

The last diagram was me trying to understand from a different perspective on how a vertical force affects sideward force at different angles. If you hang a 70kg weight from rope, grab the rope halfway between the hang point and the weight, now push it until the top half is at 45 degrees. Would I be safe to say that the sideward force applied (in my simpleman way of thinking) would be half of the 70kg weight = 35kg?

Unfortunately that is too simple. Forces are vectors quantities and that means they add different from numbers. Google 'vector addition' to find the gory details. As a simple example:

The load of 70 kg hangs from a rope, exerts a downward force of 700 N. You push a point on the rope to the right. If you want the rope to be at 45 degrees, you need to push with 700 N. That way the forces ON the rope add to the light blue force towards the lower right.
Magnitude (Pythagoras) :

Magnitude of F1 and F2 follows from $F2=F_y^2+F_x^2$

comes out as $700 * \sqrt 2 \approx 990$ Newton.

$\$

 

[BvU]

Now I can aim for bearings that are rated at or above 700kgf and know that they won't collapse.

Fair. The mass of the beam itself adds 5%

$$F_{1x}\times L_3 + mg\times L_1+Mg\times L_2 = 0$$

And Pythagoras to include the vertical forces another 2%. $g$ is not 10 but 9.8 m/s², so that is 2% the other way.

The 7 kN is hefty, so the shaft through the bearings needs to be well anchored (as @Baluncore already indicates).

And the one thing not to do is drive with the beam swung out: any bump can increase g considerably. Plus the possible damage with the thing swinging out in a turn or when braking is huge

$\$

 

[DC81]

Welcome!
How do you plan on anchoring the bearings housing onto the vertical surface, and what type of surface it is?
What the material and wall thickness of the arm will be?

Hi Lnewqban.

The arm well be 70x50x5mm RHS 700mm long. It will be MIG welded to a vertical 50mm diameter high carbon steel tube with 30mm bore, machined to house (top & bottom) NSK tapered roller bearings (30202) with static load rating of 13,200N, 35mmOD and 15mm bore, 11.75mm depth, and a machined 15mm 424 stainless steel pin to lock it in place. The pin will lock the whole lot onto a custom bullbar made from 100x75x6mm RHS foundation

 

[Lnewqban]

Hi Lnewqban.

The arm well be 70x50x5mm RHS 700mm long. It will be MIG welded to a vertical 50mm diameter high carbon steel tube with 30mm bore, machined to house (top & bottom) NSK tapered roller bearings (30202) with static load rating of 13,200N, 35mmOD and 15mm bore, 11.75mm depth, and a machined 15mm 424 stainless steel pin to lock it in place. The pin will lock the whole lot onto a custom bullbar made from 100x75x6mm RHS foundation

Never mind, it is structurally over-killed.
Protect those tappered bearings from water and mud!

 

[DC81]

Welcome!
How do you plan on anchoring the bearings housing onto the vertical surface, and what type of surface it is?
What the material and wall thickness of the arm will be?

Hi Lnewqban.

The arm well be 70x50x5mm RHS 700mm long. It will be MIG welded to a vertical 50mm diameter high carbon steel tube with 30mm bore, machined to house (top & bottom) NSK tapered roller bearings (30202) with static load rating of 13,200N, 35mmOD and 15mm bore, 11.75mm depth, and a machined 15mm 424 stainless steel pin to lock it in place. The pin will lock the whole lot onto a custom bullbar made from 100x75x6mm RHS

 

[DC81]

Never mind, it is structurally over-killed.
Protect those tappered bearings from water and mud!

Overkilled is a compliment! I wasn't sure what ballpark I was looking at for load ratings on the bearings. The materials themselves I wouldn't compromise at all, better over-engineered than on or under.

 

[Lnewqban]

Overkilled is a compliment! I wasn't sure what ballpark I was looking at for load ratings on the bearings. The materials themselves I wouldn't compromise at all, better over-engineered than on or under.

For future reference: whatever you lose in arm length, you gain in force, just like for a simple lever.

The bearing to bearing arm is about 50 mm.
Then, 700 mm / 50 mm = 14 times more force than the hanging weight.
75 kgf x 14 = 1050 kgf horizontal force on each bearing and each weld to the bullbar.

As you increase the vertical distance between the bearings, the forces decrease in the same proportion.
Same if the load moves away from the bearings.
Good luck with the project!

 

[DC81]

Thank you!

 

[Baluncore]

It will be MIG welded to a vertical 50mm diameter high carbon steel tube with 30mm bore, machined to house (top & bottom) NSK tapered roller bearings (30202) with ...

I would be concerned with the cost, complexity and weight of your rolling bearing solution. Your requirement is for never more than a 180° rotation, the majority of operation will be static, in a high vibration environment. Failure will be due to brinelling or rusting, unless seals are installed, above and below, to retain lubrication.
https://en.wikipedia.org/wiki/Brinelling
I would instead use a plastic sleeve bearing, made simply from a tight-fitting short piece of black poly pipe. That is cheap and will not rust or rattle.

 

[DC81]

Since my last reply, I'm now actually leaning towards tintered bronze or leaded bronze bushes instead of bearings and reducing the overall material mass. This way it will be cheaper, easier to replace the bushes once every few years, and I won't need to have a complete sealed bearing setup. I appreciate your advice thought mate, thanks a bundle!

 

[sophiecentaur]

Since my last reply, I'm now actually leaning towards tintered bronze or leaded bronze bushes instead of bearings and reducing the overall material mass.

Yes, I would think plane bearings would be simplest. Strength is not a big problem - it would only need to be a cantilever to support a light person, half way out; that puts it in perspective. In my mind, I have a car front suspension 20mm 'king pin' arrangement; that would handle a large vehicle and bumpy ground and hard cornering. Tapered rollers might be needed for fast rotation but these only need 90degree of slow turn.

I would instead use a plastic sleeve bearing, made simply from a tight-fitting short piece of black poly pipe.

What sort if diameter would you suggest? Sag could be a problem with plastic but a bit of slop may not be too bad a thing as the 'other end' could consist of a simple peg, dropping into a slot - similar to a field gate which you just lift and drop into place. (Plus locking pin)
If the system spends most of its time clamped up then wear is hardly an issue. IMO the worst damage would be if that swinging arm got loose and ended up jackknifed or hitting a passing car. P
Some crude (leather?) restraining strap and a brace (nothing 'springy') could avoid that problem. I'm thinking a dark rainy night when you're in a hurry. It will need to be operated by anyone who lifts the tailgate so it has to be simple.

 

[Baluncore]

What sort if diameter would you suggest?

Over time, I have greatly reduced my use of machined bronze sleeves, and increased my acceptance of plastics.

I would guess the outer tube (welded to the swing arm), would have ID = 1½”, say 40 mm. The OD of the inner pintle would then be about 1.25”, like a 1” water pipe. The gap between the two would contain the plastic sleeve, made from a short length of black poly pipe, cut once length-ways to provide a 1 mm gap when installed. That gap will allow for the temperature variation in plastic circumference, which makes for easy assembly.

The advantage of plastic is minimal machining, and low noise or rattle. A metal sleeve in a metal housing is hard to fit, will need lubrication, then will always begin to rattle. A compliant plastic sleeve can be assembled with a hammer, and will absorb sound.

I once hired a Toyota “Troopy” for a seismic exploration. The pin that locked the spare tyre arm in place was missing, so it could swing back and forth into the oncoming traffic. Until it was belayed, the camber of the road kept it in place most of the time. That frame lock should be designed to be fail-safe, without parts that can be lost.

 

[Tom.G]

A search for 'gate latch ideas' on Google Image looks promising.
https://www.google.com/search?tbm=isch&hl=en&source=hp&biw=&bih=&q=gate+latch+ideas


Cheers,
Tom

 

[Baluncore]

Since the end of a vehicle can sometimes accelerate downwards at better than 2G, a gate latch would need to be spring-loaded to about 5G.

 

[sophiecentaur]

and low noise or rattle.

That's an excellent justification.

Since the end of a vehicle can sometimes accelerate downwards at better than 2G, a gate latch would need to be spring-loaded to about 5G.

I wasn't actually suggesting that a gate latch would be for driving - just a hook to park the arm on whilst locking. If the vehicle is parked uphill, you wouldn't want to have to push the arm into place whilst locking. An actual latch would be sure to rattle.