- #1
Chantry09
- 61
- 0
Hi,
I have a question about one of the engines I am looking at for a project. I am linking two cylinders together. One is a cylinder with a pivoting shaft (piston A), the other piston has a cylinder with a fixed axle so it can only move in and out (piston B). To allow piston be to move with the cam, it will have to be attached to a pivot.
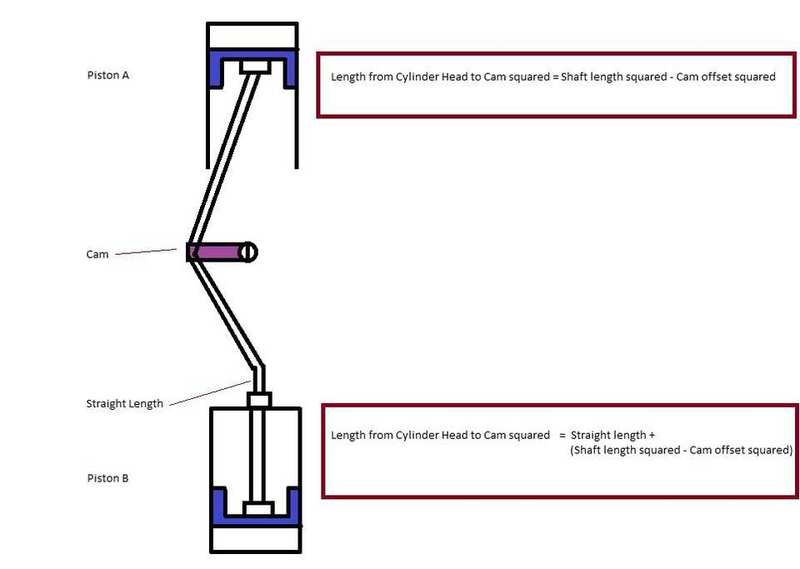
However, what I am concerned with is, these cylinders have to be "in phase" so they are moving the same speed and are at the same point during the cycle. I am thinking that the difference between the way piston a and piston b are pivoted will mean they will not always be in perfect sync.
To achieve this, do I need to fix Piston A so that it has the same straight length of shaft and the same pivoted shaft length? As shown in the diagram below:
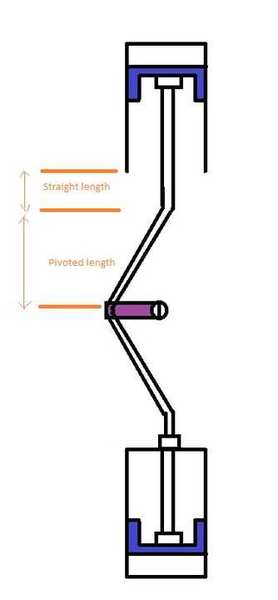
Please let me know if I have explained it well enough. I basically want the relationship between the cylinder head moving and the cam moving to be exactly the same on Piston A and Piston B, and I think the increased pivoting length will alter this relationship.
I have a question about one of the engines I am looking at for a project. I am linking two cylinders together. One is a cylinder with a pivoting shaft (piston A), the other piston has a cylinder with a fixed axle so it can only move in and out (piston B). To allow piston be to move with the cam, it will have to be attached to a pivot.
However, what I am concerned with is, these cylinders have to be "in phase" so they are moving the same speed and are at the same point during the cycle. I am thinking that the difference between the way piston a and piston b are pivoted will mean they will not always be in perfect sync.
To achieve this, do I need to fix Piston A so that it has the same straight length of shaft and the same pivoted shaft length? As shown in the diagram below:
Please let me know if I have explained it well enough. I basically want the relationship between the cylinder head moving and the cam moving to be exactly the same on Piston A and Piston B, and I think the increased pivoting length will alter this relationship.
