- #1
akvadrako
- 282
- 112
I've recently been reading about entanglement between two spatial modes of a single photon. It's a little over my head and there is one aspect about it that I'm particularly unclear on, which I was hoping someone here might be willing to shed some light on it.
The basic setup is described with this picture from the paper (I hope I'm allowed to link this):
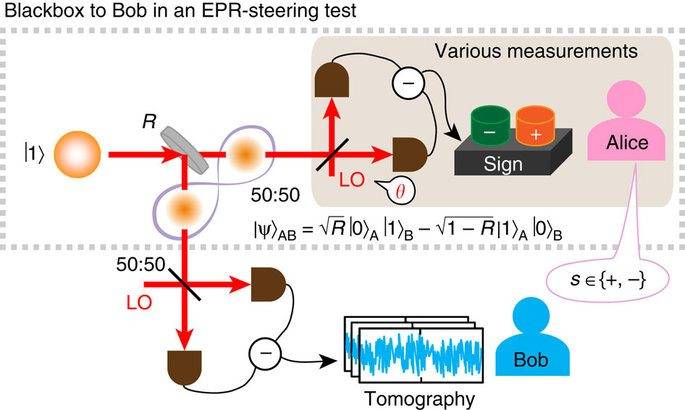
So simplified quite a bit, my understanding is that the procedure goes like this:
If there are not simultaneous measurements by both parties, I don't see how Bob's tomography results can be binned based on the binary result from Alice, unless there is some relationship between subsequent photons.
So what's wrong with my understanding?
The basic setup is described with this picture from the paper (I hope I'm allowed to link this):
So simplified quite a bit, my understanding is that the procedure goes like this:
- A single photon is sent to Bob and Alice with a ##R=50\%## probability distribution, and hence is in the state ##| \psi \rangle = |0\rangle_A|1\rangle_B + |1\rangle_A|0\rangle_B##.
- Next Alice rotates her setup by a chosen angle ##\theta##.
- Then Bob and Alice both measure their photon steams using "full quantum-state tomography using homodyne detection". I'm not very familiar with this but I assume you can't get more than one bit of information for each photon, so it must be an aggregate of many runs.
- Then when their measurements are compared it can be shown that Alice's choice of measurement angle has affected Bob's results.
If there are not simultaneous measurements by both parties, I don't see how Bob's tomography results can be binned based on the binary result from Alice, unless there is some relationship between subsequent photons.
So what's wrong with my understanding?