How did expansion take 380,000 years to let light travel freely?

[syfry]

TL;DR Summary

I know after inflation (from Planck scale to grapefruit size) the universe expanded at a constant rate, cooling as it did so at various stages of density.

Seeking a comparison to understand the density at each stage which had prevented light from traveling far.

Here's as far as I've gotten in the research (please correct any errors I'm making). The stages of high density had included these periods:

• light at high energy was in a cycle of forming into matter, forming back into light, and so on

• until things cooled enough for nucleons to form

• until things cooled enough for electrons to bind

From what I've had explained, during the 380,000 years (maybe near its end?), the light could actually travel perhaps half a light year to a few light years, before it'd get reabsorbed... so there was probably some empty -ish space, but, the universe was still opaque.

If that's the case, what doesn't make sense is how that emptier part wouldn't be cool enough for electrons to bind. Is it because the emitted light would blast away / unbind electrons that did bond?

But the other part that still isn't intuitive, is how did expansion take hundreds of thousands of years for light to travel freely? I'm thinking that enough expansion would create enough space for nucleons and electrons to form atoms, and for light to travel without bumping into stuff.

How dense was the universe at each stage of density? What can we compare each to?

Was the universe like a neutron star of density in the first few minutes?

When it was midway to becoming transparent to light, was the universe density like our sun? (sort of like how light can take thousands of years to travel from the sun's core to the sun's surface)

When the 380,000 years were almost up, was the universe like a thinner version of a nebula, with open spots of space but still enough bunches of matter to reabsorb light?

And how did it take so long for expansion to spread out the matter? Is it because gravity between particles slowly countered the effect?

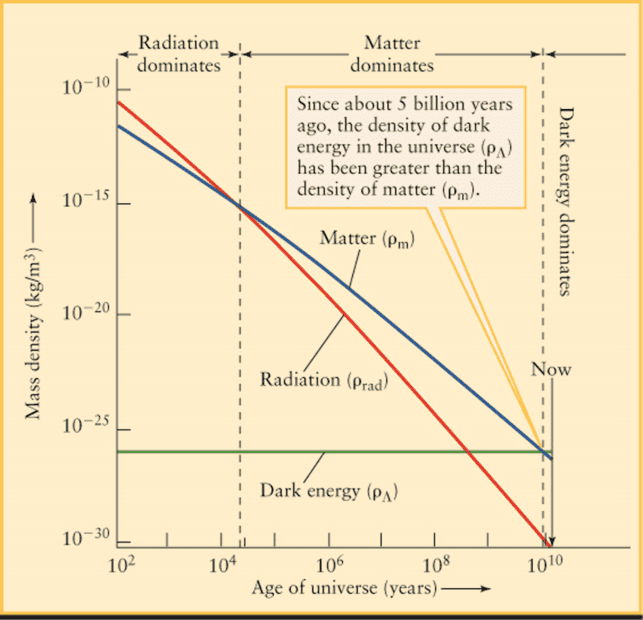
For reference on the rate of expansion, the graph below shows that the rate of expansion has remained exactly steady and consistent:

https://sites.ualberta.ca/~pogosyan/teaching/ASTRO_122/lect30a/6592_fig26_20 [Converted].jpg

 

[hutchphd]

My understanding is that the light energy that takes 8 minutes to travel from the solar surface to my eye takes perhaps 10 million years to reach the surface of the sun from inside where it is generated. So still it is pretty opaque. Not intuitive, I would reckon.

 

[syfry]

My understanding is that the light energy that takes 8 minutes to travel from the solar surface to my eye takes perhaps 10 million years to reach the surface of the sun from inside where it is generated. So still it is pretty opaque. Not intuitive, I would reckon.

I was right there with you on how that isn't intuitive, until I thought about how many atoms are in a grain of sand, then imagined how many grains of sand would fit between the sun's core and its surface, and then knowing a lot more hydrogen would fit in there. So the photon has a very long journey of meandering at random directions, even probably going backward a number of times!

So maybe a hundred thousand years is amazingly quick for light to travel through all of that haha.

Also, I'm at work for a concert so the edit to my post had a long delay since the phone network is busy from all the people in the area.

 

[hutchphd]

Light sort of diffuses out of the sun is my understanding. Somewhat odd but in fact I did some modeling of fluorescence light through skin once which effectively used diffusion ...the Temp was a bit lower of course.........it was pretty useful work actually. ....implantable glucose sensor.

 

[Bystander]

My understanding is that the light energy that takes 8 minutes to travel from the solar surface to my eye takes perhaps 10 million years to reach the surface of the sun from inside where it is generated. So still it is pretty opaque. Not intuitive, I would reckon.

https://www.google.com/search?q=how...650l3.75112j1j7&sourceid=chrome&ie=UTF-8#ip=1

I'm remembering five thousand years for the energy, not specific photons, emission to absorption path lengths O() microns; Gamov, A Star Called the Sun. YMMV

 

[hutchphd]

I said energy quite specifically and particularly......

 

[Ken G]

A good way to think about diffusion is to count the number of "mean free paths" that need to be traversed if the light was going in a straight line, and then multiply the time it would take if it could indeed go in a straight line by that number of mean free paths. That provides an estimate of how long it will actually take. The same would be true for the time to get across a room if you step in random directions, just count the steps walking straight, and how long it takes, and multiply them together to approximate the time if you are walking at random. Since the "straight path" through the Sun is about two seconds, and it actually takes something like fifty thousand years (I've seen different estimates), it means there must be about a trillion mean free paths for the light to get through. Since the radius of the Sun is some 700,000 km, that puts the mean free path, on the average, at a few centimeters.

Getting back to the cosmology, the density in the early universe became much lower than that of the Sun, pretty quickly. However, the mean free path was always much smaller than the distance light could travel in the age of the universe at the time, so it was still "opaque" in that sense. The reason is that free electrons have a very small mass for their charge, so it is easy for light to make an electron shake about. That's how light interacts with electrons, so as long as the electrons were free to move around, the light could not get very far before interacting.

About about 380,000 years old, however, the universe got cool enough (it's not a question of the space there, it's more a question of the temperature, because as you say, the light itself is keeping the atoms ionized until the temperature cools) to start forming neutral hydrogen, locking up those free electrons into the strong electric field of the protons. It is much harder to shake an electron when it is in the strong electric field of a proton! (There is one dramatic exception to this, which is if the light is the right wavelength to shake the electron at a specially chosen frequency that "resonates" with the atom, like a guitar string, and then you get a whopping interaction, but that tends to happen at ultraviolet wavelengths that were not of much importance to the mostly optical and infrared cosmic background radiation at 380,000 years old.) So all those bound electrons became mostly very transparent to light, and the cosmic veil was lifted at that age.

 

[syfry]

that were not of much importance to the mostly optical and infrared cosmic background radiation at 380,000 years old.

Wow, so the entire universe would've been bathed in visible light! (and invisible infrared, or at least invisible to human eyes)

Thanks, your reply helped me to understand how charged particles were the main thing keeping light interacting.

One part doesn't make sense: when light had cooled enough to stop ionizing the individual hydrogen atoms, and the liberated light was visible and infrared, that then implies the steps had skipped over UV light. (since ultraviolet isn't ionizing as far as I know)

 

[PeterDonis]

ultraviolet isn't ionizing

UV is a fairly wide range of energies; the energies at the higher end of the range are ionizing. (Here by "ionizing" we are referring to hydrogen atoms; ionization energies are different for different types of atoms.)

The formation of neutral hydrogen was not a one-time, all-or-nothing event. What actually happened is that, over a short period of time, cosmologically speaking, the relative concentrations of ionized hydrogen and free electrons, vs. neutral hydrogen atoms, went from very high (mostly ionized plasma) to very low (mostly neutral) in a continuous process. The process is continuous because the proportion of photons that have enough energy to ionize hydrogen continuously decreases as the temperature of the universe lowers due to expansion.

 

[Ken G]

Yes, and another fact to bear in mind is that you might think light would lose its ability to ionize hydrogen long before it cooled to 3000 K. After all, the ionization energy of hydrogen is over 13 eV, which means that a 3000 K photon has not even 1/50 the energy needed to ionize H. But a thermal distribution of light at 3000 K still extends past the near UV into the far UV, where ionization does occur. There are very few photons that can do it, but it doesn't take very many, because of the very low densities involved. Ionization competes with recombination, where a free electron is captured by a free proton to make a neutral H atom. At very low density, like the universe at that age, recombination is also very rare, so even though there are few photons capable of ionizing, it still creates an equilibrium where you have a lot of ionized H. To get an equilibrium at mostly neutral H, you must get the radiation temperature all the way down to about 3000 K. (So that's the "jump" you are talking about, you have to get the light so cool that very very few photons can ionize H.)

 

[Drakkith]

Wow, so the entire universe would've been bathed in visible light! (and invisible infrared, or at least invisible to human eyes)

Imagine being immersed in a dense fog and then having someone turn on a bunch of lights all around you. The light is scattered entirely by the fog, so all you'd see is light coming from every direction. The early universe was similar, but much, MUCH brighter. The temperature of recombination is about 3,000 K, which is about 50% higher than the temperature of an incandescent light bulb. It would have been VERY bright even at the last moments before the universe lost its opacity.

Yes, and another fact to bear in mind is that you might think light would lose its ability to ionize hydrogen long before it cooled to 3000 K. After all, the ionization energy of hydrogen is over 13 eV, which means that a 3000 K photon has not even 1/50 the energy needed to ionize H.

I assume that many of the hydrogen atoms that existed during this time were also not in their ground states, making them easier to ionize. Is that correct?

 

[Ken G]

I assume that many of the hydrogen atoms that existed during this time were also not in their ground states, making them easier to ionize. Is that correct?

Your point is well taken that ionization rates have to be considered from all levels, so there can be an upward cascade that involves lower energy photons. But that first step is still such a big one (Lyman alpha) that I don't think the upward cascade would be important. What helps the direction ionization from the ground state is that there are a lot of places for the electron to go if it leaves the atom completely, whereas if it is to go to the second level there are only a few. (In other words, the process has a much bigger target to hit if it involves direct ionization from the ground state.) Thus the rate from the first to second level is very tiny indeed.

 

[Vanadium 50]

It may be clearer to think of the process not as "ionizing hydrogen" but as "depopulating free electrons". Once the electrons are bound, it doesn't matter so much where they are or what they are doing. What matters is what they have stopped doing - scattering light.

 

[Ken G]

It's true that it is really the opposite direction of ionization that matters here, it's called the "era of recombination" because it's going from ionized to bound electrons. But recombination is happening all the time, a typical electron in the early universe will have been in neutral hydrogen many times over prior to that era. Moreover, since recombination depends mostly on density, it was happening faster prior to the era of recombination. The quantity to consider is the recombination rate times the age of the universe at the time, which would have roughly characterized the number of times an electron has been in a neutral atom in its life if it had been a quantity that grows with age. But since it scales roughly like t/a(t)^3, it is actually a quantity that decreases with age, so it doesn't give the number of times the electron has been in an atom. Instead, it shows that electrons were getting captured by protons many more times in the early times than later on, they just didn't stay captured. Hence the issue of depopulating the free electrons turns out to be controlled by how quickly they can (and eventually, cannot) be ionized. One must look to ionization to understand the recombination era.