How did they get that the vorticity = 2##\omega##?

[Hall]

Homework Statement

Deriving an expression for vorticity.

Relevant Equations

Vorticity =$2 \omega$

I'm learning Meteorology, and using the book Atmospheric Science by Wallace and Hobbs. We're discussing the kinematics of the winds (fluids). I shall post some images to say what I don't understand. This is how they define their natural coordinate system

At any point on the surface one can define a pair of axes of a sys- tem of natural coordinates (s, n), where s is arc length directed downstream along the local streamline, and n is distance directed normal to the streamline and toward the left,

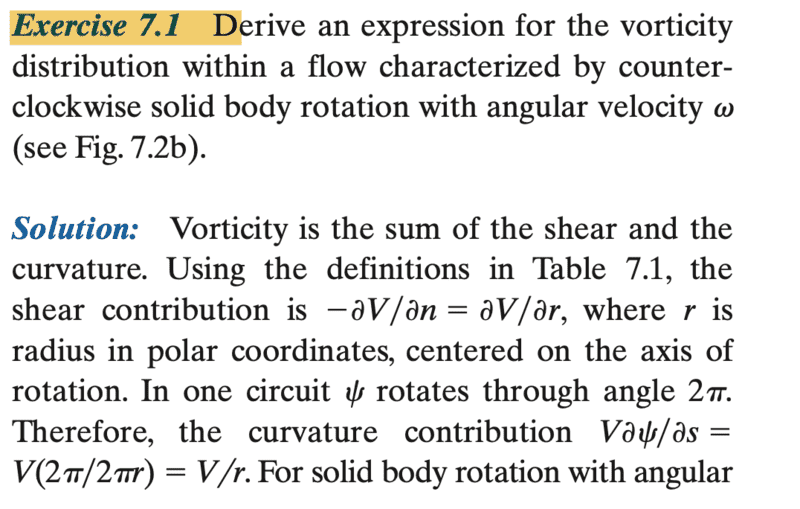
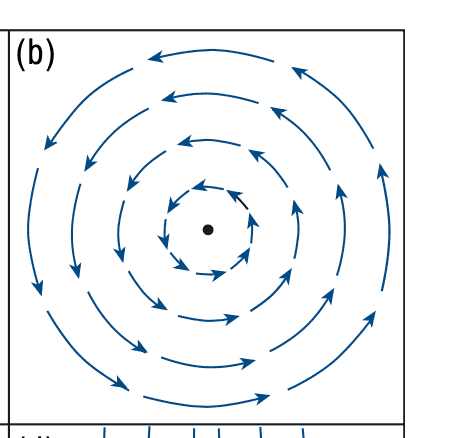I know and understand the concepts of angular velocity, shear, curvature, differentiation, and partial differentiation but for some reason, which is latent to me, I cannot understand anything that the book has done. Will you please explain it to me?

 

[anuttarasammyak]

I am not sure whether I understand the book but mathematical definition of vorticity says
\nabla \times \mathbf{u}=2\mathbf {\omega}
where
u_x=- \omega y,\ u_y=\omega x,\,\ u_z=0