How Do Eddy Current Dampers Work in Space Applications?

[Juanda]

TL;DR Summary

I would like to better understand Eddy Current Dampers.

This post is somewhat of a continuation of this other post. I would like to better understand induction and be able to link the electrical equations with Newton's.
For reference, this is a paper about the Eddy Current Damper I would like to study first.

I think these components are very well suited for space applications because the fluid counterpart might not be as convenient when dealing with vacuum and temperature changes among other potential problems. These ECDs (Eddy Current Damper) don't need wires coming out or anything like that. They are a complete and passive unit that will produce a damping force mainly proportional to the velocity. Frictional forces due to sliding friction are still there because big gear ratios are typically necessary to amplify the effects of the damper and said gear ratios will amplify the friction too. It's not great but it's something that can be dealt with as long as it is taken into account by having proper actuation margins.

First of all, the magnet configuration. The paper mentions the magnet configuration but hides the picture for some reason.

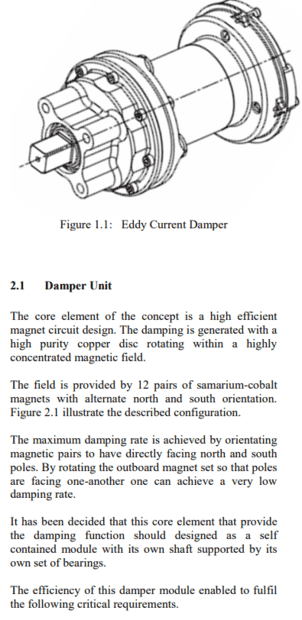
According to what I read in the document, I believe it is a magnetic axial flux configuration with a rotating copper disc as shown in the following picture.

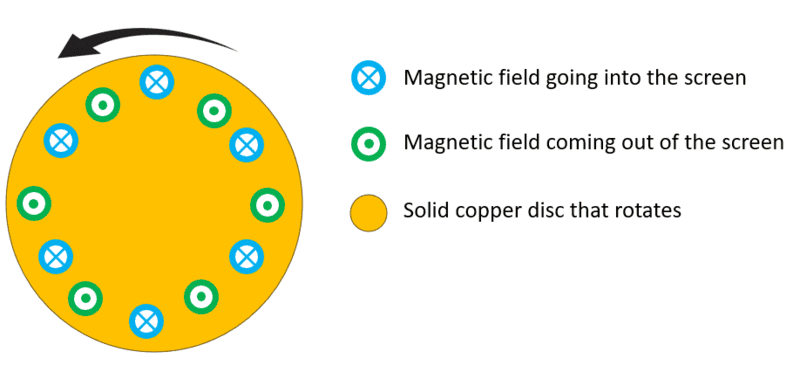
From what I learned in the previous post, the poles must be alternating. Otherwise, it'd be closer to a Faraday Disc which would not produce much damping if the circuit is not closed (even when closed it might not be ideal because of the Faraday Disc Paradox but that's a whole other issue not worth getting into now). With an open circuit (outer radius and shaft are disconnected) and all field lines oriented in the same way, the charges would bunch up at the outer radius and center depending on the magnetic field orientation. On the other hand, an alternating magnet configuration as shown would produce eddy currents that would dissipate the energy because the charges would constantly try to move to the outer radius and then back to the center when they face the opposite magnetic field. Is that reasoning correct?

Secondly, how could the damping coefficient of such a system be calculated? The case for the rod moving through a constant magnetic field (previous post) is clear and the damping would be $c=\frac{B^2l^2}{R}$ where the resistance is dependent on the physical properties of the rod, the cross-section of the rod and the temperature. However, this case is much more complicated. I wouldn't even know how to define the magnetic field. I could work with a simplification of a constant magnetic field in the different 12 sections but still couldn't crack it. The document mentions the damping being proportional to $d^3$ so this problem seems to be solved but I tried some keywords on the net and the equation that describes this element and its derivation didn't come up.
I assume the thicker the disc the greater the cross-section the charges move through so the smaller the resistance. That could have a linear impact. The effect of the diameter I would expect it to be squared because of its influence in the area but it's not the case. Maybe because there is the addition of a linear term due to the transformation from rotational movement to linear movement.
Do you know what's the proper way to derive a conceptual approximation to the damping rate of an ECD like this?

Lastly, this axial flux is the variant I found online. My experience is too limited to judge if it is the best one but I was wondering how it'd compare to a design with radial flux. That way, charges would be circulating towards the bottom and top of the hollow cylinder. I tried to derive the damping of such a configuration as well but I came empty-handed again. I hope that by understanding one of the cases I can work on the other by myself.

 

[Baluncore]

There is a problem with your pdf viewer.

 

[Juanda]

Huh... That's so weird. I use Chrome. I would have not expected compatibility issues with a PDF file. But once downloaded it and opened it with Acrobat I could visualize the document just fine. I thought that picture got redacted for some confidentiality issues.

I added some notes to the drawing.

Do you think that's a correct interpretation of the mechanism? Depending of which section is studied (12 different sections), the magnetic field would be pointing one way or the other.

Do you have any comments about the rest of the points raised in the original post?

 

[Baluncore]

Eddy current brakes were once controlled electrically by changing the current through the electromagnets.
The design with permanent magnets, to control the magnetic braking, rotates one stator magnet plate by one pole against the other. Your diagram shows maximum braking, when all upper and lower poles reinforce.

 

[Juanda]

I'm still struggling to obtain the dampening coefficient of an axial arrangement as shown in previous posts.
There is a somewhat similar problem but simpler in this link.
Example 13.4.213.4.2: A Metal Rod Rotating in a Magnetic Field.

However, in that example, the area actually changes so I can see how to calculate the change in flux $\frac{d\Phi_m}{dt}$ that will result in charges being pushed. When they're pushed through a resistance, power will be dissipated. That dissipation will be linked to the magnetic drag.

In this case, the net change in magnetic flux is 0 because all the incoming flux on one side will be negated on the other. To simplify the previous picture, consider an arrangement with only two constant magnetic fields.

It makes sense that such an arrangement has a net change in flux being zero $\frac{d\Phi_m}{dt}=0$ because it's not a generator. But I know eddy currents will be produced and dissipate energy. That's the whole point of an eddy current damper. I believe the currents will behave as mentioned in the OP.

From what I learned in the previous post, the poles must be alternating. Otherwise, it'd be closer to a Faraday Disc which would not produce much damping if the circuit is not closed (even when closed it might not be ideal because of the Faraday Disc Paradox but that's a whole other issue not worth getting into now). With an open circuit (outer radius and shaft are disconnected) and all field lines oriented in the same way, the charges would bunch up at the outer radius and center depending on the magnetic field orientation. On the other hand, an alternating magnet configuration as shown would produce eddy currents that would dissipate the energy because the charges would constantly try to move to the outer radius and then back to the center when they face the opposite magnetic field. Is that reasoning correct?

Any insights on how to write the equations to obtain the eddy currents and damping coefficient of the system?