How do I accurately calculate optical cycles in a femtosecond laser pulse?

[rinalai]

Homework Statement

A laser with the following specifications of
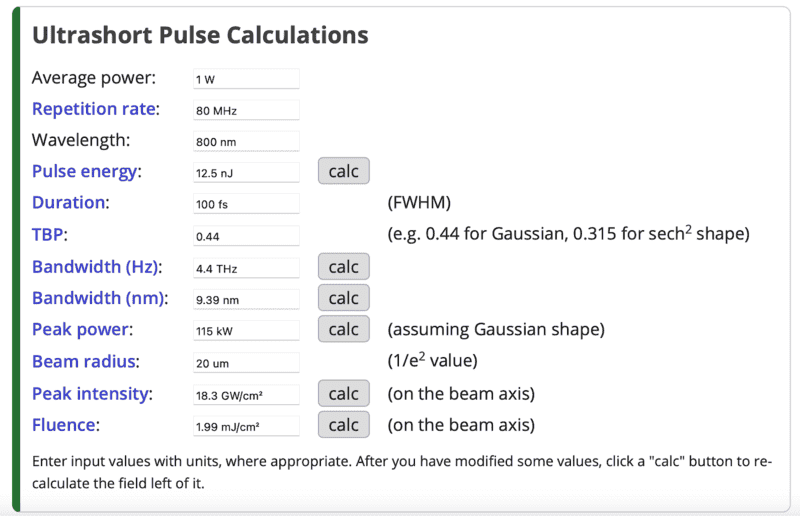
mean power: 1 W
pulse duration: 100 fs
repetition rate: 80 MHz
center wavelength: 800 nm

How many optical cycles are in one pulse?

Relevant Equations

(wavelength 800nm)÷(bandwidth 9.39nm)≈85

I utilized an Ultrashort Pulse Calculator on a website (https://www.rp-photonics.com/ultrashort_pulses.html) and got the bandwidth of 9.39nm with the specifications listed above.
Then divided the wavelength by the bandwidth, and it came out 85(optical cycles).

However, I am not sure if it is the way to calculate the optical cycles in a laser pulse.
I only had an idea that ultrafast lasers, such as femtosecond laser, generate few-cycle pulses.

Would someone here be so kind as to offer some guidance on this question? Thank you!

 

[Steve4Physics]

You need to apply the basic physics of waves, In particular you need to be familiar with the formulae $T=\frac1f$and $v = fλ$ (or $c=fλ$). Have you met these? Assuming you have...

An ‘optical cycle’ is one full oscillation of the wave in the question.
The time taken for one optical cycle is the period, T, of the wave.
The number of optical cycles per second is the frequency, f, of the wave.

Here are some questions for you to work through. Post your working and answers.

The wavelength of an electromagnetic wave is 800nm (in a vacuum or, near enough, in air).
Q1. For interest, in what part of the EM spectrum is the wave?
Q2. Calculate the wave’s frequency in Hz.
Q3. Calculate the wave’s period (i.e. the time for 1 optical cycle) in seconds.
Q4. What is the wave’s period (i.e. the time for 1 optical cycle) in femtoseconds?
Q5. If each pulse last 100fs, how many optical cycles are there in each pulse?[Edit - minor changes.]

 

[rinalai]

Q1. For interest, in what part of the EM spectrum is the wave?
A. electrical part
Q2. Calculate the wave’s frequency in Hz.
A. frequency f = repetition rate: 80 MHz= 8x10^7 Hz
Q3. Calculate the wave’s period (i.e. the time for 1 optical cycle) in seconds.
A. period T=1/f = 1/8x10^7 = 1.25x10^-8 sec
Q4. What is the wave’s period (i.e. the time for 1 optical cycle) in femtoseconds?
A. T= 1.25x10^-8 sec = 1.25x10^-8 x10^15 = 1.25x10^7 fs
Q5. If each pulse last 100fs, how many optical cycles are there in each pulse?
A. Since a pulse 100 fs is shorter than a wave’s period(1.25x10^7 fs), I stucked here...
Is there anything wrong during the process?

 

[jbriggs444]

Q1. For interest, in what part of the EM spectrum is the wave?
A. electrical part

The electromagnetic spectrum does not have an "electrical" part. Nor a "magnetic" part.

It does have sections that we call "infrared", "visible", "ultraviolet", etc.

Q2. Calculate the wave’s frequency in Hz.
A. frequency f = repetition rate: 80 MHz= 8x10^7 Hz

That is not the frequency of the wave. That is the rate at which pulses are generated.

Q3. Calculate the wave’s period (i.e. the time for 1 optical cycle) in seconds.
A. period T=1/f = 1/8x10^7 = 1.25x10^-8 sec

Again, the question asks about the wave during the pulses, not about the pulses.

Q4. What is the wave’s period (i.e. the time for 1 optical cycle) in femtoseconds?
A. T= 1.25x10^-8 sec = 1.25x10^-8 x10^15 = 1.25x10^7 fs

Again, the question asks about the wave, not the pulses.

Q5. If each pulse last 100fs, how many optical cycles are there in each pulse?
A. Since a pulse 100 fs is shorter than a wave’s period(1.25x10^7 fs), I stucked here...

See if you can do better once you correct the answers the previous questions.

 

[SammyS]

You have answered @Steve4Physics questions incorrectly - perhaps because you did not understand the questions.
They all refer to electromagnetic waves having wavelength of 800nm.

Q1. For interest, in what part of the EM spectrum is the wave?
A. electrical part
...

I see that @jbriggs444 beat me to making a reply, so I'll cut this off here.

 

[Tom.G]

The problem is asking how many cycles of the LASER light are made while the pulse is ON.

Take a look at this image.
The top trace would be the 80MHz repitition rate.
The bottom trace would be the optical laser pulse with wavelength of 800nm.

(image from: https://rfmw.em.keysight.com/spdhelpfiles/33500/webhelp/US/Content/__F_CH5_Tutorial/Waveform Generation Tutorial.htm#Modulation)

Cheers,
Tom

 

[rinalai]

Thanks, Steve4Physics,Tom.G, jbrggs, SammyS, for your comments.
I tried again:
The wavelength of an electromagnetic wave is 800nm
Q1. For interest, in what part of the EM spectrum is the wave?
A. infrared
Q2. Calculate the wave’s frequency in Hz.
A. f=c/λ = 3x10^8 m/s ÷ (800nm = 8x10^-7 m) = 3.75x10^14 Hz
Q3. Calculate the wave’s period (i.e. the time for 1 optical cycle) in seconds.
A. T=1/f =1/ 3.75x10^14=2.6x10^-15 sec
Q4. What is the wave’s period (i.e. the time for 1 optical cycle) in femtoseconds?
A. 2.6x10^-15 sec=2.6fs
Q5. If each pulse last 100fs, how many optical cycles are there in each pulse?
A. 100fs/2.6fs=38.46 optical cycles

 

[rinalai]

There are a few more questions on the same laser:

mean power: 1 W​

pulse duration: 100 fs​

repetition rate: 80 MHz​

center wavelength: 800 nm​

What is the (1)spectral width (2)peak power of a pulse ?

For spectral width, I tried calculation as 100fs x 0.3µm/fs = 100 µm
but it seemed incorrect.

I assumed that energy per pulse= 1W(J/s) ÷ 80MHz= 1.25x10-8 J= 12.5nJ
but what will peak energy(=energy per pulse width) be?

 

[Steve4Physics]

Thanks, Steve4Physics,Tom.G, jbrggs, SammyS, for your comments.
I tried again:
The wavelength of an electromagnetic wave is 800nm
Q1. For interest, in what part of the EM spectrum is the wave?
A. infrared
Q2. Calculate the wave’s frequency in Hz.
A. f=c/λ = 3x10^8 m/s ÷ (800nm = 8x10^-7 m) = 3.75x10^14 Hz
Q3. Calculate the wave’s period (i.e. the time for 1 optical cycle) in seconds.
A. T=1/f =1/ 3.75x10^14=2.6x10^-15 sec
Q4. What is the wave’s period (i.e. the time for 1 optical cycle) in femtoseconds?
A. 2.6x10^-15 sec=2.6fs
Q5. If each pulse last 100fs, how many optical cycles are there in each pulse?
A. 100fs/2.6fs=38.46 optical cycles

Well done. But watch out for rounding errors. For example the period of the wave is T=2.7×10⁻¹⁵s (rounded to 2 significant figures). Not 2.6x10⁻¹⁵s.

Unrounded values should be used in calculations, Final answers should be rounded appropriately,

That means the number of cycles per pulse is 100/2.66666667 which is not 38.46.

 

[Steve4Physics]

What is the (1)spectral width (2)peak power of a pulse ?

For spectral width, I tried calculation as 100fs x 0.3µm/fs = 100 µm
but it seemed incorrect.

Where does 0.3µm/fs come from?

100 x 0.3 is not 100!

Have you been taught the relationship between pulse width (τ) and frequency-bandwidth (Δf) for a rectangular pulse? I think that's what you are expected to use.

I assumed that energy per pulse= 1W(J/s) ÷ 80MHz= 1.25x10-8 J= 12.5nJ
but what will peak energy(=energy per pulse width) be?

I guess the pulse is rectangular. That means the peak energy [edit - I mean power] during a pulse is the same thing as the average energy [edit - I mean power] during the pulse. So I think the question is badly worded and should really say: "What is the average power during a single pulse".

(For a non-rectangular pulse, you could find the instantaneous maximum power but to do this you would need information on the pulse-shape.)

 

[rinalai]

Well done. But watch out for rounding errors. For example the period of the wave is T=2.7×10⁻¹⁵s (rounded to 2 significant figures). Not 2.6x10⁻¹⁵s.

Unrounded values should be used in calculations, Final answers should be rounded appropriately,

That means the number of cycles per pulse is 100/2.66666667 which is not 38.46.

Thank you for pointing out the rounding error.
Recalculated cycles of pulse is 37.

 

[rinalai]

Where does 0.3µm/fs come from?

100 x 0.3 is not 100!

Have you been taught the relationship between pulse width (τ) and frequency-bandwidth (Δf) for a rectangular pulse? I think that's what you are expected to use.I guess the pulse is rectangular. That means the peak energy during a pulse is the same thing as the average energy during the pulse. So I think the question is badly worded and should really say: "What is the average power during a single pulse".

(For a non-rectangular pulse, you could find the instantaneous maximum power but to do this you would need information on the pulse-shape.)

 

[Steve4Physics]

Thank you for pointing out the rounding error.
Recalculated cycles of pulse is 37.

I get 37.5. Rounding-rules say 37.5 should be rounded to 38. But if you want the number of complete pulses then I agree with 37.

 

[rinalai]

0.3µm/fs is the speed of light c=3x10^8 m/s= 0.3µm/fs
100fs x 0.3µm/fs = 30 µm (sorry that I mistyped)
Is this the spectral width of the pulse?

Besides, I think the pulse-shape was a Gaussian pulse.
Should I go for FWHM in this case?

 

[jbriggs444]

0.3µm/fs is the speed of light c=3x10^8 m/s= 0.3µm/fs
100fs x 0.3µm/fs = 30 µm (sorry that I mistyped)
Is this the spectral width of the pulse?

Besides, I think the pulse-shape was a Gaussian pulse.
Should I go for FWHM in this case?

The "spectral width" of the pulse is how uncertain we are about a measurement of the frequency of the signal during a pulse. It tells us how smeared out our measurements would look when plotted against the electromagnetic spectrum.

It is not measured in units of distance (micro-meters). It is measured in units of frequency (Hertz).

I do not know if the chapter you are working on goes into Fourier analysis, so let us ignore that.

One way of thinking about the measurement is that you are counting wave peaks. You have this wave form that is chopped off at the ends. You count peaks and you get 37. Or maybe you get 38. That is how fuzzy your measurement is. So what is the frequency associated with 37 peaks per pulse? And what is the frequency associated with 38 peaks per pulse? That is an approximation for the frequency range of your measurements.

Another, somewhat more accurate way of thinking about it is that your measurement process will involve something like a resonator. If you only have 37 cycles of signal to resonate with, a signal that only gets one quarter cycle off over the course of those 37 cycles will still excite your measurement device.

However, I've been out of school a long time and we never covered this material. So I do not know whether you are expected to throw in a divisor of $\pi$ or something similar when you calculate the spectral width of a truncated signal.

 

[Steve4Physics]

0.3µm/fs is the speed of light c=3x10^8 m/s= 0.3µm/fs
100fs x 0.3µm/fs = 30 µm (sorry that I mistyped)
Is this the spectral width of the pulse?

No. You have simply calculated how far the (infrared) light travels in 100fs (distance = speed x time).

Besides, I think the pulse-shape was a Gaussian pulse.
Should I go for FWHM in this case?

Have you posted the full question? The shape of the pulse can make a difference.

In addition to what @jbriggs444 has said, here are a few facts which might help:

For a rectangular pulse of duration τ and carrying energy E:
- peak power is Pmax = E/τ; it is the same as the average power during the pulse;
- optical bandwidth in terms of frequency is Δf = 1/τ.

For a Gaussian pulse with FWHM = τ and carrying energy E:
- peak power is Pmax ≈ 0.94E/τ
- optical bandwidth in terms of frequency is Δf = 0.44/τ.

I got some of the information from memory and some from https://www.rp-photonics.com/gaussian_pulses.html so can’t guarantee accuracy. You should check your notes/text-book to make sure you are given these (or equivalent) formulae.

[Edit - spelin korekted.]

 

[rinalai]

Here is the full question:

The circled ones are those I am still trying to figure out

 

[Steve4Physics]

Here is the full question:
View attachment 292290
The circled ones are those I am still trying to figure out

For question-a, what formula are you given (in notes or textbook) for spectral width?

Note, 'spectral width' and optical bandwidth are basicaly the same. The former would usually be expressed as the spread of wavelength and the latter would usually be expressed as the spread of frequency. It is not clear which (wavelength or frequency) you are being asked to find – you will need to refer to your notes.
______________

For quesion-g, there is a mistake in the question. It asks for peak power (of a Gaussian pulse). But then says ‘energy per pulse width’ which means average power.

You will have to decide which (peak or average) is actually required. If the work is to be handed in, give both answers with an explanation. See my formulae in Post#16.
______________

For question-i you need the answer from question-g. If the radius of the lens is R, what is its area? Write an epxression for the sunlight power collected by the lens and equate it to your answer to question-i. Then solve for R.

 

[PhDeezNutz]

Is it appropriate to provide help on an assignment designated as a “quiz”?

If it was designated as HW I understand why help would be allowed.

 

[rinalai]

For question-a, what formula are you given (in notes or textbook) for spectral width?

Note, 'spectral width' and optical bandwidth are basicaly the same. The former would usually be expressed as the spread of wavelength and the latter would usually be expressed as the spread of frequency. It is not clear which (wavelength or frequency) you are being asked to find – you will need to refer to your notes.
______________

For quesion-g, there is a mistake in the question. It asks for peak power (of a Gaussian pulse). But then says ‘energy per pulse width’ which means average power.

You will have to decide which (peak or average) is actually required. If the work is to be handed in, give both answers with an explanation. See my formulae in Post#16.
______________

For question-i you need the answer from question-g. If the radius of the lens is R, what is its area? Write an epxression for the sunlight power collected by the lens and equate it to your answer to question-i. Then solve for R.

Thanks, Steve4Physics, for your kind comments.

Here is my answers and the professor's feedback(in italic):

1.6x10^14(Hz) corresponds to 9.4 nm

g. Peak power is pulse energy per pulse duration: 12.5 nJ/100 fs = 125 kW

h. Focus is the same as in h. So we want 125 kW from the sun.
Since we get 1 kW/m^2, we need an area of 125 m^2

 

[Steve4Physics]

Hi @rinalai.

Here is my answers and the professor's feedback(in italic):
View attachment 292553
1.6x10^14(Hz) corresponds to 9.4 nm

I believe your answer is wrong.

If you have written the professor’s feedback correctly, your professor hasn’t spotted your (serious) mistake and has incorrectly written:
“1.6x10^14(Hz) corresponds to 9.4 nm “
which itself is wrong (though 9.4nm is the correct answer).

Optical bandwidth ($Δν$) is the spread of frequencies required to make a pulse. For a Gaussian pulse:
$Δν = \frac {0.441}{Δτ}$ where $τ$ is the pulse-width (100fs); $τ$ is NOT the period (T) of the wave. You mustn’t confuse the two. Please look at the diagram in Tom.G’s Post’6. Make sure you know the difference between a pulse and a wave-cycle.

$Δν= \frac {0.441}{100*10⁻¹⁵} = 4.41 \times 10^{12}$ Hz

Spectral width ($Δλ$) is the spread of wavelengths. It can be calculated from $Δν$ using a formula you should have:

$Δλ = \frac {λ²Δν}{c}$

You should calculate $Δλ$ for yourself to see that it giveS the same answer as your professor’s (9.4nm).

g. Peak power is pulse energy per pulse duration: 12.5 nJ/100 fs = 125 kW

I would call this “ average power during a pulse”, not “peak power” – as already noted in Post #18.

h. Focus is the same as in h. So we want 125 kW from the sun.
Since we get 1 kW/m^2, we need an area of 125 m^2

I hope you understand this. I would have taken it slightly further and also found the radius of the lens.

 

[rinalai]

Hi @rinalai.

I believe your answer is wrong.

If you have written the professor’s feedback correctly, your professor hasn’t spotted your (serious) mistake and has incorrectly written:
“1.6x10^14(Hz) corresponds to 9.4 nm “
which itself is wrong (though 9.4nm is the correct answer).

Optical bandwidth ($Δν$) is the spread of frequencies required to make a pulse. For a Gaussian pulse:
$Δν = \frac {0.441}{Δτ}$ where $τ$ is the pulse-width (100fs); $τ$ is NOT the period (T) of the wave. You mustn’t confuse the two. Please look at the diagram in Tom.G’s Post’6. Make sure you know the difference between a pulse and a wave-cycle.

$Δν= \frac {0.441}{100*10⁻¹⁵} = 4.41 \times 10^{12}$ Hz

Spectral width ($Δλ$) is the spread of wavelengths. It can be calculated from $Δν$ using a formula you should have:

$Δλ = \frac {λ²Δν}{c}$

You should calculate $Δλ$ for yourself to see that it giveS the same answer as your professor’s (9.4nm).

Thanks for pointing out the mistake.
Yes, by using the formula

Spectral width= (800x10^-9 m)^2 x (4.41x10^12 sec)/3x10^8(m/s) = 9.4nm
could finally be derived.

Thank you so much for patiently guiding through this question.
It had been a difficult one for me

 

[Steve4Physics]

Thanks for pointing out the mistake.
Yes, by using the formula View attachment 292602
Spectral width= (800x10^-9 m)^2 x (4.41x10^12 sec)/3x10^8(m/s) = 9.4nm
could finally be derived.

Thank you so much for patiently guiding through this question.

Edited

You are welcome. For information, note that you have a couple of minor mistakes (wrong unit, missing brackets) in your equation:
"Spectral width= (800x10^-9 m)^2 x (4.41x10^12 sec)/3x10^8(m/s)"