How do I change this integral limit from x to t?

[PainterGuy]

Homework Statement

How do I change the integral limit from x to t.

Relevant Equations

I've included the equations in main posting. Thanks.

Hi,

It's not a homework problem. I was just doing it and couldn't find a way to change the integral limit from "x" to "t". I should end up with kinetic energy formula, (1/2)mv^2. I've assumed that what I've done is correct. Thank you!

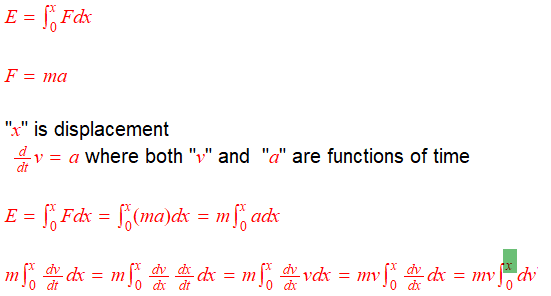
Edit:
"E" is work done.

 

[Delta2]

In the very last step of your derivation, where you change the variable of integration from $dx$ to $dv$ the integral limit has to change but not from $x$ to $t$ but from $x$ to $v_f$ that is the final velocity. Also the lower limit has to change from$0$ to $v_i$ that is the initial velocity.

P.S the two last steps where you pull out $v$ out of the integral, is not a correct thing to do. Velocity $v$ is a function of time and displacement $x$ is also a function of time, so there is an implicit equation between v and x, that is essentially velocity v is a function of distance x that is $v=v(x)$ so it just can't be taken out of the integral.

To see this more clearly, take the case where the force F is constant, hence we have constant acceleration $a$ and the velocity $v(t)=at$ (assuming zero initial velocity). But it is also $$x(t)=\frac{1}{2}at^2\Rightarrow t=\sqrt{\frac{2x}{a}}$$ and thus replacing this t in the first equation we get $$v(x)=a\sqrt{\frac{2x}{a}}=\sqrt{2ax}$$

 

[andrewkirk]

You have two problems.

First you can't write $\int_0^x F\,dx$. It doesn't mean anything, because you are using the same symbol for integration variable and limit.
So instead, use $x'$ as your integration variable: $\int_0^x F\,dx'$.

Secondly, you can't move $v$ outside the integral as you do in the 2nd last step, because it changes with the integration variable $x'$.

The development you want is:

$$
m\int_0^x \frac{dv}{dt}dx'
=m\int_{x'=0}^{x'=x} \frac{dx'}{dt}dv
=m\int_{v=v_0}^{v=v_x} v\,dv
$$
where $v_0,v_x$ are the velocities at locations 0 and $x$.

The integration is then straightforward.