- #1
Lia44
1. Convert 6.7 km/hr/sec to m/sec^2.
I was given the initial value and the units (kilometers/hour/seconds) to (meters/seconds squared).
2. image for clarification
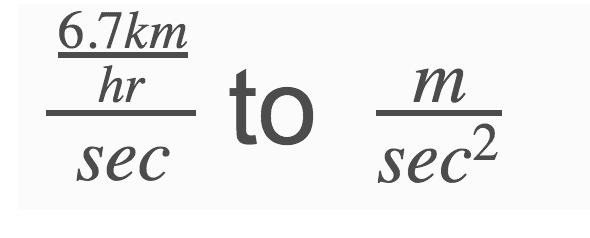
3. The correct answer is 1.89 m/s^2. I watched a video on how to do it (and followed the video to get the following), but I don't understand why I can write the initial value as 6.7km/(hr*s), as shown in the following image.
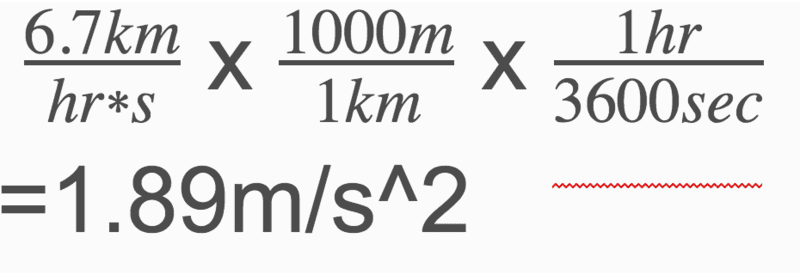
I was given the initial value and the units (kilometers/hour/seconds) to (meters/seconds squared).
2. image for clarification
3. The correct answer is 1.89 m/s^2. I watched a video on how to do it (and followed the video to get the following), but I don't understand why I can write the initial value as 6.7km/(hr*s), as shown in the following image.
