How do I find the circumference and Area of this figure?

[arhzz]

Homework Statement

Find the circumference

Relevant Equations

-

Hello! Consider this figure

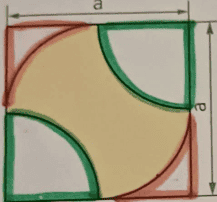
Where a = 5 cm. I need to find the Circumference and Area of this figure.

For the Circumference I tried it like this. I have these 2 shapes, in red and in green

For the green one its basically 2 times a quater of a circle so it should be $$ C_1= 2 * \frac{2\pi r}{4} $$ where r is the radius. Now for the radius looking at the picuture it should be a/2 so 2,5 cm.

Now for the red one I thought it is a square minus the quater of a circle. Since we have that 2 times as well I multiplied it by two

$$ C_2 = (4a - \frac{2\pi r}{4}) * 2 $$

Where a should be a/2 and r should also be r/2 looking from the picture. Now I add $C_1$ and $C_2$ together and I should get the total circumference. Now the solution should be 15,7 cm but I dont get that, and I dont see what I am doing wrong.

Any insight?

 

[Mark44]

Hello! Consider this figure
View attachment 343542
Where a = 5 cm. I need to find the Circumference and Area of this figure.

Circumference and area of which figure? Are you supposed to find the circumference and area of the yellow region in the middle?
For the circumference of the yellow figure, it is bounded by four arcs that are quarters of the circumference of a circle. The circle's diameter is 5, so the circumference would be how much?

For the Circumference I tried it like this. I have these 2 shapes, in red and in green
For the green one its basically 2 times a quater of a circle so it should be $$ C_1= 2 * \frac{2\pi r}{4} $$ where r is the radius. Now for the radius looking at the picuture it should be a/2 so 2,5 cm.

Now for the red one I thought it is a square minus the quater of a circle. Since we have that 2 times as well I multiplied it by two

$$ C_2 = (4a - \frac{2\pi r}{4}) * 2 $$

Where a should be a/2 and r should also be r/2 looking from the picture. Now I add $C_1$ and $C_2$ together and I should get the total circumference. Now the solution should be 15,7 cm but I dont get that, and I dont see what I am doing wrong.

 

[WWGD]

By circumference, you mean the peeimeter?

 

[Lnewqban]

At midpoint of each “a” side, the first portions of the curves must be either tangent or perpendicular to it.
Therefore, it is safe just to flip the green curves about the “a-a” axis, to make any calculation easier.

 

[SammyS]

Circumference and area of which figure? Are you supposed to find the circumference and area of the yellow region in the middle?
For the circumference of the yellow figure, it is bounded by four arcs that are quarters of the circumference of a circle. The circle's diameter is 5, so the circumference would be how much?

I suspect that the problem asks for the circumference and area of the region you found the circumference of.

The area of this region is even easier to determine .

 

[arhzz]

By circumference, you mean the peeimeter?

Yes the perimeter.

 

[arhzz]

Circumference and area of which figure? Are you supposed to find the circumference and area of the yellow region in the middle?
For the circumference of the yellow figure, it is bounded by four arcs that are quarters of the circumference of a circle. The circle's diameter is 5, so the circumference would be how much?

Yea the yellow region, should have maybe stated that explicitly, sorry.

Well if the diameter is 5, than the circumference is just 5 times pi.

 

[Mark44]

Yea the yellow region, should have maybe stated that explicitly, sorry.

Well if the diameter is 5, than the circumference is just 5 times pi.

Yes.

 

[arhzz]

Yes.

Oh and that gives me 15,7 cm. Neat

But could you elaborate more on how you came to that conclusion, I can see that the diameter is 5 but how did you know that the yellow thing is simply the perimieter of the whole circle around it?

 

[Hill]

Oh and that gives me 15,7 cm. Neat

But could you elaborate more on how you came to that conclusion, I can see that the diameter is 5 but how did you know that the yellow thing is simply the perimieter of the whole circle around it?

Does this picture help?

 

[Mark44]

how did you know that the yellow thing is simply the perimieter of the whole circle around it?

My assumption is that all four arcs, the two green ones and the two red ones, are each a quarter of the circle. Flip the two green arcs in the picture you posted (and @Hill reposted), and you get a circle whose diameter is 5.

 

[arhzz]

Okay I see it now thank you. And how would I calculate the area now. The solution says it should be 12,5 cm^2.

I tried finding the area of the red and green parts and subtracting them. But I am having trouble finding the area of the red area.

 

[Hill]

Okay I see it now thank you. And how would I calculate the area now. The solution says it should be 125 cm^2.

I tried finding the area of the red and green parts and subtracting them. But I am having trouble finding the area of the red area.

Look at this picture again:

Every one of the four small squares has the same two parts, $x$ and $y$, some internal for your figure and some external. You have enough data to find the areas of $x$ and $y$, and from them, the area of your figure.
However, before finding the areas of $x$ and $y$ you can see that you don't need them separately in order to find the area of your figure.

 

[Hill]

The solution says it should be 125 cm^2.

It certainly NOT 125 cm^2. The area of the entire square is 25 cm^2.

 

[Mark44]

The area of this region is even easier to determine .

And how would I calculate the area now. The solution says it should be 125 cm^2.

Either you're looking at the solution to a different problem, or the solution given is obviously wrong.

 

[Orodruin]

It certainly NOT 125 cm^2. The area of the entire square is 25 cm^2.

Missing a decimal dot perhaps …

 

[arhzz]

Oh I did not even see that, obviously it is 12,5 cm^2. I will edit my post. But still I cannot get on that value :/

 

[arhzz]

Look at this picture again:
View attachment 343597
Every one of the four small squares has the same two parts, $x$ and $y$, some internal for your figure and some external. You have enough data to find the areas of $x$ and $y$, and from them, the area of your figure.
However, before finding the areas of $x$ and $y$ you can see that you don't need them separately in order to find the area of your figure.

Okay so basically what you are saying if I find the values of the y's lets say (green) I should be able to automatically find the value of x (the red ones). Is it something alon the lines I find the value of the entire thing which is $25 cm^2$ and if I subract the values of the 2 green y's i should find the values of the x's right? Or am I understanding it wrong

 

[SammyS]

Okay so basically what you are saying if I find the values of the y's lets say (green) I should be able to automatically find the value of x (the red ones). Is it something alon the lines I find the value of the entire thing which is $25 cm^2$ and if I subract the values of the 2 green y's i should find the values of the x's right? Or am I understanding it wrong

Try it and see.

 

[Orodruin]

I suggest adding up the areas to see what the total area of the square equals. Then add up the areas that make up the yellow figure. Notice something?

 

[Hill]

Okay so basically what you are saying if I find the values of the y's lets say (green) I should be able to automatically find the value of x (the red ones). Is it something alon the lines I find the value of the entire thing which is $25 cm^2$ and if I subract the values of the 2 green y's i should find the values of the x's right? Or am I understanding it wrong

There are several ways to find the area $S$ of your figure. In any way, you need to do something - not just talk about doing something.

 

[arhzz]

There are several ways to find the area $S$ of your figure. In any way, you need to do something - not just talk about doing something.

Was pressed for time today, I will sit down with a pen and paper and put the words into practice.

 

[arhzz]

Okay so I was able to solve it. This trick that Hill gave me worked for me. When I put a cross over the entire square I was able to find the area of the quater circles and the area of the squares that "appeared" after I put the cross. Than I just substracted the area of the quater circle (y) in the picture from Hill from the square and was able to find x. The same analogy for y, and I got the Area that I was looking for.

Thanks everyone for the help!

 

[Hill]

Okay so I was able to solve it. This trick that Hill gave me worked for me. When I put a cross over the entire square I was able to find the area of the quater circles and the area of the squares that "appeared" after I put the cross. Than I just substracted the area of the quater circle (y) in the picture from Hill from the square and was able to find x. The same analogy for y, and I got the Area that I was looking for.

Thanks everyone for the help!

That's fine, but you could get the answer in simpler ways.

For example, you can just count $x$'s and $y$'s. The yellow shape consists of 2 $x$'s and 2 $y$'s. The entire square consists of 4 $x$'s and 4 $y$'s. Thus, the yellow shape area is half of the entire square area.

Alternatively, you can imagine the yellow $x$ from the bottom-left quarter moved to the white $x$ in the top-left quarter, and the yellow $x$ from the top-right quarter moved to the white $x$ in the bottom-right quarter. Then the yellow shape would occupy two out of four quarters of the square. That is, half again.

 

[Orodruin]

Just to spell that out algebraically:

Total area of square:
$$
A_\square = 4x + 4y = 4(x+y)
$$
Total area of figure:
$$
A = 2x + 2y = 2(x+y)
$$
Hence
$$
\frac{A}{A_\square} = \frac{2(x+y)}{4(x+y)} = \frac 12 \qquad \Longrightarrow \qquad
A = \frac{A_\square}{2}
$$

Edit: Note that you don't even need to compute neither $x$ nor $y$ to conclude this. The result will be the same for any division of the quarter squares where one piece is included in the figure in two of the quarter squares and the other piece is included in the other two.