- #1
Keplini
- 1
- 0
Homework Statement
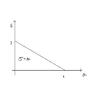
Find the center of mass and inertia tensor at the CoM of the following triangle. Density of the triangle is [tex]\sigma(x,y)[/tex] = x and y=3-3/4x .
Homework Equations
Find the inertia tensor at the origin (x,y,z) and apply the parallel axis theorem
I[tex]_{ij}[/tex]=[tex]\int[/tex]dV([tex]\delta^{ij}[/tex][tex]\vec{x}^{2}-x^{i}x^{j}[/tex])
The Attempt at a Solution
I've been able to find the mass (which gave me 8 -correct me if I'm wrong-), the CoM and now I'm trying to find the inertia tensor. For the first component, I get something like:
I[tex]_{xx}[/tex]=[tex]\int^{4}_{0}[/tex][tex]\int^{3-3/4x}_{0}[/tex] [tex]xy^2 dx dy[/tex]
which gives me something like
[tex]I_{xx}=\int^{4}_{0}x\frac{(3-3/4x^)^{3}}{3}dx[/tex]
which looks like a big monster and I don't feel like integrating that ! ;) Basically, I believe it's getting way too complicated to be the good answer. Any help on finding that inertia tensor would be greatly appreciated !
Thanks,
Kep
Attachments
Last edited: