How do I get the book's answer for finding the area of a gable?

[xyz_1965]

Good morning everyone. I'm working on some right-triangle trigonometry problems in the Cohen textbook as I wait to receive my Sullivan precalculus book. It should arrive next week.

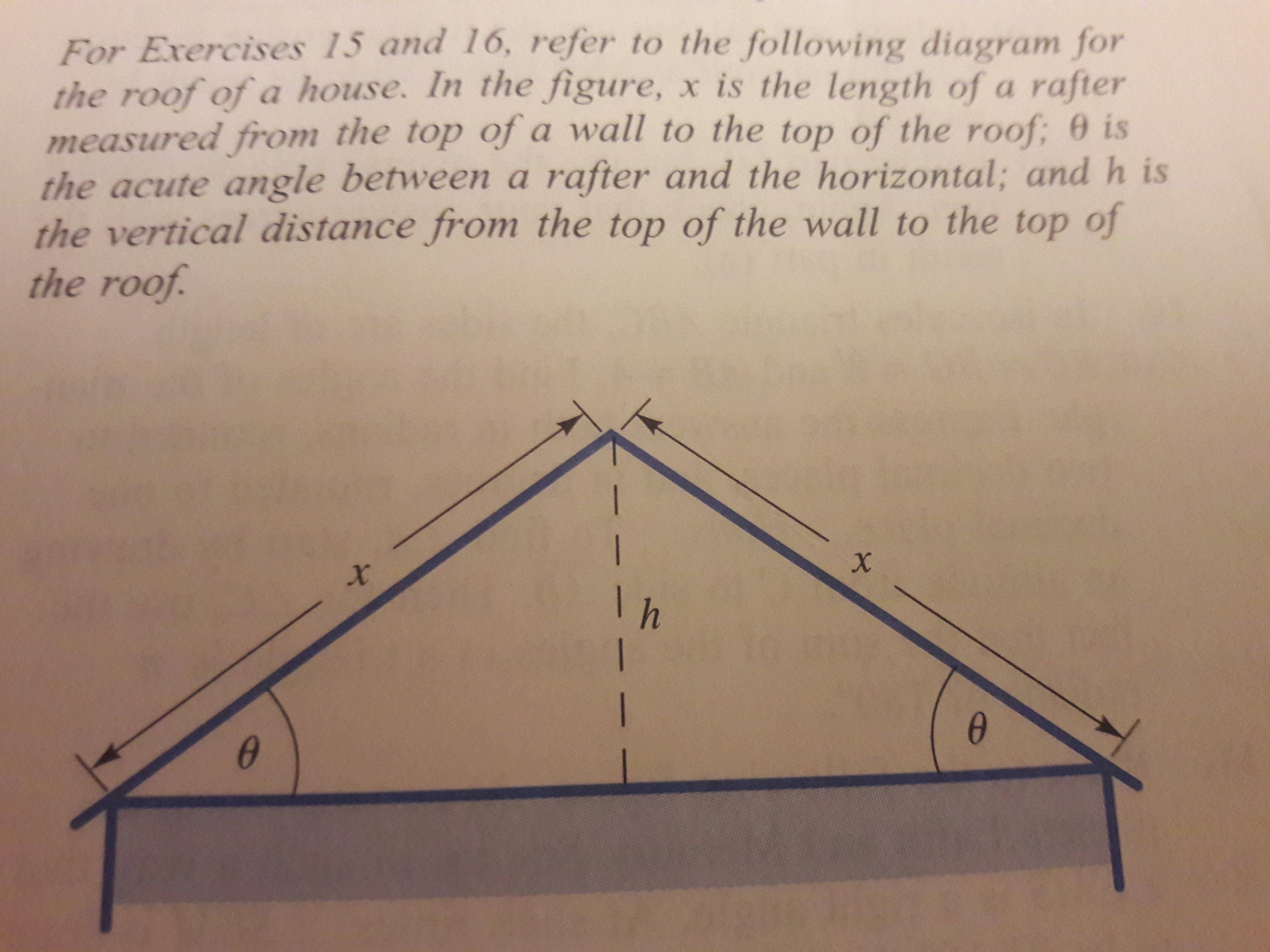
Suppose that theta = 39.4° and x = 43.0 feet. Find h and round answer to one decimal place.

I found h to be 27.3 feet.

The gable is the triangular region bounded by the rafters and the attic floor. Find the area of the gable. Round the final answer to one decimal place.

Before calculating the area, I needed to find the radius, which turns out to be 33.2 feet.

I then used A = (1/2)(base)(height).
My answer is 906.4 ft^2.
The book's answer is 906.9 ft^2.
How do I get the book's answer?

 

[HOI]

I wouldn't use the word "radius" since there is no circle. I presume you mean half the base of the gable. Yes, the height is the "opposite side" of a right triangle with angle 39.4 degrees and hypotenuse 43 feet. h= 43*sin(39.4)= 27.3 feet rounded to one decimal place. One half the base is b= 43*cos(39.4)= 33.2 feet.

The area of the gable is (27.3)(33.2)= 906.4 square feet, not 906.7

 

[skeeter]

$A = \dfrac{1}{2} \cdot 43^2 \cdot \sin[180 - 2(39.4)] \approx 906.9 \, ft^2$

 

[xyz_1965]

I wouldn't use the word "radius" since there is no circle. I presume you mean half the base of the gable. Yes, the height is the "opposite side" of a right triangle with angle 39.4 degrees and hypotenuse 43 feet. h= 43*sin(39.4)= 27.3 feet rounded to one decimal place. One half the base is b= 43*cos(39.4)= 33.2 feet.

The area of the gable is (27.3)(33.2)= 906.4 square feet, not 906.7

Thank you very much. Yes, I meant to say half the base of the gable not radius. Please, read my next thread in the Chat Room and reply.

 

[xyz_1965]

$A = \dfrac{1}{2} \cdot 43^2 \cdot \sin[180 - 2(39.4)] \approx 906.9 \, ft^2$

Simply put. Please, read my next thread in the Chat Room and reply.