- #1
soccerjayl
- 53
- 0
Encountered the following question. For (a), I repetitively found the answer 12 for magnitude of displacement and 131° for the direction; after being told i was incorrect...i need help: 
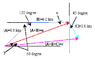
Path A is 8.0 km long heading 60.0° north of east. Path B is 6.0 km long in a direction due east. Path C is 3.0 km long heading 315° counterclockwise from east.
(a) Graphically add the hiker's displacements in the order A, B, C.
What is the magnitude of displacement (km)? What is the direction of displacement (°counterclockwise from East)?
(b) Graphically add the hiker's displacements in the order C, B, A.
What is the magnitude of displacement (km)? What is the direction of displacement (°counterclockwise from East)?
(c) What can you conclude about the resulting displacements?
thanks.
Path A is 8.0 km long heading 60.0° north of east. Path B is 6.0 km long in a direction due east. Path C is 3.0 km long heading 315° counterclockwise from east.
(a) Graphically add the hiker's displacements in the order A, B, C.
What is the magnitude of displacement (km)? What is the direction of displacement (°counterclockwise from East)?
(b) Graphically add the hiker's displacements in the order C, B, A.
What is the magnitude of displacement (km)? What is the direction of displacement (°counterclockwise from East)?
(c) What can you conclude about the resulting displacements?
thanks.