How Do Impulse and Friction Affect Particle Dynamics and Block Motion?

[tommywan410]

I have two question that I don't know how to start.

1st question, three particles A, B and C of equal mass m lie on a horizontal suface at the vertices of an equilateral triangle; they are joined by light inextensible string. An impulse I is applied on C in the direction of BC. Find the speed of C just after the jerk, express the answer in terms of I and m. Calculate the ratio of the instantaneous speeds of B and A. [Hint: Backward impulses will act on particle C through the strings whenever there is an impulse I on C]

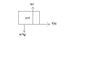
2nd question, A block of mass m1 is placed on the top of another block of mass m2 resting on the floor of an elevator, where m1<m2. The coefficient of static friction between the block is μ(s) and the coefficient of kinetic friction between the lower block and the floor is μ(k), where μ(s)<μ(k). A horizontal force F is applied on the lower block when the elevator is accelerating upward with a magnitude of a. As a result, the blocks move together without relative slipping.(F is in a rightward direction)

(a) Draw the free body diagram of each block
(b) Find the maximum magnitude of F and the acceleration a of the blocks for such case.

My attempt:
1st question: I don't know what is the meaning of an impulse acting on an object, does it mean that there is a force or what? If yes, could I use force to calculate the speeds of the particles. However, for C, I am simply using change of momentum to calculate. So, speed of c= I/m. Am I correct? If yes, how could I link this up with the two remain speeds of A and B.

2nd question: For the free body diagram, am I drawing them right? If no, what are the problems? Also, I don't know how to link up F(max) with f(k) and f(s). Is it something like F(max)-f(k)=<f(s)?

 

[tiny-tim]

welcome to pf!

hi tommywan410! welcome to pf!

1st question: I don't know what is the meaning of an impulse acting on an object, does it mean that there is a force or what? If yes, could I use force to calculate the speeds of the particles.

impulse means a force acting for a time

(we use it when we don't know the force or the time individually, but we do know their product)

impulse diagrams and equations behave exactly like force diagrams and equations, so you can use components and moments in the same way

(and don't forget the impulsive tensions in the strings)

show us what you get ​

Newton's second law for impulses is net impulse = total change of momentum

2nd question: For the free body diagram, am I drawing them right? If no, what are the problems? Also, I don't know how to link up F(max) with f(k) and f(s). Is it something like F(max)-f(k)=<f(s)?

your diagrams are fine (personally, i'd put an acceleration arrow on the side in your FBDs, same as in your overall diagram)

write out the F = ma equations first …

the solution should then be clear​

 

[tommywan410]

thx~ question 5, i already work it out myself! But for question 4, I know impulse=force x time. What really confuses me is how can I relate the impulse I given by the questions and ball C. Is it simply v(c)= I/m, or other equation?
I would like to ask about the tension impulse, are those three the same? And when I solve the tension impulse between A and C, there would a vertical impulse acting on C, so where I got it wrong?

 

[tiny-tim]

hi tommywan410!

What really confuses me is how can I relate the impulse I given by the questions and ball C. Is it simply v(c)= I/m, or other equation?

don't expect to get the actual speeds …

Calculate the ratio of the instantaneous speeds of B and A.

… from the question, you can tell that you'll only get the ratio of speeds

I would like to ask about the tension impulse, are those three the same? And when I solve the tension impulse between A and C, there would a vertical impulse acting on C, so where I got it wrong?

not following you

tension impulse acts along the string, same as ordinary tension

(and don't forget you know the direction of motion of the centre of mass )

 

[tommywan410]

So, according to the graph, is it I-T-Tcos60=change of momentum of C in x-direction
Tsin60= change of momentum of C in y-direction??

About speed of centre of mass, what is its relationship between the speed of C and I. I know the whole system velocity= I/3m