How Do You Apply Zonal Spherical Harmonics in Electromagnetism Problems?

[Zaitul Hidayat]

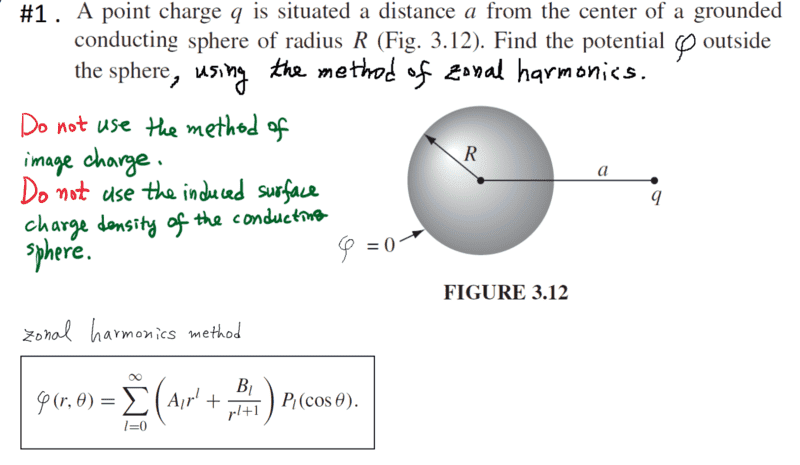
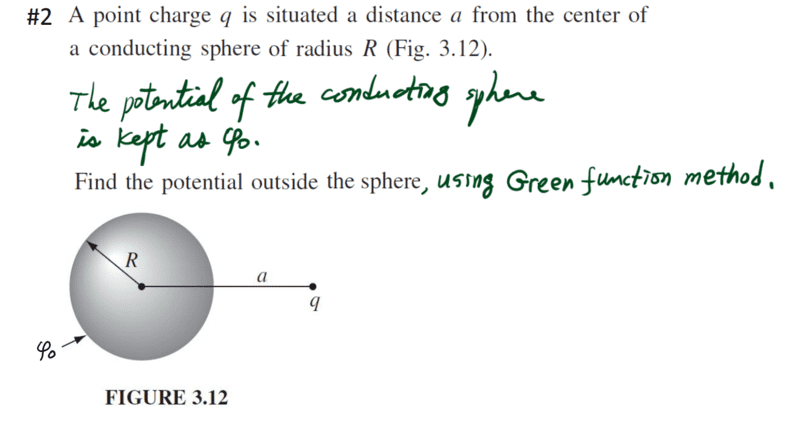
I don't really understand how to find the solution. I've tried to find the solution in books and google but still can't find it. In general, the Question 1 the problem is using the method of Image charge and Induced surface charge density. but this time my professor changed it to something else. can you guys help me? Thank You.

 

[MathematicalPhysicist]

Which books have you searched for a solution to problem 1?

 

[MathematicalPhysicist]

But you can do this exercise by noticing the boundary condition: $\varphi=0$ on the sphere and $\varphi=0$ at infinity; and plugging the Zonal Spherical Harmonics.

 

[Zaitul Hidayat]

Which books have you searched for a solution to problem 1?

I'm not sure which book I read, because I just googled it. and I found some questions that are very similar but only different methods are used.

 

[Zaitul Hidayat]

But you can do this exercise by noticing the boundary condition: $\varphi=0$ on the sphere and $\varphi=0$ at infinity; and plugging the Zonal Spherical Harmonics.

but I still don't understand how I plugged the Zonal Spherical Harmonics into it

 

[MathematicalPhysicist]

but I still don't understand how I plugged the Zonal Spherical Harmonics into it

At $r=R$ you get $\varphi(R,\theta)=0$, plug $r=R$ into the Zonal Spherical harmonic and equate to zero. For $\varphi(r=\infty,\theta)=0$, notice that only $P_0(\cos\theta)$ contribution here, since there's no dependence on $\cos \theta$ in the boundary conditions.