How Do You Calculate Counterweights for a Rotating Shaft with Variable Forces?

[Vandeta]

Hi guys,

I'm trying to dimension a counterweight for a rotating shaft to which a disk, with a force applied at it's end, is attached. (Unfortunately I don't know the exact english term - maybe it's called an eccentric?) It's pretty much the same as the counterweights on a crankshaft (or atleast I think they're counterweights...).

Can someone please explain to me how exactly I should go about this, because I have been thinking for quite a while now. My assumption would be that I have to go with:

M1 = - M2 and then just m1*g*r1 = - F*r2...
Seems far too simple to me, so I kind of don't believe it. Furthermore, the force applied is not constant, but instead is in the form of a sine wave.
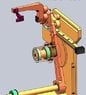
I would like the shape of the counterweight to be like that of the counterweight in the red rectangle in the attached file. I would also like to ask, how does one determine the center of mass of such an object?

The questions are coming from a soon to be an engineer...

I really hope I have explained the problem thoroughly. If anyone has any questions please ask. And if someone takes the time and helps me out on this one it would be amazing.

Thanks in advance :)

 

[Dr.D]

This question does not have an entirely simple answer, but instead it depends upon your objective. Specifically, do you want to balance the crank shaft alone, or are you trying to balance the crank-con rod-piston assembly?

It is possible to perfectly balance the crank alone, but it is not possible to entirely balance the crank-con rod-piston assembly.

Can you tell us more clearly what your objective is so that we can try to be of more help, please?

 

[Vandeta]

I'm uploading a picture of the 3D model of the ... problem. (not very high quality, but I'm sure you'll get the point)

The shaft in the middle is being rotated and thus rotates the disk (transperent). To the disk is a lager attached that goes into the middle of the big arm. So the arm is being rotated back and forth creating a translation of the sled (not very clearly visible, but veery light blue in colour) along the rail (clearly visible - yellow with holes in it).
So the thing is that I'd like to put a counterweight on the rotating shaft because of the oscillations. (i'm not sure if i expressed myself in the best way, but I still hope that you get the point).

We're talking about a rotation of the shaft at about 300rpm, so I'm pretty confident that a counterweight is needed.

Hope that makes it clearer :)
If you have any other questions please, do ask!

Thanks a lot.

 

[Stephen Tashi]

To the disk is a lager attached that goes into the middle of the big arm.

I can't resist asking whether the term "lager" (for a bearing) is specific to this kind of situation ( rotation to translational motion, a bearing that rolls in a slot.)

Assuming there is clearance in the slot for the lager bearing to roll instead of slide then as it transitions from rolling down to the slot to rolling up, will there be short interval when it leaves contact with one side os the slot and slaps the opposite side of the slot?

So the arm is being rotated back and forth creating a translation of the sled (not very clearly visible, but veery light blue in colour) along the rail (clearly visible - yellow with holes in it).

One use of a counterweight could be to even-out the load on the motor, or whatever is supplying the motive power to the machine.

 

[Dr.D]

Wow! You second figure looks nothing like the engine mechanism you posted first, but whatever...

The basic principle for balancing any part is to move the center of mass to the axis of rotation. You can find the center of mass without the counter weight, then calculate how much additional mass in a convenient location will result in the combined center of mass being on the axis of rotation,

 

[Vandeta]

To Stephen Tashi:

Yeah, sorry, I did mean a bearing and not a lager (studying in switzerland sorry...)

I have to tell you that I don't really exactly know why there should be a counterweight (I have some gut feeling that there should be, but not an exact physical explanation to it)... so I would be very grateful if you guys could explain this to me... What problems could a counterweight solve and how does one actually do that (what would "to even-out the load on the motor" mean?)?

To Dr.D:

I did guess that my first explanation isn't very good so I uploaded a picture of the real problem... Sorry for the caused confusion.

What I can understand from your explanation is pretty much what I wrote -> The moment that the force at the bearing is creating around the shaft = - the moment that the counterweight creates, again, around the shaft
So M1=-M2
and we have the moment of the counterweight: m*g*r (r being the radius from the shaft to the center of mass of the counterweight)

As I already said... seems far too simple to me. And also there is the thing that the force at the bearing isn't a constant, (but then again the moment created by the counterweight isn't a constant as well...).

Can you guys tell me if I'm on the right track and if so, how do I consider the thing that the moment of m*g (of the counterweight) is constantly changing and it should equal the - again changing - moment created by the force?

I know it's quite a lot I'm asking here, but... hope you guys are kind enough to help :) thanks.

 

[OldEngr63]

Counter weights operate on the idea of centrifugal force, not gravitation.

 

[Vandeta]

oh, god, that's my bad here... I've been considering the static case and I have completely forgotten that we're actually going at 300rpm...

Soo in that case we have the mass of the counterweight times the radial(normal) acceleration. Then I have to find the magnitude of the force applied to the bearing in radial direction and set them equal right?
Anybody got some ideas as to how I can calculate the force (applied at the bearing) in radial direction? (the centrifugal force is quite easy, but this seems a lot more complicated)?

 

[OldEngr63]

If I am understanding correctly, your second figure looks like a variation on what has traditionally been called the "shaper mechanism" because it is the basis for the machine tool called a shaper. To the best of my knowledge, there is no way to balance a shaper mechanism, although a good size flywheel could be beneficial in many cases. The shaper is one of the several inversions of the basic slider-crank mechanism.

One thing that is not clear in your figure is the matter of the yellow shaft at the bottom of the figure. Is it mounted in fixed bearings, or does the shaft center line translate?

What is the drive point in your mechanism? Is it the stubby yellow shaft with the green bearing near the center of the figure? What does the mechanism do (what is its function)?

 

[Stephen Tashi]

Design it so whatever counterweight is put on it can be easily removed and replaced with a different counterweight - in case you must resort to trial and error !

Counterweights can have some effect as "counterbalances". A "point mass attached to a horizontal shaft by an arm exerts varying torque. For example when the arm is vertical, the weight of the mass isn't supplying any torque about the shaft. In other positions the weight does exert a torque. The torque that the load demands from your shaft may be so large that it would be impractical to use a counterweight as a counterbalance.

Any sort of mass attached to the shaft (e.g. a flywheel) has a moment of intertia about the shaft, so it "resists" changes in angular velocity. It exerts a torque that "helps" the motor when the rotation is slowing down and demainds more of the motor when rotation is speeding up. It tends to even out the torque demanded (as a function of time) from a motor that's keeping the shaft rotating at a constant angular velocity.

The balancing of a rotating machine about the axis of a shaft concerns the force on the shaft, not merely the torques on it. Think of the familiar textbook scenario "A mass m is moving in a circular orbit of radius R about the orgin at constant angular velocity. It is held in orbit by a string attached to the origin...". There is a tension on the string. At a given instant of time, the string is pulling the origin toward the mass, so, as far as the origin is concerned, the situation is unbalanced.

To conceptualize balancing a crankshaft, imagine a rigid bar with one end pivoting about the origin. Some one grabs the free end of the bar and does a complicated series of maneuvers that both pull and push on the bar and exert torques on its free end. The forces exerted on the orgin are not longer given by a vector of constant magnitude rotating at a constant velocity. (The forces exerted by a rod on the crankshaft in an automobile engine would be complicated. It would vary depending on whether it was a compression stroke, power stroke etc. as well as the changing reaction forces at rod bearings due the geometry of the situation.)

The complexity of the situation doesn't rule out the utility of a counter weight. For example, a purely mathematical question could be posed: Suppose we are given the vector valued function of F(t) of force vs time that describes the forces exerted on the origin (the axis of the shaft). Assume the shaft is rotating at a constant velocity. Find a vector valued function B(t) which describes a vector of constant magnitude rotating as the crankshaft does such that F(t) + B(t) has less strength than F(t) alone. ( Strength could be defined in various ways. For example, it could be defined as the root-mean-square value of magnitude of force vs time over one period. Or it could described as the maximum of force vs time over one period.) As Dr.D indicated, you can't perfectly balance the forces on the shaft in a complicated situation with a counterweight. However, you might lessen the imbalance.

-----
My bias in interpreting the rendering of the machine makes me think of in terms of an automotive or woodworking contraption. When I do that, I don't worry about the stubby shaft vibratiing. I worry most about that poor bearing riding in the slot. It will get the heck beat out of it. I worry about the rod and the joints at the end. If the joints at the end of the rod are "tight" and there is some error in fabricating the machine that puts their axes out-of-parallel or causes the rod not stay within a vertical plane then the rod will get twisted as it moves and the joints at the end will tend to be pulled apart.