How Do You Calculate Tension in a Three-Mass Pulley System?

In summary: Thank you, I will work on determining the direction now.ehildT1 = 1.00*1.4+9.80 = 11.2-T2 = 4.00*1.4-19.6 = -14(thanks for all your help and patience so far btw.)i may be jumping ahead, but in order to find the direction don't you need x and y?As for the direction, it is the direction of the acceleration, which is along the length of the string. So it is either left or right. And the tension in the string always "points" away from the block. ehildthe direction of the acceleration is along the length of the string.
  • #1
lolcheelol
21
0

Homework Statement



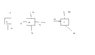
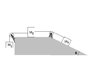
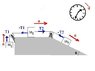
In the figure below, M1=1.00 kg, M2=2.00 kg and M3=4.00 kg. Theta is 30.0°. The pulley and all surfaces are frictionless. Find the tension in the two strings and the direction (entire system to the left/counterclockwise or entire system to the right/clockwise) and magnitude of the acceleration.

Diagram attached.

Homework Equations



F=ma

The Attempt at a Solution



m1=A, m2=B, m3=C
I drew a free-body diagram for each of the blocks and I came up with these equations.
A=T1-W=ma
B=-T1-W+Fn+T2=ma
C=-T2-W+Fn=ma (I rotated it to have the block on a straight surface)

After doing these steps I am completely lost. I think I did my force equations correctly, but if someone can help me with this, step by step, it would be GREATLY appreciated.
 

Attachments

  • freebody.jpg
    freebody.jpg
    5 KB · Views: 478
  • physicstension.jpg
    physicstension.jpg
    5.6 KB · Views: 497
Physics news on Phys.org
  • #2
Remember, force is vector quantity. The components add up separately.
You can take this as a one-dimensional problem, considering the motion along the string. The force components parallel to the strings add up to ma, where a is the acceleration in the direction of the string: If A accelerates upward, B will accelerate to the right and C accelerate down the slope.
The normal force components cancel with gravity. You do not need to include them into the equations.

C feels a force component along he slope due to gravity. That is missing from equation C.


ehild
 
  • #3
ehild said:
Remember, force is vector quantity. The components add up separately.
You can take this as a one-dimensional problem, considering the motion along the string. The force components parallel to the strings add up to ma, where a is the acceleration in the direction of the string: If A accelerates upward, B will accelerate to the right and C accelerate down the slope.
The normal force components cancel with gravity. You do not need to include them into the equations.

C feels a force component along he slope due to gravity. That is missing from equation C.

ehild

so you would separate them into Fx and Fy vectors?

Fx = -T1+T2 =ma
Fy = T1 - W = ma

does that look correct?
 
  • #4
lolcheelol said:
so you would separate them into Fx and Fy vectors?

Fx = -T1+T2 =ma
Fy = T1 - W = ma

does that look correct?

I said to relate the acclerations and forces parallel with the string for each blocks. I do not understand what your your equations mean. Do they refer to the same mass?

ehild
 
  • #5
they were the x components from all 3 blocks and the y components from the blocks. I'm not sure how to do what you're saying to do, any help?
 
  • #6
These are your equations from post 1.

A:T1-W1=m1a
B:-T1[STRIKE]-W+Fn[/STRIKE]+T2=m2a
C=-T2[STRIKE]-W+Fn[/STRIKE]+Fslope=m3a

Omit the Fn-W terms.

Do you know what force acts downward along the slope, because of gravity?

ehild
 
  • #7
kinetic friction? i thought it would have been mgsin∅
 
  • #8
It is not kinetic friction, but it is m3gsin(theta). Substitute the values given for the masses and for theta and write up the equations again with the numbers.


ehild
 
  • #9
A:T1-(9.8)(1.00)=(1.00)a
B:-T1+T2=(2.00)a
C=-T2+(4.00)(9.8)(sin(30))=(4.00)a

do these look correct?
 
  • #10
When you have calculated the acceleration a useful double check is to look at the whole system and recognise that T1 and T2 are INTERNAL forces. There are only 2 external forces (W1 and Fslope) and it is the resultant of these 2 forces that cause acceleration of the TOTAL mass. You should get the same acceleration !but this will not give you T1 and T2.
You need the full analysis as outlined by ehild.
 

Attachments

  • forces.jpg
    forces.jpg
    36.5 KB · Views: 523
  • #11
lolcheelol said:
A:T1-(9.8)(1.00)=(1.00)a
B:-T1+T2=(2.00)a
C=-T2+(4.00)(9.8)(sin(30))=(4.00)a

do these look correct?

Good!Now the trick comes: Add all three equation. What do you get?

ehild
 
  • #12
T1-(9.8)(1.00)=(1.00)a
T1+T2=(2.00)a
T2+(4.00)(9.8)(sin(30))=(4.00)a

T1-(9.8)=(1.00)a --- T1 = (1.00)a/(9.8)
T2+19.6=(4.00)a ---- T2 = (4.00)a/(19.6)

Insert T1 and T2 into 2nd equation

(1.00)a/(9.8) + (4.00)a/(19.6) = (2.00)a
 
  • #13
lolcheelol said:
T1-(9.8)(1.00)=(1.00)a
-T1+T2=(2.00)a
-T2+(4.00)(9.8)(sin(30))=(4.00)a

T1-(9.8)=(1.00)a --- T1 = (1.00)a/(9.8) wrong!

You missed some minuses. And you did not isolate T1 and T2 correctly.


ehild
 
  • #14
T1 = (1.00)a + 9.80
-T2 = (4.00)a - 19.6

that better?
 
  • #15
OK. Substitute T1 and T2 into the second equation.

ehild
 
  • #16
i come up with

-(1.00a + 9.80)-(4.00a-19.6)=2.00a
-29.4 - 5a = 2.00a
-29.4 = 7a
-4.2 = a

does that look correct? would that be the magnitude of the acceleration?
 
  • #17
lolcheelol said:
i come up with

-(1.00a + 9.80)-(4.00a-19.6)=2.00a
-29.4 - 5a = 2.00a
-29.4 = 7a
-4.2 = a

does that look correct? would that be the magnitude of the acceleration?

No... How did you get -29.4?

Resolving the parentheses: -1.00a - 9.80-4.00a+19.6=2.00a

ehild
 
  • #18
sorry, math error. i end up with a = 1.4

(-9.80+19.6) = 9.8 --- (-1.00a-4.00a) = -5a
9.8 - 5a = 2.00a
9.8 = 7a
1.4=a
 
  • #19
Correct. And the direction you assumed proved to be correct.
Now find the tensions.ehild
 
  • #20
T1 = 1.00*1.4+9.80 = 11.2
-T2 = 4.00*1.4-19.6 = -14

(thanks for all your help and patience so far btw.)

i may be jumping ahead, but in order to find the direction don't you need x and y?
 
  • #21
Well, so T1 = 11.2 N and T2=14 N. Do not forget the units.

As for the direction of acceleration, it is the acceleration of the whole system, along the length of the string instead of x and y direction. The string defines the direction, it is "x" and there is no y. That is what the problem said:
Find the direction (entire system to the left/counterclockwise or entire system to the right/clockwise) and magnitude of the acceleration.
So is it clockwise or anticlockwise?

ehild
 
  • #22
i think counterclockwise because the tension is positive.
 
  • #23
What is counterclockwise? The tension is always positive, it can not be negative.

ehild
 
  • #24
it would be going to the right(clockwise) because T2>T1
 
  • #25
Well done!
If you look at the external forces you see the resultant = 9.81N
The total mass is 7kg therefore acceleration = F/m = 9.81/7 = 1.4ms^-2
Now you know the acceleration you can apply F = ma to each mass to double check that T1 = 11.2N and T2 = 14.02N (I would round this off to 14.0N)
It is accelerating in the direction that I have taken to be +ve.
 

Attachments

  • forces.jpg
    forces.jpg
    37.8 KB · Views: 416
  • #26
thanks for all your assistance guys, i really appreciate it.
 
  • #27
Clockwise is the right direction!

ehild
 

Attachments

  • slope3.JPG
    slope3.JPG
    13.2 KB · Views: 355

FAQ: How Do You Calculate Tension in a Three-Mass Pulley System?

What causes tension in a pulley system?

Tension in a pulley system is caused by the weight of objects being lifted or moved by the system. This weight creates a downward force, which is counteracted by tension in the ropes or cables of the system.

How is tension calculated in a pulley system?

Tension in a pulley system can be calculated using the principle of mechanical advantage. The force required to lift an object is equal to the weight of the object divided by the number of ropes supporting it.

What factors affect tension in a pulley system?

The main factors that affect tension in a pulley system are the weight of objects being lifted, the number of ropes or cables in the system, and the angle at which the ropes are pulled. Friction and the weight of the pulleys themselves may also affect tension.

How can tension be increased in a pulley system?

Tension in a pulley system can be increased by adding additional ropes or cables to the system, increasing the angle at which the ropes are pulled, or using a larger pulley. However, these changes may also increase the amount of friction in the system.

What are the practical applications of understanding tension in a pulley system?

Understanding tension in a pulley system is important in various fields such as engineering, physics, and mechanics. It is used in the design and construction of cranes, elevators, and other lifting devices. It is also important in understanding the principles of mechanical advantage and calculating the force required to lift heavy objects.

Similar threads

Newton's Second Law (Pulley Sustem)
Replies
3
Views
1K
Problem with two pulleys and three masses
3
Replies
102
Views
6K
Accelerating pulleys (3 pulleys and 3 masses)
Replies
5
Views
1K
System with Pulleys and a Rope That Might Break
Replies
26
Views
2K
Pulleys with Torque: Free Body Diagram Analysis
Replies
34
Views
3K
Calculate the initial acceleration of this pulley system
Replies
8
Views
2K
System of two pulleys and three masses
Replies
22
Views
4K
Solve Pulley-Mass System: m1=4.00 kg, m2=1.00 kg
Replies
2
Views
1K
Calculating tensions and acceleration
Replies
10
Views
2K
Calculating Tension in a System of Trolleys
Replies
18
Views
2K

Hot Threads

Recent Insights

Back
Top