karush
Gold Member
MHB
- 3,240
- 5
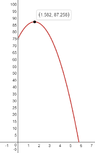
A stone is thrown vertically upward with a speed of 15.5 m/s
from the edge of a cliff 75.0 m high
ok, I was going to graph this with Desmos first
but don't know how to derive the equation for the parabola.
there are 11 questions following this...$$\tiny{Embry-Riddle \, Aeronautical \, University, \, HW3 \, PS103}$$
from the edge of a cliff 75.0 m high
ok, I was going to graph this with Desmos first
but don't know how to derive the equation for the parabola.
there are 11 questions following this...$$\tiny{Embry-Riddle \, Aeronautical \, University, \, HW3 \, PS103}$$
Last edited: