harpazo
- 208
- 16
Set up an integral for both orders of integration.
DO NOT EVALUATE THE INTEGRAL.
Let S S = double integrals
Let R = region
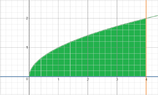
S S y/(1 + x^2) dA
R: region bounded by y = 0, y = sqrt{x}, x = 4
I can graph the region but have no idea how to proceed from there. I need solution steps.
DO NOT EVALUATE THE INTEGRAL.
Let S S = double integrals
Let R = region
S S y/(1 + x^2) dA
R: region bounded by y = 0, y = sqrt{x}, x = 4
I can graph the region but have no idea how to proceed from there. I need solution steps.