- #1
Precursor
- 222
- 0
Homework Statement
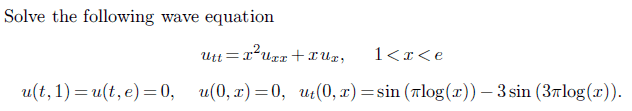 The attempt at a solution
The attempt at a solution
I'm using the method of separation of variables by first defining the solution as [itex]u(x,t) =X(x)T(t)[/itex]
Putting this back into the PDE I get: [itex]T''X = x^{2}X''T + xX'T[/itex]
which is simplified to [tex]\frac{T''}{T} = \frac{x^{2}X'' + xX'}{X} = -\lambda^{2}[/tex]
The spatial problem is then: [itex]x^{2}X'' + xX' = -X\lambda^{2}[/itex]
Is this correct so far? How do I continue?
I'm using the method of separation of variables by first defining the solution as [itex]u(x,t) =X(x)T(t)[/itex]
Putting this back into the PDE I get: [itex]T''X = x^{2}X''T + xX'T[/itex]
which is simplified to [tex]\frac{T''}{T} = \frac{x^{2}X'' + xX'}{X} = -\lambda^{2}[/tex]
The spatial problem is then: [itex]x^{2}X'' + xX' = -X\lambda^{2}[/itex]
Is this correct so far? How do I continue?