How do you write the -- in Hom(--,B) ?

[quasar987]

TL;DR Summary

I want to know how to write the dash in Hom(--,B)

Hello!

Here's a piece of a print screen I did on Wikipedia's page on "Hom functor":

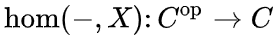
Does anybody knows how to do that dash that's longer than a mere minus sign?

 

[fresh_42]

What is wrong with \operatorname{Hom}(-,X) ?
$\operatorname{Hom}(-,X)$

 

[quasar987]

What is wrong with \operatorname{Hom}(-,X) ?
$\operatorname{Hom}(-,X)$

Not a thing! I was certain that I had tried that in Overleaf and it didn't look good. But now I tried it and it looks fine.