- #1
Weatherkid11
- 18
- 0
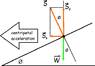
A train is running smoothly along a curved track at the rate of 50 m/s. A passenger standing on a set of scales observes that his weight is 10% greater than when the train is at rest. The track is banked so that the force acting on the passenger is normal to the floor of the train. What is the radius of curvature of the track?
I think i use the equation
F= [(GMm)/r^2](r/r), and Multiply the little m by .10, and try to solve for r
I think i use the equation
F= [(GMm)/r^2](r/r), and Multiply the little m by .10, and try to solve for r
Last edited: