- #1
ahazen
- 49
- 0
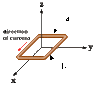
A rectanglular loop consists of 5 turns of wire carrying a current of 2.8 A. The loop is in the x-y plane, and the direction of flow of the current is shown in the figure. The loop has dimensions a = 4 cm and b = 5 cm. Consider a uniform magnetic field of strength 3.8 × 10-4 T in x, y, or z directions.
I need to find the:
-Fa on x-axis
-Fb on y-axis
-Fa on z-axis
-Fb on z-axis
For the X-axis, I found that torque is 1.064e-5 and Fb=0
For the y-axis, I found the torque is 1.064e-5 and Fa=0
I also found that the torque for z-axis is 0.
But, how do I find the other Fa and Fb?
I am given: F=I*L*B sin(theta)
I need to find the:
-Fa on x-axis
-Fb on y-axis
-Fa on z-axis
-Fb on z-axis
For the X-axis, I found that torque is 1.064e-5 and Fb=0
For the y-axis, I found the torque is 1.064e-5 and Fa=0
I also found that the torque for z-axis is 0.
But, how do I find the other Fa and Fb?
I am given: F=I*L*B sin(theta)