ChuFta
- 4
- 0
New user has been reminded to always show their work on schoolwork problems.
- Homework Statement
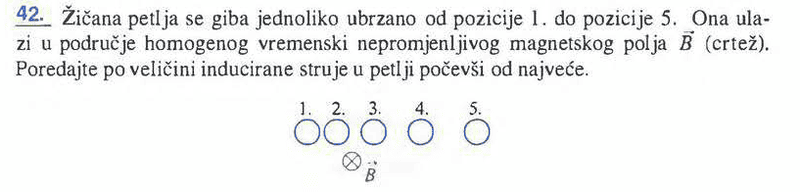
- A wire loop accelerates from position 1 to position 5. It enters an area of a homogenous non changing magnetic field B. Rank the induced currents in the loop starting from the biggest
- Relevant Equations
- /
A wire loop accelerates from position 1 to position 5. It enters an area of a homogenous non changing magnetic field B. Rank the induced currents in the loop starting from the biggest. in solutions it says I4>I2>I1=I3=I5=0 but i dont understand why