How Does Weight Distribution Affect Wheel Spin Duration and Speed?

[dascribe]

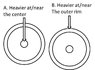
If I make 2 wheels of identical size and weight, so that the only difference bewteen the 2 is that wheel A has most of its weight at the center of the wheel and wheel B has most of its weight equally distributed on the circumference of the wheel:

1. Which wheel would spin longer if both wheels were initially spun with equal force?
2. Would one wheel spin faster than the other given the same force?


***The wheel is placed horizontally like a top

thanks

 

[mitch_1211]

It will depend on the moment of inertia of each wheel, a list of common configurations can be found here http://en.wikipedia.org/wiki/List_of_moments_of_inertia

The best relationship to use is probably L = Iw
Where;
L - angular momentum
I - moment of inertia
w (omega) - angular velocity

Hope this helps you out a little bit

 

[dascribe]

Hi Mitch,

Thanks for responding.

I'm not that strong when it comes to formulas, so I'm not sure what the answer is based on the link.

I'll re-phrase the question because I'm not sure that the term interia was correct vs momentum, or something else.

Assuming both wheels are the same, and both wheels are spun from close to the center, and both wheels are spun with the same amount of initial energy, would a wheel with most of its weigh near the center of the wheel spin longer than a wheel that has most of its weight at near the circumference of the circle.

I think that the wheel with with more weight in the cetner would move more quickly initially and possibly come to a stop first because it used more of the energy up front.

Then again, maybe they would stop at the same time, or close to it.

 

[cryora]

Well if you set an object into spinning motion, Newton's first law of inertia states that the object will keep spinning at its same rate unless acted upon by an outside force. Rotational force is known as "torque" so by mentioning that the wheels are going to slow down and stop implies that there is a net torque acting on the wheels opposite to the direction of spin. I suppose this could be like a frictional force. But let's assume that there is such a force.

With this in mind, the wheel with the greater angular momentum will spin longer than the wheel with less angular momentum if a net torque is applied to each wheel equally. You said that both wheels are spun with equal energy. I'm not exactly comfortable with the word energy, because I'm not sure I want to get into energy considerations right now, so let's say, instead, that the wheels are spun with the same amount of torque over the same interval of time. This means that the angular momentum will be the same for each wheel, but the wheels will be spinning at a different rate. The wheel with the greater moment of inertia will spin slower and the wheel with the lower moment of inertia will spin faster. Now let's say you apply a net "frictional" torque of equal magnitude to each wheel in the opposite direction of their spin. Both wheels should come to a stop at the same time, since they both have the same angular momentum, and torque is merely the change in angular momentum over change in time, dL/dt.

But let's say you spin both wheels so that both are spinning at the same rate. This means that the wheel with the greater moment of inertia will have greater angular momentum than the wheel with the lower moment of inertia. Inertia is resistance to change in motion, so the wheel with the greater moment of inertia will be less wanting to slow down as the other wheel, and so the wheel with the greater moment of inertia will be spinning longer than the other wheel should an net torque act in the opposite direction of spin on each wheel equally.

Moment of inertia is an object's resistance to spinning, and it depends on how the mass of the object is distributed. So if you look at the list of moments of inertia, the moment of inertia of the wheel with most of its mass at its center can be approximated by the solid cylinder, and it has a much smaller radius. As for the wheel with most of its mass on the outside, its moment of inertia can be approximated by the thin cylindrical shell with open ends, and its radius would be significantly larger than that of the solid cylinder.

If you look at the formulas, the solid cylinder already has a smaller inertia (mr^2)/2 as opposed to that of the thin cylindrical shell mr^2 even with the same radius. So by reducing the radius of the solid cylinder, you're only making its moment of inertia even smaller.

 

[dascribe]

Thanks for responding.

So if the weight of the object of was evenly distributed, the cylinder with the greater radius would spin longer.

Just to confirm, you are saying that even if the 2 wheels had an identical radius, the wheel with more weight at the circumference would last longer, correct?

I guess that makes sense.
When the center is the driving force behind the spin, it has to move the circumference mass a greater distance than if the circumference holds the bulk of the momentum and has to drive the movement of the center.

Given that same concept, it would probably require more energy to get the heavy circumference wheel to the same speed as the one with a heavy center because the center weight has to move less distance to gain the same rotating speed of the circumference.

 

[jetwaterluffy]

I'd say that if you set them off with the same torque, then the one with central mass should spin faster, and therefore will generate a greater friction/ air resistance force, so should stop first.

 

[cryora]

So if the weight of the object of was evenly distributed, the cylinder with the greater radius would spin longer.

Just to confirm, you are saying that even if the 2 wheels had an identical radius, the wheel with more weight at the circumference would last longer, correct?

Yes that is assuming that they both start spinning at the same rate. However if you gave the same amount of force, then the cylinder with the greater radius would spin slower, but they would both stop at the same time assuming the resistive forces are equal. But jetwaterluffy brings up a good point. If the resistive forces is greater for an object spinning faster, then the initially faster spinning object would overall lose more momentum over time.