- #1
Rheegeaux
- 6
- 0
[Note: Post moved to homework forum by mentor]
So I stumbled upon a reviewer for my physics exam tomorrow and I was wondering how the equation was formulated. Your help is very much appreciated :) ! Normally I would consult my professor for this but it's Sunday in my country today so I can't.
Question:
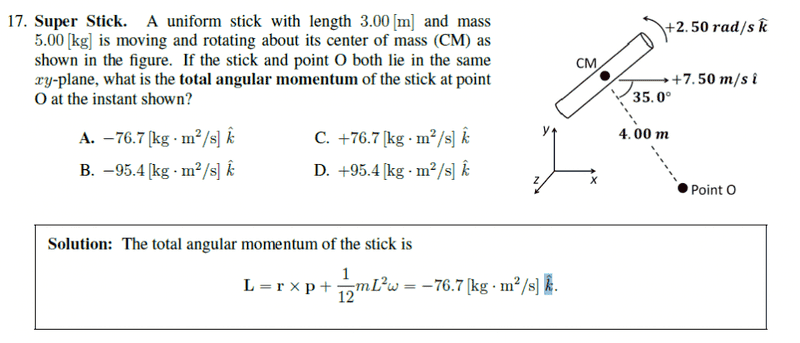
A uniform stick with length 3.00 [m] and mass
5.00 [kg] is moving and rotating about its center of mass (CM) as
shown in the figure. If the stick and point O both lie in the same
xy-plane, what is the total angular momentum of the stick at point
O at the instant shown?
answer:
L = (r )(p) +1/12mL^2(w) = -76.7kmm^2/s positive k hat
Picture: http://postimg.org/image/u5eeo77el/96cf1d1b/
So I stumbled upon a reviewer for my physics exam tomorrow and I was wondering how the equation was formulated. Your help is very much appreciated :) ! Normally I would consult my professor for this but it's Sunday in my country today so I can't.
Question:
A uniform stick with length 3.00 [m] and mass
5.00 [kg] is moving and rotating about its center of mass (CM) as
shown in the figure. If the stick and point O both lie in the same
xy-plane, what is the total angular momentum of the stick at point
O at the instant shown?
answer:
L = (r )(p) +1/12mL^2(w) = -76.7kmm^2/s positive k hat
Picture: http://postimg.org/image/u5eeo77el/96cf1d1b/
Last edited by a moderator: