WMDhamnekar
MHB
- 376
- 28
How did the author compute the highlighted term 2 from the highlighted term 1 in the following answer to the given question?
If $\rho =\frac{d\psi}{ds}$, then the term 2 should be $\upsilon^2 \frac{d\hat{T}}{d\psi}\rho$, but instead, it was written $\frac{\upsilon^2}{\rho}\frac{d\hat{T}}{d\psi}$
How is that computed? How to compute radius of curvature($\kappa$) if $\frac{d\hat{T}}{ds}= \kappa\hat{N}$
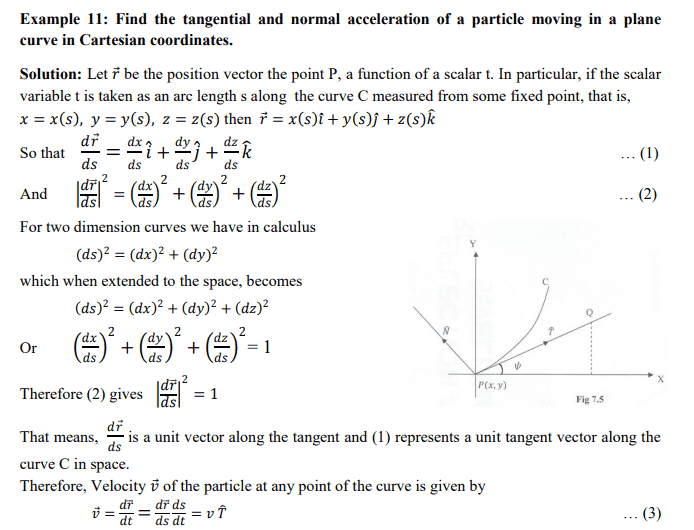
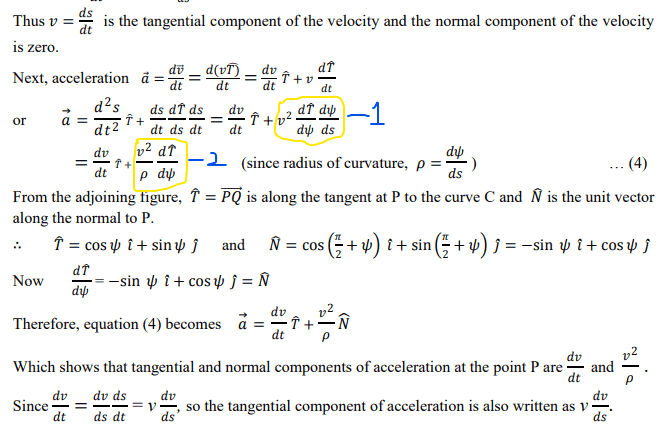
If $\rho =\frac{d\psi}{ds}$, then the term 2 should be $\upsilon^2 \frac{d\hat{T}}{d\psi}\rho$, but instead, it was written $\frac{\upsilon^2}{\rho}\frac{d\hat{T}}{d\psi}$
How is that computed? How to compute radius of curvature($\kappa$) if $\frac{d\hat{T}}{ds}= \kappa\hat{N}$
Last edited: