How is the Angle of Deflection in a Prism Calculated?

[Lindsayyyy]

Hi,

Can anyone tell me how I get to the angle of deflection in a prism?

How do I get to

\delta=\alpha_{1}-\beta_{1}+\alpha_{2}-\beta_{2}

Thanks for the help

 

[xts]

Reading forum rules:
DO NOT POST YOUR HOMEWORK/COURSEWORK QUESTIONS HERE
and posting your question at the right place.

 

[I like Serena]

Hey Lindsayyyy!

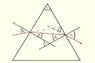
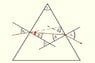
Can you tell what the angles are in the top triangle, bounded by the dotted lines and the red line?

 

[I like Serena]

Reading forum rules:
DO NOT POST YOUR HOMEWORK/COURSEWORK QUESTIONS HERE
and posting your question at the right place.

Ah yes, I missed that.
I'll ask a moderator to move this thread.

 

[Lindsayyyy]

Well, this is for understanind purpose. I thought homework section is actually for homework.
And actually I can't tell you :( I'm quite bad at trigonometry. I know that the sum in an angle is 180° but I don't know how I can express the unknown angles. I guess I find ,for example, alpha1 etc on another place in the drawing again, but I have no idea :/

 

[I like Serena]

Ah, but the homework section is also for coursework, and I think this qualifies.

Okay, let's take it a step back.
If we look at the angle α₁.
What would be the angle opposite to this angle?
That is the angle on the inside of the prism, between the normal and the dotted line?

(Do you know what I mean with "the normal"?)

 

[I like Serena]

Let me mark it in the drawing.

Can you tell me what the angle marked with red is?

 

[Lindsayyyy]

This is so distressing, I guess I should rather exmatricualte myself :/ I can't.

edit: just forget about it, this is senseless, would take hours for me to understand this, don't want to waste anyones time with this **** to be honest

 

[I like Serena]

Well, it's certainly not my intention to distress you.
But I don't feel like my time is wasted, so as long as you're up to it, so am I. :shy:

Actually, you should be able to see that the angle that I marked in red is about the same size as α₁.
And it is the same size!

Are you? Up to it I mean?
If you don't want to continue that's fine too of course.

 

[Lindsayyyy]

as my english isn't that good, I'd rather say embarrasing instead of distressing. That I'am not even able to see such easy things. Goes on and on, as you already see in my other threads :/ I'm off to bed, thanks for your help though

 

[I like Serena]

All right.
If you change your mind and you do want to continue, just post in this thread.

Sleep well.

P.S.: and no, the only thing I saw in your other threads was an interest to learn stuff like electronics.