- #1
SparkimusPrime
- 35
- 0
Excerpt from:
Beicher and Serway, "Physics for Scientists and Engineers with
Modern Physics, 5th edition"
Chapter 24, Problem 53, part e:
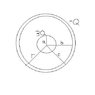
A solid insulating sphere of radius a carries a net positive charge 3Q, uniformly distributed throughout its volume. Concentric with this sphere is a conducting spherical shell with inner radius b and outer radius c, and having a net charge -Q, as shown...
(e) construct a spherical Gaussian surface of radius r, where
b < r < c, and find the net charge enclosed by this surface.
So far, I can safely assume that both charged surfaces can be treated as point charges independently. But together their net electric field is 2Qke / r^2 (where r is a radius of a Gaussian surface greater than length c) and their net charge is 2Q. The outer sphere (since its a conductor) has an internal electric field equal and opposite to the inner electric field (due to conservation of energy). The net electric field is base on a net charge of 2Q, but I'm not sure how to approach that charge distribution on the inside of the outer sphere. It seems that the excess charge is pulled to the inner surface of the outer sphere due to induction (caused by the inner electric force), so should the total charge for part e, be 2Q as well? Or is the net charge inside the outer sphere zero and the total net charge equal to the inner sphere's charge?
Beicher and Serway, "Physics for Scientists and Engineers with
Modern Physics, 5th edition"
Chapter 24, Problem 53, part e:
A solid insulating sphere of radius a carries a net positive charge 3Q, uniformly distributed throughout its volume. Concentric with this sphere is a conducting spherical shell with inner radius b and outer radius c, and having a net charge -Q, as shown...
(e) construct a spherical Gaussian surface of radius r, where
b < r < c, and find the net charge enclosed by this surface.
So far, I can safely assume that both charged surfaces can be treated as point charges independently. But together their net electric field is 2Qke / r^2 (where r is a radius of a Gaussian surface greater than length c) and their net charge is 2Q. The outer sphere (since its a conductor) has an internal electric field equal and opposite to the inner electric field (due to conservation of energy). The net electric field is base on a net charge of 2Q, but I'm not sure how to approach that charge distribution on the inside of the outer sphere. It seems that the excess charge is pulled to the inner surface of the outer sphere due to induction (caused by the inner electric force), so should the total charge for part e, be 2Q as well? Or is the net charge inside the outer sphere zero and the total net charge equal to the inner sphere's charge?