- #1
Daniel Petka
- 147
- 16
A while ago, I saw an explanation of radio waves. Overall, it's a decent vid. I bumped into some nasty problems.
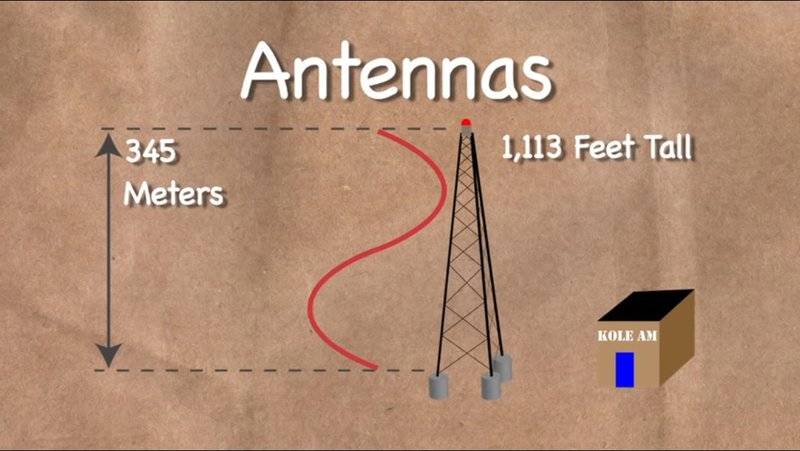
Basically, the image shows that the wavelength of a EM-wave corresponds to the height of the antenna. The visualisation however can't be right since the wave cannot go upwards- the wave's path is oriented 90 degrees to the antenna. So... How.. How the heck should I combine these statements?!
It just keeps bugging me to figure out the right image of a EM wave cause it's extremely counter intuitive and I can't find anything on the net.
This wasn't the first time I came across this problem. Remember the process in a DVD? The red laser passes through holes. It cannon pass holes smaller than its wavelength (hole~antenna). Thus, you need a shorter wavelength- for example violet with 405nm in a BluRay.
Again I have trouble with this phenomenon. Please show me some useable sources if you've found some.
Basically, the image shows that the wavelength of a EM-wave corresponds to the height of the antenna. The visualisation however can't be right since the wave cannot go upwards- the wave's path is oriented 90 degrees to the antenna. So... How.. How the heck should I combine these statements?!
It just keeps bugging me to figure out the right image of a EM wave cause it's extremely counter intuitive and I can't find anything on the net.
This wasn't the first time I came across this problem. Remember the process in a DVD? The red laser passes through holes. It cannon pass holes smaller than its wavelength (hole~antenna). Thus, you need a shorter wavelength- for example violet with 405nm in a BluRay.
Again I have trouble with this phenomenon. Please show me some useable sources if you've found some.