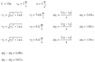How Late Did the Man Arrive to Catch the Train?

[Jenny Physics]

Homework Statement

A man arrives $T$ seconds late to a train station. He watches the last three cars of the train pass by.
The next-to-the-next-to-last car takes time $t$ to pass by the passenger, the next-to-last car takes time $t'$ to pass by and the last car takes time $t''$ to pass by.
Assume the train's acceleration $a$ is constant. What is $T$?

Homework Equations

Kinematics.

The Attempt at a Solution

The train departs at $t=0$ (man's time). At the time $t_{l}=T$ that the man arrives at the train station, the speed of the train is $v_{l}=aT$. If the length of a car is $L$ then at time $t_{2}=t_{l}+t$ we have $L=\frac{1}{2}at^{2}+v_{l}t$, at time $t_{3}=t_{l}+t+t'$ we have $2L=\frac{1}{2}a(t+t')^{2}+v_{l}(t+t')$ and at time $t_{4}$ we have $3L=\frac{1}{2}a(t+t'+t'')^{2}+v_{l}(t+t'+t'')$. Therefore $at^{2}+2v_{l}t=\frac{1}{2}a(t+t')^{2}+v_{l}(t+t')$ or $at^{2}+2aTt=\frac{1}{2}a(t+t')^{2}+aT(t+t')$. Solving for $T$ we get

$$T=\frac{t^{'2}+2tt'-t^{2}}{2(t-t')}$$

If we use $L$ and $3L$ we get instead $\frac{3}{2}at^{2}+3v_{l}t=\frac{1}{2}a(t+t'+t'')^{2}+v_{l}(t+t'+t'')$ or $3at^{2}+6aTt=a(t+t'+t'')^{2}+2aT(t+t'+t'')$ which leads to

$$T=\frac{(t+t'+t'')^{2}-3t^{2}}{2(2t-t'-t'')}$$

We could then equal these two expressions for $T$ and get some relation between $t,t',t''$. This seems right to me but is it?

 

[Philippe Verdy]

If acceleration "a" is contant, and each each car has length "L", then it's just enough to use t an t': the difference (t'-t) is proportional to the acceleration "a" and to "L" (and does not even depend on the initial speed of train when it starts going from the station)

We can also say (t''-t' =t'-t) if we assume a cartesian space-time (we ignore the effects of relativity contracting space "L" caused by acceleration where cars would possibly no longer have equal lengths): the last third car is not different from the second last car, we suppose the length of the train and each car is conserved (the train does not approaches the speed of light for the observer).

The two equations for T above should then be equivalent: just make the substitution (t''=2t'-t) implied by (t''-t' =t'-t) in the second formula, you should get the first one...

 

[Charles Link]

The unknowns are $L$, $a$ , and $v_l$, with $T=\frac{v_l}{a}$. Three equations and 3 unknowns. I think what you did on your first attempt was correct, but I need to check it.

 

[Philippe Verdy]

Note: we must also assume that there's no elastic deformation of cars (this elastic deformation exists for non relativist speeds, but for solid trains it should may be neglected... that's not really true in actual trains where elastic deformation really occurs at each attachment between each pair of cars, and is compensated by elastic springs, so not all cars start accelerating at the same time; the first car start moving when the last car is still steady for some measurable fraction of seconds, sometimes more for very long trains where we can really see the significant variation of total length during accelerations or brakes...)

The second equation gives T=(t'+t)/2 after the substitution.

So you can determine (a) directly from the average of (t') and (t), and from (L).

You don't need the third variable v_l, for the speed of the train when the last car exits the station, which only depends on the speed v_0 [of the front of the train when it leaves the station at time=0, but in your problem v_0=0] and from the impulsion (a), and (L) and the number of cars in the train.

Note that your solution based on the second does not depend at all on (t'').

You could also make the same substitution of variables in the 1st equation for T and get a solution that depends only on (t') and (t'') but not on (t).

Conclusion: you don't need three measurements of time, two of them are enough.

You may compare use the 3 measurements only to check that there was no elastic deformation of the train: if the two solutions gives different results, the difference is proportional to the elastic elongation of the train (not really caused by relativity, but by friction of car wheels on rails and by friction of wheels on their axis), which is more or less proportional to the acceleration (a) and to some mechnical properties of the building materials of cars used to resist this elongation.

 

[Charles Link]

@Jenny Physics I believe both of your answers are correct. For this problem, $t, t'$, and $t''$ are all related, but I don't think the simple relation given in post 2 is correct. To determine that relation, simply make both of your answers equal, (assuming all of your algebra is correct), and simplify the relation as much as possible.

 

[Philippe Verdy]

You can check by yourself: the substitution works on both formulas to give a solution to your problem, that does depends only on two time measurements (not three). The solution is necessarily the same if there's no elastic elongation of the train. And you've noted yourself that T=v_l/a:
We've got too many variables for a single solution of the non-elastic train: you only need to know t, t', and L to determine a and v_l, you don't even need to know how many cars were in the train.

If you need three times, you have a solution for an uniformly elastic train (assuming also that friction of wheels on rails or friction of wheels on their axis is uniform all along the train and the rails on which it runs), or possibly one of the cars from which you measured the passage duration was actually longer (not all cars are equal; but here also you can generally know the length of each car, by their known construction, or known location in the station when the train was parked there: these locations may be even marked on the waiting dock ground of the station even if the train is now gone; for trains like automatic metros, passenger cars usually are all with equal length, except the first and/or last car with engines, so that they align to the steps or protection doors that give access to the cars, and so that trains may have more or less cars depending on expected trafic of passengers)

 

[Philippe Verdy]

Also for trains that transport very heavy weights, the cars cannot be safely built to be completely rigid. They would not resist to elongation forces and would crack. Instead, the structure is built with an elastic floor supported by non-rigid, non-straight horitontal bars which are slightly curved. The charge is then hold on one or several several points fixed along the bars supporting also the rotating wheel axis, and within cabins of passenger cars, the floor is slightly elastic (with several gliding plates).

The structure of trains cannot be completely rigid (including side walls to resist lateral accelerations when trains take curves: the cabin is constantly changing form; rails are also slightly elastic and can be elongated and slide on the balast, otherwise they would also crack when trains are passing on them, and they must also adapt to changes of temperature caused by weather conditions).

The inelastic trains and railways do not really exist. Any attempt to rigidify them have failed catastrophically, just like the fact that they require regular maintenance to check for the existence of cracks: this is mandatory routine inspection for planes, but needed as well for trains and railways, because the elasticity of materials is not eternal and small cracks in the structure will fataly occur; but the construction may help maintaining a good enough elasticity with minimum damages. In trains the elastic springs connecting cars, and the elastic curved bars supporting cabins are standard ; just like the elastic construction of wings in planes, or the elastic suspensions in all transportation systems with wheels, and the elastic transmission of every motor "engines" -- including for animal traction, or transmission of human force by flexible chains on a bicycle, without which bicycles would be too risky for human bones (even if they are protected by elastic muscles and membranes whose "maintenance" is ensured by the renewal of new living cells to recycle the damaged cells, replace them and reglue them with elastic fluids, a natural process which is much longer in bones than in muscles and membranes, that cannot resist high pressures for long like what bones allow).

 

[gneill]

Also for trains that transport very heavy weights, the cars cannot be safely built to be completely rigid. They would not resist to elongation forces and would crack. Instead, the structure is built with an elastic floor supported by non-rigid, non-straight horitontal bars which are slightly curved. The charge is then hold on one or several several points fixed along the bars supporting also the rotating wheel axis, and within cabins of passenger cars, the floor is slightly elastic (with several gliding plates).

The structure of trains cannot be completely rigid (including side walls to resist lateral accelerations when trains take curves: the cabin is constantly changing form; rails are also slightly elastic and can be elongated and slide on the balast, otherwise they would also crack when trains are passing on them, and they must also adapt to changes of temperature caused by weather conditions).

The inelastic trains and railways do not really exist. Any attempt to rigidify them have failed catastrophically, just like the fact that they require regular maintenance to check for the existence of cracks: this is mandatory routine inspection for planes, but needed as well for trains and railways, because the elasticity of materials is not eternal and small cracks in the structure will fataly occur; but the construction may help maintaining a good enough elasticity with minimum damages. In trains the elastic springs connecting cars, and the elastic curved bars supporting cabins are standard ; just like the elastic construction of wings in planes, or the elastic suspensions in all transportation systems with wheels, and the elastic transmission of every motor "engines" -- including for animal traction, or transmission of human force by flexible chains on a bicycle, without which bicycles would be too risky for human bones (even if they are protected by elastic muscles and membranes whose "maintenance" is ensured by the renewal of new living cells to recycle the damaged cells, replace them and reglue them with elastic fluids, a natural process which is much longer in bones than in muscles and membranes, that cannot resist high pressures for long like what bones allow).

That's all very well and good, but does nothing to address the OP's puzzle. Please restrict your replies to homework help requests to matters that directly pertain the problem at hand and in the spirit in which it was framed. Relativity was not mentioned, strength of materials was not mentioned, neither was flexibility nor does manner of deformation of the train or tracks appear in the original problem statement.

If you wish to open a discussion about incidental matters that occur to you after reading a homework help request, the technical forums would be a better place to post. Members their would be more than happy to engage such topics.

 

[Philippe Verdy]

The comments was about the contest that claimed there was a need of 3 time variables in the problem.

I argue that only 2 are needed but only under some strict inelastic conditions that actually never exist in any real train.
At least 3 time variables are needed for the minimum elastic problem which is the real one but more variables may be needed if we want to be very precise (because elasticity is not uniform across actual materials, and not constant over time when materials are aging).

These are real physical contraints far from the "ideal" situation of the theoretical-only initial problem: we can use this simple solution however, if we don't seek very high precision and accept an actual margin of error.
The highest error will be most probably in the three measurements (t,t',t'') of time and in the knowledge of the exact value of (L).
You can minimize the errors if you have (t,t',t'') by solving the two equations for T using only the two largest times (t+t', t+t'+t''), if these measures were taken on the same clock with the same known absolute precision.

 

[PeroK]

The comments was about the contest that claimed there was a need of 3 time variables in the problem.

I argue that only 2 are needed but only under some strict inelastic conditions that actually never exist in any real train.
At least 3 time variables are needed for the minimum elastic problem which is the real one but more variables may be needed if we want to be very precise (because elasticity is not uniform across actual materials, and not constant over time when materials are aging).

These are real physical contraints far from the "ideal" situation of the theoretical-only initial problem: we can use this simple solution however, if we don't seek very high precision and accept an actual margin of error.
The highest error will be most probably in the three measurements (t,t',t'') of time and in the knowledge of the exact value of (L).
You can minimize the errors if you have (t,t',t'') by solving the two equations for T using only the two largest times (t+t', t+t'+t''), if these measures were taken on the same clock with the same known absolute precision.

That's all very well, but the assumption that the train has constant acceleration trumps all that!

 

[Philippe Verdy]

"constant acceleration of the train" ? So why I was conter-argued that we needed 3 time measurements? The experiment exposed in the problem is already testing the elastic model if it really needs the 3 time measurement variables (in which case there's NO constant acceleration of the **whole** train: the experiment effectively uses 3 distinct measurements on selected **parts** of the train, assuming already that acceleration may be variable). It's not clear what is "constant aceleration of the train", it may just mean the constant acceleration of the leading engined car...

 

[gneill]

We can also say (t''-t' =t'-t)

Let us make a concrete model to test this assertion. In our model the train is constructed from materials we store in the same box that we keep our physicists best stuff, like massless pulleys and light flexible strings. In other words, no flexing, stretching, or other shenanigans.

Each car has length L = 15 m. Acceleration is a constant a = 1 m/s². The passenger arrives when the train is already moving at v_o = 5 m/s.

We can calculate the speed of the train for each instant when a car has passed, and the duration of time that car took to pass:

Not the same.

 

[gneill]

We've got too many variables for a single solution of the non-elastic train: you only need to know t, t', and L to determine a and v_l, you don't even need to know how many cars were in the train.

We are not given L. It is an unknown constant.

 

[haruspex]

We can also say (t''-t' =t'-t)

Clearly not. Add more carriages. Asymptotically, the time per carriage tends to zero, so the differences will tend to zero.

the difference (t'-t) is proportional to the acceleration "a"

Did you mean inversely proportional to a? Even if so, that would require some proof. It is not evidently true.

 

[Philippe Verdy]

@haruspex, you're wrong on BOTH your claims.

The problem specifies "v_l" this limits the number of cars because "v_l" is already the speed reached at the station where the train started to go.
This implies a fixed number of cars which can "tend to infinity", so the time per carriage cannot tend to zero.

Suppose "v_l" is not known, then the number of cars must be known because the problem searches the time since when the train quitted the station, at the measurement location.

The difference of time between two cars really grows proportionaly to acceleration: if there was no acceleration at all (=0), this difference of times between carriages (t'-t) or (t''-t') between cars of equal length is also zero, so it can '''certainly not''' be inversely proportional to this null acceleration (otherwise this time difference would be... infinite !).

In fact such type of measurement is how we traditionally measure speeds, on naval ships when there's no other visible fixed point, by dragging a rope with regular "knots", the knots being like the points separating cars in a train, and consequently also accelerations by measuring time differences between equal number of knots (this measured speed however is relative to the speed and direction of water current and assumes this current is constant)

 

[Charles Link]

I agree with @haruspex on the first claim (post 14), and I mentioned this in post 5 above. And I also agree, as a couple of people have stated, that they only needed to give $t$ and $t'$, and that is sufficient to solve for the time that the passenger was late, as the OP correctly computed with the first answer they got for $T$.

 

[gneill]

The problem specifies "v_l" this limits the number of cars because "v_l" is already the speed reached at the station where the train started to go.
This implies a fixed number of cars which can "tend to infinity", so the time per carriage cannot tend to zero.

The idea is not to add cars to the front of the train, but to imagine cars following the "last" car in the problem. So the passenger arrives just as some car in a long string of cars is passing him. Clearly, as the number of cars passing at a constant acceleration grows, the velocity must grow and hence time to pass for each must diminish. The same must hold true for only three cars.

 

[Philippe Verdy]

I don't know where the formulas used by gneill to compute successive speeds come from "magically".

The problem speaks about a train where the passenger clearly knows the number of cars (he was arriving in the station where he expected to see the first car before it left the station: how does he know then he is counting the last cars if he does not know how the train is composed?

Yes there are is some implied variable not clearly stated about train composition (the fixed number N of cars in the train, or its total length NL), but also an additional "polluting" unneeded variable in the 3 measurements of time.

The measurement of times between cars and the knowledge of length L per car directly gives average speeds for the time each car passes in front of the traveler, like knots on a naval ship. It is the difference of these times that gives differences of speeds, which is proportional (not inversely proportional) to the "constant" acceleration, by definition of an acceleration.

These measurements are not *instantaneous* measurements of speeds and acceleration, but just averaged values between separate instants (the actual definition would require car lengths to tend to zero: this is no longer a train with cars of finite length, this is a single thread made with infinitely narrow cars, and the "3 last time measurements" would be all zero in that case).

Now comes the problem: the speed of train is also not specified: "He watches the last three cars of the train pass by." but we can assume the passenger was knowing how many cars there were in the train, and knew the length of each car, or that he knows the speed of the train when he arrives to the station.

Otherwise there's no solution at all to the problem if we have absolutely no knowledge at all for what is in front of the train when the passenger arrived to the station and no way to know the instantaneous speed of the train (which is why the passenger takes times measurements between the last 3 cars passing in front of him).

If the passenger assumes that the train is infinitely long and only sees the last 3 cars and knows absolutely nothing about the length of the train, this is non-sense. The passenger arrives in a station whose docking area has a known finite length were he could wait for the train before coming too late to take it. The total length of the train is necessarily not infinite, and must be known (otherwise it could not even park along the waiting dock of the station, these docks would also be need to be infinitely long, and the traveller would have no chance at all to take the train on time in the last car where he would have reserved a seat, he would always be too late to take it), otherwise this is not a real train station.

The problem states that the train contains more than 3 cars. The traveler coming to the station cannot walk indefinitely, the station is necessarily limited in total length, and this also limits the total length of the train.

So you can argue that the problem did not state all necessary variables, but still it exposes an unnecessary time variable. Something must be missing, the problem is not complete in the case we don't allow any assumption about:
- what is a traveler (a human that cannot walk at infinite speed to reach a specific car position in due time before the train starts going),
- what is a train (a non-infinite vehicle of finite length),
- what is a station (a non-infinite dock area where travelers can wait their train and walk to the right position to enter the correct car in which he will find his seat).

Let's assume that the position where the traveler arrived too late in the station was the correct position to enter the correct car in the train (so he would have not needed to walk along the dock if he was in time when the train arrived and was parked waiting for travelers to come inside), then we know that he's looking at the trail of the train and not interested at all in what was on the front side: we reduce the train only to these last 3 cars, the rest of the train does not matter (he does not need to know then how many cars have already passed in front of that position)

May be the train only had these 3 cars and there's no other cars in front, the traveler could not measure a non zero speed of the train because it has not already left the station so its speed must be zero, and the passenger is not too late to take his train, and this gives a contradiction. So the train necessarily has more than 3 cars (at least 4, but not an infinity: it is still limited by the total length of the station).

 

[haruspex]

The difference of time between two cars really grows proportionaly to acceleration:

Let's see...
Suppose one car takes Δt, the next takes Δt', and the speed at the boundary is v.
I get 2v(Δt-Δt')=a(Δt²+Δt'²).
Maybe you have some other formulation, using something other than v?

Edit: using L instead of v:
L(Δt-Δt')=aΔtΔt'(Δt+Δt')
So if we were to double a then the factor that must be applied to all the time deltas to get the same L is 1/√2.

 

[Philippe Verdy]

There's a way to represent the problem geometrically: if the acceleration of the train is constant, the graph of the position x of the start of the train according to time forms an vertical parabolic curve. The 3 measurements are made at regular intervals (of length L) along the vertical x axis, and indicate 4 positions on the parabolic arc made at regularly spaced vertical positions.

The traveller does not measure all points on the arc, only the 3 nodes on the parabolic arc, and its time measurements are vertical distance along the time axis between 4 instants. Basically the travelers only knows 3 chords of the parabolic arc, and wants to know the length to the origin of both axis where the parabolic arc was tangeant to the horizontal axis (speed=0 at x=0).

How many parobolic curves like this can exist that passes by the 4 points of the plane ? if the origin of x and y-axis are unknown (time measurements are indications of absolute time with an unknown origin at T horizontal distance of the instant he saw the first car passing), we know that the parabolic arc must have its focal axis vertical, this does not leave many choices: there's a single vertical parabole passing throught the four nodes which are equally spaced vertically and whose relative horizontal distances (measured time differences) is also determined by the problem.

This is a geometric problem, it's not possible to have multiple parabols. And in fact only 3 nodes are needed to define the parabole. A 4th one may give no solution at all.

 

[Charles Link]

There's a way to represent the problem geometrically: if the acceleration of the train is constant, the graph of the position x of the start of the train according to time forms an horizontal parabolic curve. The 3 measurements are made at regular intervals (of length L) along the x axis, and indicate 4 positions on the parabolic arc.
The traveler does not measure all points on the arc, only the 3 nodes on the parabolic arc, and its time measurements are vertical distance along the time axis between 4 instants. Basically the travelers only knows 3 chords of the parabolic arc, and wants to know the length to the point on the x-axis where the parabolic arc is vertical.

Your construction is accurate, and as I think we have all concluded, we only need two adjacent intervals, which comprise 3 points, to locate the origin of the parabola. The 3rd interval is extra info.

 

[Philippe Verdy]

Your construction is accurate, and as I think we have all concluded, we only need two adjacent intervals, which comprise 3 points, to locate the origin of the parabola. The 3rd interval is extra info.

It's extra info that allows checking the solution, or verifying that there was no elastic elongation or that all cars of the train have the same length L (if the train is inelastic)

So now assume we don't know L: it's the same as saying that the x vertical axis has no known unit. We can use any arbitrary unit because this only scales that axis and scaling vertically any vertical parabol still gives a parabol... L does not matter we can set to 1.
As well if we don't know the origin of time, we can fix it arbitrarily: this just slides horizontally the vertical parabol along the horizontal time axis, and this translation is still a parabolic arc. We can set the one instant arbitrarily at position t=0.
So we can finally position the 4 nodes on our arbitrarily chosen base. But at least the horizontal time axis has a known scale (in seconds).
Two chords are fully positioned on the resulting graph. The only unknown position is the position where the parabolic arc is tangeant to the horizontal time axis. But the parabolic arc is uniquely defined by 3 of the 4 nodes whose position are known on our arbitrarily chosen coordinate system. It is enough to determine T because the time axis has a known scale.

 

[gneill]

I don't know where the formulas used by gneill to compute successive speeds come from "magically".

Basic kinematic equations:
$v_f^2 - v_o^2 = 2 a d$
$v_f = v_o + a t$

The problem speaks about a train where the passenger clearly knows the number of cars (he was arriving in the station where he expected to see the first car before it left the station: how does he know then he is counting the last cars if he does not know how the train is composed?

In the problem as given he sees the last three cars with no other cars following. We are also told explicitly in the problem that they are the last three cars.

Yes there are is some implied variable not clearly stated about train composition (the fixed number N of cars in the train, or its total length NL), but also an additional "polluting" unneeded variable in the 3 measurements of time.

It would be nice to see your two-time solution in detail. Remember, the length of the train cars is not a given.

The measurement of times between cars and the knowledge of length L per car directly gives average speeds for the time each car passes in front of the traveler, like knots on a naval ship. It is the difference of these times that gives differences of speeds, which is proportional (not inversely proportional) to the "constant" acceleration, by definition of an acceleration.

We are NOT given L. So you need another variable to replace it.

These measurements are not *instantaneous* measurements of speeds and acceleration, but just averaged values between separate instants (the actual definition would require car lengths to tend to zero: this is no longer a train with cars of finite length, this is a single thread made with infinitely narrow cars, and the "3 last time measurements" would be all zero in that case).

The definitions of the values are clearly stated in the problem statement. In pure thought experiment such as this one we can assume that they are rigorously known givens.

Now comes the problem: the speed of train is also not specified: "He watches the last three cars of the train pass by." but we can assume the passenger was knowing how many cars there were in the train, and knew the length of each car, or that he knows the speed of the train when he arrives to the station.

No. While we as analysts may be mildly interested in such musings, all we're told is that the last three cars pass by the passenger. What he otherwise knows about the train (it's color maybe?) is irrelevant to the problem as stated.

Otherwise there's no solution at all to the problem if we have absolutely no knowledge at all for what is in front of the train when the passenger arrived to the station and no way to know the instantaneous speed of the train (which is why the passenger takes times measurements between the last 3 cars passing in front of him).

We know that the train has some speed as the cars are passing. What's in front of those three cars is irrelevant to the problem as given. You're free to use your imagination or to determine some realistic number cars that lay ahead of the last three. But that will not change the problem statement.

If the passenger assumes that the train is infinitely long and only sees the last 3 cars and knows absolutely nothing about the length of the train, this is non-sense.

Agreed. So why go there?

The passenger arrives in a station whose docking area has a known finite length were he could wait for the train before coming too late to take it. The total length of the train is necessarily not infinite, and must be known (otherwise it could not even park along the waiting dock of the station, these docks would also be need to be infinitely long, and the traveller would have no chance at all to take the train on time in the last car where he would have reserved a seat, he would always be too late to take it), otherwise this is not a real train station.

The problem states that the train contains more than 3 cars. The traveler coming to the station cannot walk indefinitely, the station is necessarily limited in total length, and this also limits the total length of the train.

In fact it's not a real train station. This is a math puzzle. All of this talk about infinities and docking space and walking times are utterly irrelevant to the puzzle as it is stated. It's just obfuscation to no good purpose.

Station length is irrelevant to the specific problem as it is spelled out. For our purposes the train might be exactly three cars long being pulled by a single engine.

So you can argue that the problem did not state all necessary variables, but still it exposes an unnecessary time variable. Something must be missing, the problem is not complete in the case we don't allow any assumption about:
- what is a traveler (a human that cannot walk at infinite speed to reach a specific car position in due time before the train starts going),
- what is a train (a non-infinite vehicle of finite length),
- what is a station (a non-infinite dock area where travelers can wait their train and walk to the right position to enter the correct car in which he will find his seat).

All irrelevant musings. We are told that he watches the last three cars go by. That is the only fact about his location and what happens that you can get from the problem statement. Everything else is irrelevant speculation. And we'd still like to see your algebraic two-time variable solution.

Let's assume that the position where the traveler arrived too late in the station was the correct position to enter the correct car in the train (so he would have not needed to walk along the dock if he was in time when the train arrived and was parked waiting for travelers to come inside), then we know that he's looking at the trail of the train and not interested at all in what was on the front side: we reduce the train only to these last 3 cars, the rest of the train does not matter (he does not need to know then how many cars have already passed in front of that position)

May be the train only had these 3 cars and there's no other cars in front, the traveler could not measure a non zero speed of the train because it has not already left the station so its speed must be zero, and the passenger is not too late to take his train, and this gives a contradiction. So the train necessarily has more than 3 cars (at least 4, but not an infinity: it is still limited by the total length of the station).

Three cars plus an engine would suffice.

 

[Charles Link]

@gneill I do like the latest input from @Philippe Verdy in post 20. It does suggest that you should be able to compute $a$ in terms of $L$, $t$, and $t'$, which I believe I just did, and also then determine how far away the head of the train is when the passenger arrived, which is $s=\frac{1}{2}aT^2$, in terms of $L$, $t$, and $t'$. $\\$ Some of his inputs, I think, were going off on a tangent, but post 20 looks like a very useful one.

 

[gneill]

@gneill I do like the latest input from @Philippe Verdy in post 20. It does suggest that you should be able to compute $a$ in terms of $L$, $t$, and $t'$, which I believe I just did, and also then determine how far away the head of the train is, which is $s=\frac{1}{2}aT^2$, in terms of $L$, $t$, and $t'$. $\\$ Some of his inputs, I think, were going off on a tangent, but post 20 looks like a very useful one.

I agree that the geometric approach is elegant and a worthy contribution to the thread. Unfortunately for the OP, the problem statement does not give us L.

 

[PeroK]

@gneill I do like the latest input from @Philippe Verdy in post 20. It does suggest that you should be able to compute $a$ in terms of $L$, $t$, and $t'$, which I believe I just did, and also then determine how far away the head of the train is when the passenger arrived, which is $s=\frac{1}{2}aT^2$, in terms of $L$, $t$, and $t'$. $\\$ Some of his inputs, I think, were going off on a tangent, but post 20 looks like a very useful one.

The timings depend on the ratio of $a/L$. If you assume the engine is the same length as a carriage, then from two timings you can calculate the number of carriages. And, from three timings, you can calculate the the length of the engine compared to the length of each carriage. It would be a bit messy though.

 

[Jenny Physics]

The timings depend on the ratio of $a/L$. If you assume the engine is the same length as a carriage, then from two timings you can calculate the number of carriages. And, from three timings, you can calculate the the length of the engine compared to the length of each carriage. It would be a bit messy though.

Interesting. How?

 

[Charles Link]

Interesting. How?

Here is my solution for $a$: $\\$ $L=v_o t+\frac{1}{2}at^2$ $\\$ $L=(v_o+at)t'+\frac{1}{2} at'^2=v_o t' +a(tt'+\frac{1}{2}t'^2)$ $\\$ Eliminate $v_o$ by miltiplying the first equation by $t'$ and the second by $t$ and subtracting. $\\$ The result is $a=\frac{(t-t')(2L)}{tt'(t+t')}$ if my algebra is correct.

 

[Philippe Verdy]

There's another difficulty in this problem: it's clear that even if we restrict the conditions to use only 2 times measurements, the solution for T is a zero of a quadratic equation.

Now let's project the origin of the parabol at time -T along the x axis: we get an x0 position which is NOT necessarily an integer multiple of L ! (To simplify thing, the x-axis should be scaled by dividing it by L: it counts the number of cars.

We know that the travelers arrives just to see the last 3 cars passing "completely" in front of him. This does not necessarily means that the traveler arrived at the station exactly at the time where the first of the last 3 cars was passing there, it may have been there between the two times the last 4 cars were starting to pass and the last 3 ones. but he must have waited a bit before seeing the exact moment where there remained exactly 3 cars passing.

If the resulting coordinate on the x-axis of the origin of the parabolic arc is not an integer, this means that the traveler was not at the correct position to take its train just before the train started to leave the station (he would have needed to walk a little along the waiting dock, by a distance between 0 and L) ! So the traveler was in fact a bit more late to take his train because he also needed to walk to the correct start position (at walking speed, not specified).

 

[Charles Link]

@Philippe Verdy We know that. You are making it overly complicated then. We need to assume he arrived just as he observed the edge of one car right in front of him. Otherwise, we have no way of computing anything precisely.

 

[PeroK]

Interesting. How?

The easy case, counting the engine as the first carriage (same length):

If the train starts from rest, the time $t_n$ for $n$ carriages to pass satisfies:

$nL = \frac12 a t_n^2$

The time for the n+1 st carriage to pass is then:

$T_{n+1} = t_{n+1} - t_n = \sqrt{\frac{2a}{L}}(\sqrt{n+1} - \sqrt{n})$

Hence you have:

$\frac{T_{n+2}}{T_{n+1}} = \frac{\sqrt{n+ 2} - \sqrt{n+1}}{\sqrt{n+1} - \sqrt{n}}$

That's going to be different for every $n$, so you just need to work out for which $n$ that equation holds.

Now, if there's an engine of length $K$ ...

 

[Charles Link]

The easy case, counting the engine as the first carriage (same length):

If the train starts from rest, the time $t_n$ for $n$ carriages to pass satisfies:

$nL = \frac12 a t_n^2$

The time for the n+1 st carriage to pass is then:

$T_{n+1} = t_{n+1} - t_n = \sqrt{\frac{2a}{L}}(\sqrt{n+1} - \sqrt{n})$

Hence you have:

$\frac{T_{n+2}}{T_{n+1}} = \frac{\sqrt{n+ 2} - \sqrt{n+1}}{\sqrt{n+1} - \sqrt{n}}$

That's going to be different for every $n$, so you just need to work out for which $n$ that equation holds.

Now, if there's an engine of length $K$ ...

@PeroK If you choose an $L$, e.g. $L=30$ m, (and tell us what it is), and choose your own $a$, e.g. $a=1$ m/sec^2, but don't tell us what it is, if you give us $t$ and $t'$ (in seconds) from your formula, (you are free to select $n$ but don't tell us what it is), I believe our calculations for $T$, $a$, and $s=\frac{1}{2}aT^2$ would allow us to readily compute both the value for $a$ as well as the number of cars in the train that had already passed as the passenger arrived. Such a test would basically be showing that our algebra is correct. $\\$ Edit: I tried it using $n=9$ (and 10, and 11), and $a=1$ and $L=30$, and it computed like it should.

 

[haruspex]

some relation between t,t′,t′′

(t'-t-t")³=t'³-t³-t"³?

 

[Philippe Verdy]

@Philippe Verdy We know that. You are making it overly complicated then. We need to assume he arrived just as he observed the edge of one car right in front of him. Otherwise, we have no way of computing anything precisely.

If you assume that, then there's no general solution with the rigid train !

I repeat what we know: we have a unique parabolic arc with vertical passing by three nodes in the cartesian plane, and the horizontal axis of distance is subvided at regular intervals of length L. The measured times are not constrained and can be any real value.

But then the extreme point of the unique ellipse passing by the three nodes is NOT (in the general case) located (on the distance axis of coordinates) at an exact multiple of L because that coordinate is a zero of a quadratic function. We then have a contradiction in the problem or we must seek other reasons:
- the clock is not exact but the error margins allows an imprecision for the determination of the position of the extreme point of the eliipse that includes a region of the 2D plane where it falls between two integer multiples of L.
- if the clock is precise enough and there's no integer multiple L for locating that point of the elliptic arc, the only solution is to admit elasticity. If we suppsoe that the train is rigid (when measured inside the train, then the elasticity is what the traveler observes outside the the train in the station: he must see the effect of relativity.
- now if the three mesures give 3 different locations for the extreme point of the ellipse, the elasticity is no longer an hypothese, it is the only solution and must be observed in the train as well as in the station, independantly of general relativity (whose we can predict the effect precisely).

The important point is that the solution cannot respect the condition that all cars must have the same length L, because it's impossible in the general case! Or the curve of movement is not elliptic, i.e. the acceleration "a" cannot be constant indefinitely !

And we know that acceleration cannot be constant because general relativity cannot support speeds higher than c, so the elliptic movement is certainly wrong (it is only possible as an approximation at low speeds): the two branches of the curve of positions are necessarily decelerating, because the derived curve of speeds is not a straight line, but is a sigmoid whose two branches will necessarily be converging to -c and +c. But, let's ignore the first branch which concerns the train before he arrived in the station there remains only the positive half-sigmoid branch for speeds ! The general relativity applies a negative acceleration on top of the positive acceleration ignited by the engines of the train.

And this is an interesting result, because the traveller with his own precise-enough clock can measure the effect of general relativity, just by observing the passing train (or equivalently by taking place in an elevator falling freely in front of stages of a building, and measuring the time when it passes in front of eah of them; here also we know that it cannot fall freely at constant acceleration, because the acceleration will be zero at the center of Earth and will become negative to continue "falling" to the other side of Earth; the elevator will reach its maximum speed which is about 28000 km/h in the middle of Earth after about 20 minutes and will then decelerate to come to the surface of at the antipode, where it will emerge and will not go above the initial height from where it was initially falling).

We can then observe general relativity on Earth by just looking at trains accelerating in front of us: this just requires a precise enough clock.

And on short distances in a laborary, we can also observe general relativity by measuring the time it takes for a small accelerated graduated wheel to measure interval of times where the regularly spaced graduations passes in front of a sensor: we should see significant differences depending only on the initial angular position of the wheel where it started rotating, and on the precision of the clock.

 

[gneill]

@Jenny Physics : Have you achieved in this thread, to your satisfaction, your goals in solving your mathematical puzzle?

 

[Jenny Physics]

@Jenny Physics : Have you achieved in this thread, to your satisfaction, your goals in solving your mathematical puzzle?

Yes thank you all. I learned quite a bit.

 

[gneill]

Yes thank you all. I learned quite a bit.

Thank you @Jenny Physics . I believe that this thread has served it's original purpose. If others would like to continue the discussion of issues, suppositions, or conundrums related to the premise of the original puzzle, I suggest that that discussion be taken to one of the technical forums. The homework question here has been resolved.

I am closing this thread as it has served its initial purpose.

If anyone feels that there is something substantive and relevant to add to the solution of the original problem as posed, please feel free to PM a mentor to request that your submission be added.