- #1
LonelyElectron
- 18
- 1
Hey all! I have three questions below and was hoping someone could proof read, and offer some guidance for the last one... Any help is greatly appreciated!
Question 1:
1. Homework Statement
Ryan needs to carry a bag of potatoes to the car. Determine how much work Ryan has to do to lift a 20 kg bag of potatoes to his shoulder, 1.7 m above the floor, and how much work he does as he carries this bag of potatoes to his car, which is parked 45 m away? Show your work.
F_g = mg
W = Fd

Question 2:
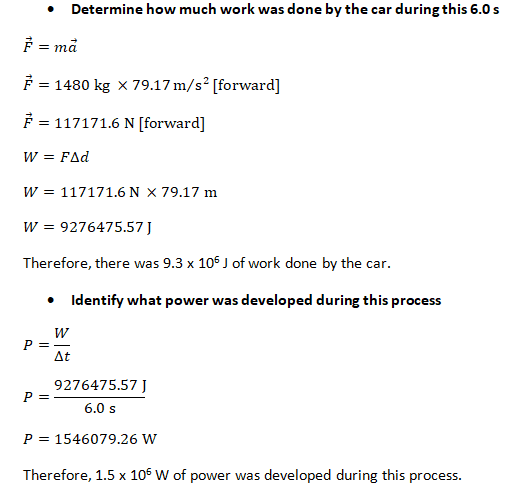
For a 1480 kg car that accelerates uniformly from a position of rest to a speed of 95 km/h in 6.0 s:
-calculate the car's acceleration
-determine how far the car traveled during this acceleration
-determine how much work was done by the car during this 6.0 s
-identify what power was developed during this process
F=ma
W=fd
v_f = v_i +at
d=1/2(v_i + v_f)t
P=W/t

Question 3:
Question 1:
1. Homework Statement
Ryan needs to carry a bag of potatoes to the car. Determine how much work Ryan has to do to lift a 20 kg bag of potatoes to his shoulder, 1.7 m above the floor, and how much work he does as he carries this bag of potatoes to his car, which is parked 45 m away? Show your work.
Homework Equations
F_g = mg
W = Fd
The Attempt at a Solution
Question 2:
Homework Statement
For a 1480 kg car that accelerates uniformly from a position of rest to a speed of 95 km/h in 6.0 s:
-calculate the car's acceleration
-determine how far the car traveled during this acceleration
-determine how much work was done by the car during this 6.0 s
-identify what power was developed during this process
Homework Equations
F=ma
W=fd
v_f = v_i +at
d=1/2(v_i + v_f)t
P=W/t
The Attempt at a Solution
Question 3:
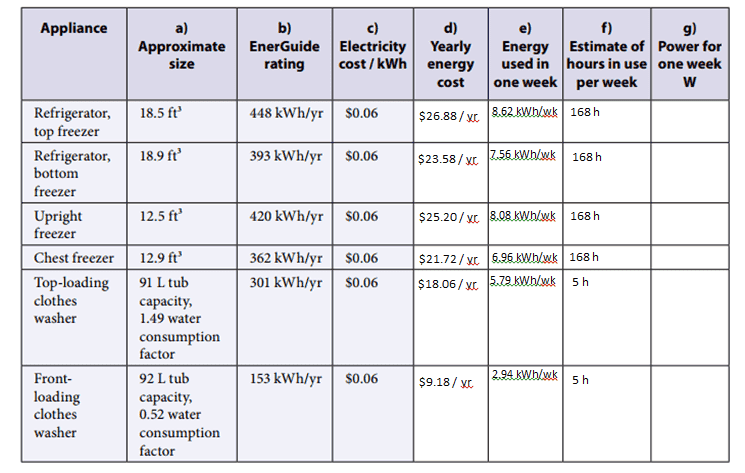
Homework Statement
Fill in the empty columns of the following table.
Homework Equations
No idea. I used common sense.
The Attempt at a Solution
very lost as to how I can fill out the last column...



