How to Determine Motor Torque for Preloaded Grinding Collar?

[Mech King]

Hi guys, as pert he attached file;

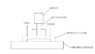
I have a motor rotates the shaft which is attached (keyed) to grinding collar. This grinding collar has a spring force which keeps it flush against the surface.

I’m trying to determine if I have enough torque from the motor to turn the preloaded grinding collar. Because the grinding collar is parallel with the force of gravity, there is not direct torque on the grinding collar, so how do I determine if the motor can turn the collar? I worked out the COF for the grinding faces, and multiplied this by the normal preload value (acting to compress the collar against the surface) in order to get my frictional force. The problem is, how can I translate this frictional force into a torque to see if it is less than the motor torque?

I can’t use the radial distance to the grinding surface of the collar acting on the ground, because this is not a torque as it is parallel with gravity.

Can anyone help clarify my thinking and approach to the problem?

Cheers

 

[CanisMajor]

if you have calculated your frictional resistance wrt area and inertial properties of the main disc these added will be equal to the resistive forces of the system... P = RV where p=power; r the resistive forces and v the velocity... if your motor summates to be greater than this, (either from a spec sheet or testing) then you should have enough power to drive the unit.

you will obviously have to have enough velocity to conquer the forces as frictional and inertial forces will not be constant during instantaneous engagement.