How to determine the angles using geometry (specific example)

[bolzano95]

Homework Statement

The following problem is taken from a physics problem, but I insulated the geometrical part for better understanding.

Relevant Equations

α=?
β=?

I'm trying to find angles α and β.

No additional information except: d, h, a.

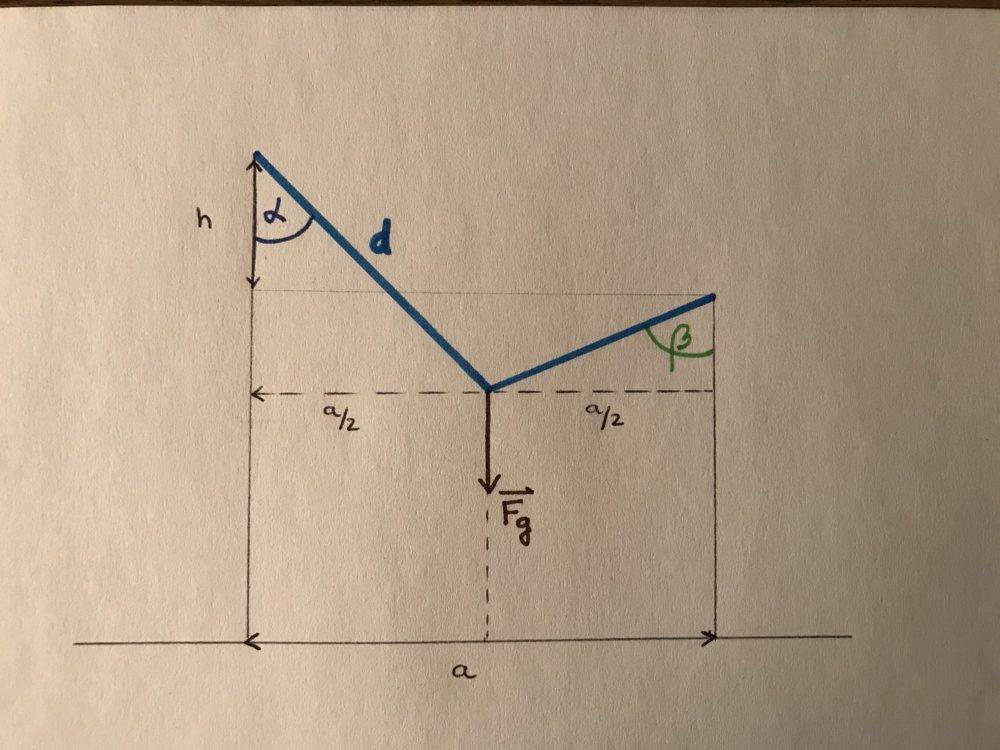
I already tried to figure it out by using isosceles triangles, but this is only true when there is a equilibrium of forces. I thought there are similar triangles incorporated, but I get too many unknown variables and not enough equations. I also tried using trapeziums but it doesn't pan out.

Maybe I overlooked something? Or is there a possibility that this problem is unsolvable?

 

[BvU]

What is d ?

 

[bolzano95]

What is d ?

d= complete length of a rope

 

[BvU]

At PF we don't like to give full soluitons, just ask guiding questions and give hints.
I like to think 'if you can draw it, you can calculate it'

Top left triangle is known: you have two sides and an angle (not $\alpha$). So you have third side. So you have two sides and an angle (not $\beta$) of the other triangle ... bingo !

 

[Orodruin]

but this is only true when there is a equilibrium of forces.

What is the full problem? Why are you assuming there is not equilibrium? This is the reason you should always post the entire problem statement without leaving things out.

 

[BvU]

In a math problem there are no forces.
[edit] missed the post that explains d.

Going to bed

 

[gneill]

Seems to me that you have two equations in two unknowns. If you can find the "heights" of the two triangles then you're golden: you'll be able to find the angles given two sides of each triangle.

You know that the sum of the two hypotenuses (hypoteni?) is d. You have a relationship between the height of the smaller triangle and the larger one. Two equations, two unknowns. Sweat the algebra and you should arrive at a result.

 

[haruspex]

Top left triangle is known

Not quite. h is the difference in heights of the two triangles.

@bolzano95 , you can find all the sides of the right hand triangle in terms of a and beta.
Combining these with h and d you can find all the sides of the left hand triangle.
What equations does that give you?

 

[BvU]

Not quite. h is the difference in heights of the two triangles.

As I posted, I missed the post that d is the full length of the blue line and thought it was the hypothenusa.

@bolzano95 : I still miss the full physics problem statement. What about the non equilibrium remark ?

 

[DEvens]

Recall your definitions of sin and cos.

Let d1 be the part of d on the left, d2 on the right. So d = d1+d2.

Then what is d1 sin alpha, d1 cos alpha, d2 sin beta, and d2 cos beta? What equations can you write relating these items?

 

[bolzano95]

gneill and BvU... I GOT IT! YES!

If I use a pythagorean theorem to express a "height" of the right hand triangle: $x^2= d^2_2-\frac{1}{4}a^2$ and then put it in a equation of the left hand triangle $(h+x)^2+\frac{1}{4}a^2=d^2_1=(d-d_2)^2$ I get $d_2$ and my life is solved! YES!

Orodruin, BvU: I thought about posting the whole problem on the forum, but I was only struggeling with geometric aspect. If you want (and insist :) I can post the whole problem here. But again, in my opinion the physics part is not vital for understanding with what I was having problem with. Let me know about the problem!