How to Determine the Stability Condition in Couette Flow?

[URIA]

Homework Statement

Hi all,
I would like to solve the attached .

Relevant Equations

Can someone help with the attached?

Dear All,
I tried to solve the attached question. it's about Couette flow, where the 2 plates move.

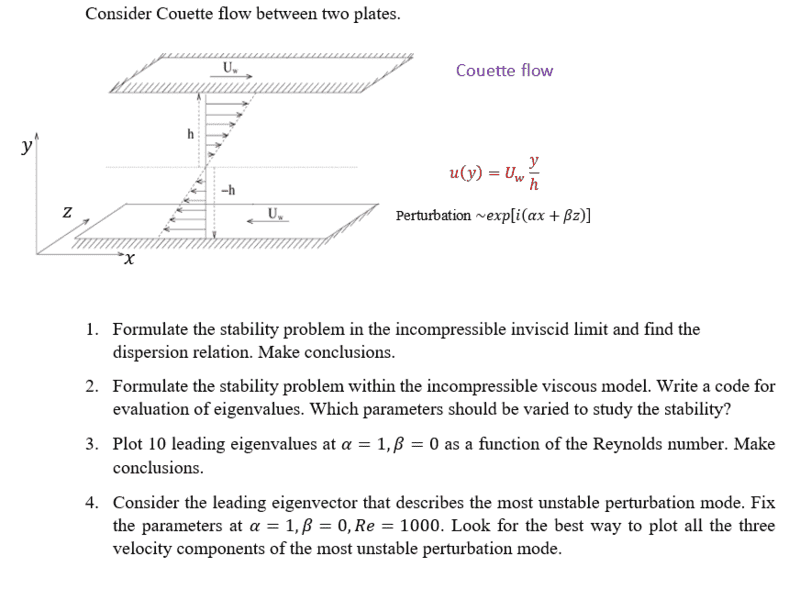
in fact, I have to find the stability condition. is someone familiar with this and can help?
many thanks,
uria

 

[vanhees71]

Do you have a general idea on how to do the stability analysis? Basically it's expanding around the un-perturbed given solution and linearizing the NS equations.

 

[URIA]

HI,
I assume I have to use the rayleigh equation because of the incompressible inviscid limit.