How to find the angle where the required pull is minimum?

[simphys]

Homework Statement

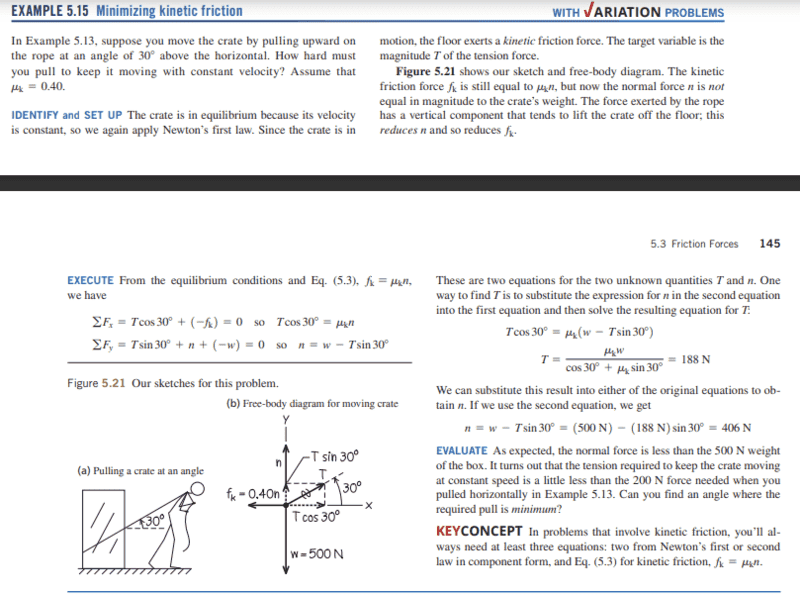
I have placed the problem as a picture. Just bevore 'Evaluate' it says find the angle where the required pull is minimum.

Relevant Equations

T = mu_k*w / ( cos(alpha) + mu_ksin(alpha) )

Can I get some help on how I'd do that?
I would parametrize the angle in the equation of where T = 188N and then take the derivative.
And then, what should I do then? it's not T' = 0 and I didn't have maxima minima vals in calc so yeah.
Thanks in advance.

 

[PeroK]

$T = 188N$ isn't relevant for the problem of minimising the required pull.

 

[kuruman]

The expression you have for T is a minimum when the denominator is at a maximum. How do you find the maximum of that? Take the derivative, set it equal to zero and solve for the angle.

 

[BvU]

Relevant Equations:: T = mu_k*w / ( cos(alpha) + mu_ksin(alpha) )

it's not T' = 0

Why not ? Plot $1/(\cos\alpha + 0.4 * \sin\alpha)$ to see there definitely is a minimum ...

$\$

 

[simphys]

$T = 188N$ isn't relevant for the problem of minimising the required pull.

it is isn't it? if I paramterize it by as a function of alpha ((nvm kuruman explained what you meant, it's only the denominator)

 

[BvU]

The 188 you mention is a function of $\alpha$

 

[simphys]

The expression you have for T is a minimum when the denominator is at a maximum. How do you find the maximum of that? Take the derivative, set it equal to zero and solve for the angle.

oooh thanks, okay! makes total sense...

 

[BvU]

Your relevant equation is the parametrization $T(\alpha)$

 

[simphys]

The 188 you mention is a function of $\alpha$

yes I should just take the derivative of the denominator instead of T itself as i did.

 

[BvU]

Can do that - but maximizing the denominator is less work

 

[simphys]

Can do that - but maximizing the denominator is less work

what do you mean if I may ask?
taking the derivative of the denominator and setting it equal to zero, isn't that maximizing?

 

[simphys]

Can do that - but maximizing the denominator is less work

Derivative is -sinx + mu_k*cos(x) = 0
Can't really find a way to solve this to be honest.

 

[BvU]

Haha, how to solve $\mu\cos \alpha-\sin\alpha = 0 \ \$

Spoiler

via
$\ sin(\beta-\alpha) = 0$

 

[simphys]

lol I am dying
I literally was summing up all those formulas besides the sum formulas

 

[simphys]

Haha, how to solve $\mu\cos \alpha-\sin\alpha = 0 \ \$

Spoiler

via
$\ sin(\beta-\alpha) = 0$

Thanks for the help by the way

 

[simphys]

Haha, how to solve μcos⁡α−sin⁡α=0

Spoiler

via
sin(β−α)=0

@BvU you know what works... just dividing by cos alpha and then we get alpha = arctand(mu_k) loll

 

[PeroK]

Derivative is -sinx + mu_k*cos(x) = 0
Can't really find a way to solve this to be honest.

What have you tried?

 

[simphys]

What have you tried?

of course :) ( oh apologies I didn't see the 'what')

 

[simphys]

What have you tried?

but I got it in the end by doing a very veryyy simple thing...

 

[simphys]

What have you tried?

acosx - sin x = 0
acosx/cosx - tanx = 0/cosx
a = tan x
x = arctanx

(didn't see the what, tried with double angle formulas,...) but I don't know why I didn't think of this at first...

 

[PeroK]

One thing I would recommend is putting these problems on a spreadsheet. In this case, you can have each variable: $m$, $\mu_k$, $g$, $\theta$, $T$. From this you can calculate the normal force $F_N = mg - T\sin \theta$, the friction force $F_f= \mu_k N$, the accelerating force $F \cos \theta$ and the acceleration (if $F \ge F_f$). Then you can play about by, say, fixing $\theta$ and varying $T$; or, fixing $T$ and varying $\theta$.

There are several benefits of having a numerical model of these problems. Not least it helps you gain an understanding of what is happening physically.

 

[simphys]

One thing I would recommend is putting these problems on a spreadsheet. In this case, you can have each variable: $m$, $\mu_k$, $g$, $\theta$, $T$. From this you can calculate the normal force $F_N = mg - T\sin \theta$, the friction force $F_f= \mu_k N$, the accelerating force $F \cos \theta$ and the acceleration (if $F \ge F_f$). Then you can play about by, say, fixing $\theta$ and varying $T$; or, fixing $T$ and varying $\theta$.

There are several benefits of having a numerical model of these problems. Not least it helps you gain an understanding of what is happening physically.

wooww.. Thanks for this amazing idea.
That's 100% facts. When I need to derive a general formula (which I needed to do in the successive example) I just reason about it in my head but when you actually have numericals it will be a 100 % more beneficial.

(and by the way: on which ones 'd you recommend to do it, on general problems only perhaps that are of the kind that are not really 'directly' derived and depend on a couple of values like $m$, $/mu_k$, $g$, $/theta$ ,...)

 

[PeroK]

wooww.. Thanks for this amazing idea.
That's 100% facts. When I need to derive a general formula (which I needed to do in the successive example) I just reason about it in my head but when you actually have numericals it will be a 100 % more beneficial.

(and by the way: on which ones 'd you recommend to do it, on general problems only perhaps that are of the kind that are not really 'directly' derived and depend on a couple of values like $m$, $/mu_k$, $g$, $/theta$ ,...)

I use spreadsheets generally in preference to a calculator. There are several uses: getting a grasp of the problem; calculating a numerical answer; sanity checking a formulaic answer; ... Whenever numbers help.

 

[BvU]

@BvU you know what works... just dividing by cos alpha and then we get alpha = arctand(mu_k) loll

I have to admit that is considerably quicker

But it's still a good addition to one's toolkit to know how to solve $\ a\cos\alpha + b\sin\alpha = c \$

$\$

 

[PeroK]

Another addition to the toolkit:

The angle that minimises $T$ is defined by $\tan \theta_0 = \mu$, and the minimum $T$ is:
$$T_0 = \frac{mg\mu}{\cos \theta_0 + \mu \sin \theta_0} = \frac{mg\tan \theta_0}{\cos \theta_0 + \tan \theta_0 \sin \theta_0} = mg\sin \theta_0$$Also:
$$\tan \theta_0 = \mu \ \Rightarrow \ \sin \theta_0 = \frac{\mu}{\sqrt{1 + \mu^2}}, \ \cos \theta_0 = \frac{1}{\sqrt{1 + \mu^2}}$$Therefore:$$T_0 = \frac{mg\mu}{\sqrt{1 + \mu^2}}$$For $\mu = 0.4$ the man could have saved himself $2N$.

 

[kuruman]

There are several benefits of having a numerical model of these problems. Not least it helps you gain an understanding of what is happening physically.

I always advised my students to use a spreadsheet instead of a calculator. From the standpoint of the problem author, I used a spreadsheet exclusively when designing multiple choice questions with numerical answers. It allowed me to craft the most likely "wrong" answers given the input parameters by simply altering this or that in the cell containing the right formula. It also enabled me to easily produce three different versions of the same test, given in a crowded lecture hall, with identical problems but different input numbers. A discreet marking on the front page identified the version. The copies were distributed so that each student was flanked on either side by a different version. Of course, the students were advised that, if their eyes "wander during the test and happen to see that their neighbor's answer does not match theirs, not to worry; they could both be correct." Students who put down answers belonging to the wrong version were referred to the university's Academic Honesty Committee for a disciplinary hearing and adjudication.