simphys
- 327
- 46
- Homework Statement
- see picture
- Relevant Equations
- M = r X F
Hello, so I have a question about the sense of rotation of the body.
I get the calculating part nd stuff like that. But what I don't understand is how we would determine the sense of rotation about the moment axis?
Could someone explain this to me please? (to add to this, I know that it is determined by the right-hand rule, but how do we do that for the resultant moment?
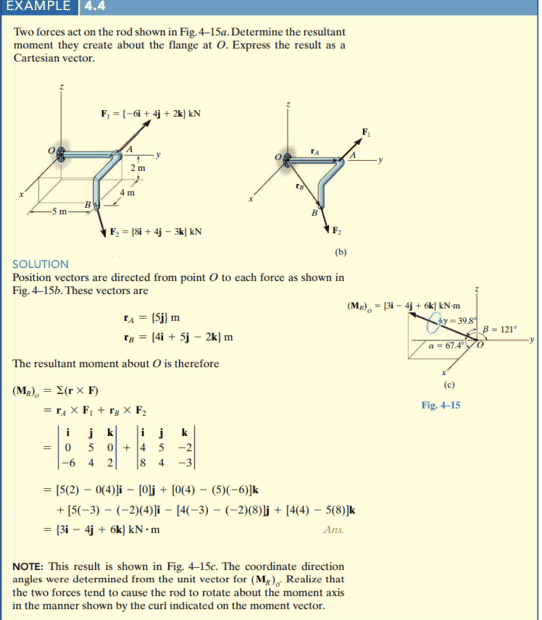
I get the calculating part nd stuff like that. But what I don't understand is how we would determine the sense of rotation about the moment axis?
Could someone explain this to me please? (to add to this, I know that it is determined by the right-hand rule, but how do we do that for the resultant moment?