How to form the stress tensor component from the equilibrium equation?

[lachgar]

Homework Statement

An elastic homogene and isotrope cuboid that is under a constant pressure (-p) in its 4 lateral surfaces. We neglect the weight .

Relevant Equations

equilibre equation, bondary conditions, s

Good evening everybody.
This is my suggestion for answer.
The tensor is diagonal and the compression is a plane stress

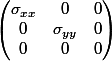
equilibre equation div(σ)=0
so:

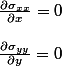
So, does that means that

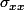= f(y.z) = Ay+Bz and

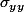=f(x.z)= Cx+Dz
A,B,C and D are constants.
Is that what the question meant?
Thank you in advance.

 

[Orodruin]

What are the boundary conditions?

 

[lachgar]

Hi. Thanks for replying.
As shown in the picture, all lateral surfaces are under a constant pressure.
There is no stress on the superior S(z=h/2) and inferior S(z=-h/2) surfaces. (h is the thickness).
The red arrow represent the stress vector, and the black one represent the unit normal vector of the surface.

Thank you.

 

[Orodruin]

So, to repeat my question, what does this imply for the boundary conditions?

 

[lachgar]

The bondary conditions are:

T(e_x)=-p e_x
T(-e_x)=p e_x
T(e_y) =-p e_y
T(-e_y) = p e_y
T is the stress vector.

 

[Orodruin]

So how does this relate to your solution?

 

[lachgar]

The question was posed as:
-Using the equilibre equations, give the form of

and

?

 

[Orodruin]

Yes, and you started this thread by writing down differential equations for them. To solve differential equations you need boundary conditions.

 

[lachgar]

it's not demanded to solve it, it's bout giving the general form

So,can we just say that

= f(y.z) = Ay+Bz

How can I be sure that it's a linear form, I mean we only know that it depends on y and z.

 

[lachgar]

Thank you