- #1
VinnyCee
- 489
- 0
Using superposition, find [itex]v_0[/itex] in the following circuit.
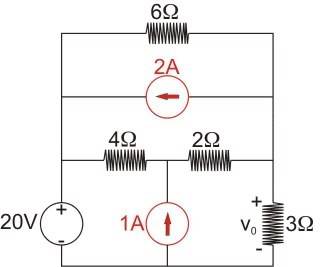
My work so far:
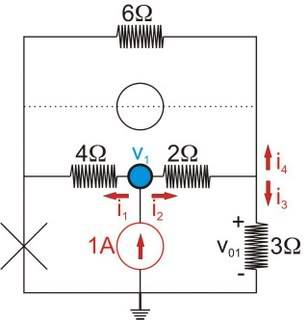
[tex]v_1\,=\,2\,i_2\,+\,v_{0\,1}[/tex]
[tex]v_1\,=\,4\,i_1[/tex]
[tex]v_{0\,1}\,=\,3\,i_3[/tex]
[tex]v_{0\,1}\,=\,6\,i_4[/tex]
KCL @ v1:
[tex]1\,A\,=\,i_1\,+\,i_2[/tex]
[tex]i_2\,=\,i_3\,+\,i_4[/tex]
Using these six equations, with 6 variables:
[tex]v_{0\,1}\,=\,\frac{12}{7}\,V[/tex]
Is that correct?
My real question is how to put the six equations above into matrix form to enable solving using RREF.
Thanks
EDIT: I have fixed this part with Paallikko's guidance (thanks!)
[tex]i_1\,=\,\frac{1}{4}\,v_1[/tex]
[tex]i_2\,=\,\frac{1}{2}\,v_1\,-\,\frac{1}{2}\,v_{01}[/tex]
[tex]i_3\,=\,\frac{1}{3}\,v_{01}[/tex]
[tex]i_4\,=\,\frac{1}{6}\,v_{01}[/tex]
[tex]i_1\,+\,i_2\,=\,1\,A\,\,\,\Rightarrow\,\,\,\frac{3}{4}\,v_1\,-\,\frac{1}{2}\,v_{01}\,=\,1[/tex]
[tex]i_3\,+\,i_4\,=\,i_2\,\,\,\Rightarrow\,\,\,\frac{1}{3}\,v_{01}\,+\,\frac{1}{6}\,v_{01}\,=\,\frac{1}{2}\,v_1\,-\,\frac{1}{2}\,v_{01}[/tex]
[tex]v_{01}\,=\,1\,V[/tex]
My work so far:
[tex]v_1\,=\,2\,i_2\,+\,v_{0\,1}[/tex]
[tex]v_1\,=\,4\,i_1[/tex]
[tex]v_{0\,1}\,=\,3\,i_3[/tex]
[tex]v_{0\,1}\,=\,6\,i_4[/tex]
KCL @ v1:
[tex]1\,A\,=\,i_1\,+\,i_2[/tex]
[tex]i_2\,=\,i_3\,+\,i_4[/tex]
Using these six equations, with 6 variables:
[tex]v_{0\,1}\,=\,\frac{12}{7}\,V[/tex]
Is that correct?
My real question is how to put the six equations above into matrix form to enable solving using RREF.
Thanks
EDIT: I have fixed this part with Paallikko's guidance (thanks!)
[tex]i_1\,=\,\frac{1}{4}\,v_1[/tex]
[tex]i_2\,=\,\frac{1}{2}\,v_1\,-\,\frac{1}{2}\,v_{01}[/tex]
[tex]i_3\,=\,\frac{1}{3}\,v_{01}[/tex]
[tex]i_4\,=\,\frac{1}{6}\,v_{01}[/tex]
[tex]i_1\,+\,i_2\,=\,1\,A\,\,\,\Rightarrow\,\,\,\frac{3}{4}\,v_1\,-\,\frac{1}{2}\,v_{01}\,=\,1[/tex]
[tex]i_3\,+\,i_4\,=\,i_2\,\,\,\Rightarrow\,\,\,\frac{1}{3}\,v_{01}\,+\,\frac{1}{6}\,v_{01}\,=\,\frac{1}{2}\,v_1\,-\,\frac{1}{2}\,v_{01}[/tex]
[tex]v_{01}\,=\,1\,V[/tex]
Last edited: