How to interpret the center of aero forces on a sports car?

[Rok]

Homework Statement

car simulation

Relevant Equations

none

Hello. I am working on a physics project for a simulation title and have stumbled upon on an interesting challenge.

Below is the example from wind tunnel data of a Dodge Viper GTS sports car.

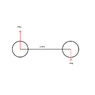
Wheelbase: 2,44m
Lift front axle: 54kg
Negative lift rear axle: 26kg

Can somebody please explain to me how to:

1. Determine the sum of two opposing forces acting on front and rear axles? I don't think that simply substracting the two values is the correct method here as one must take into account car balance also. For example if you had equal amount of forces acting in opposite directions like 50kg lift at the front, -50kg rear negative lift car would have a large understeer so the sum of forces cannot be 0.
2. Where is the center of those forces relative to the wheelbase?

 

[jack action]

so the sum of forces cannot be 0.

Yes, it is. But the sum of moments is not zero.

Where is the center of those forces relative to the wheelbase?

How would you do it if the forces were called front & rear weights? Where would be the center of gravity for the total weight?

 

[Rok]

If both forces acted in the same direction I would add 54kg + 26kg = 80kg
Then 54kg/80kg = 68% front aero balance. Then multiply wheelbase with 0,68.
0,68 x 2,44m = 1,66m meaning center of forces being this distance away from rear axle.

But I don't know how to calculate if forces are pointed opposite of each other. Obviously aero center will shift? Note those are not static weights. They represent aerodynamic vertical loads.

 

[haruspex]

If both forces acted in the same direction I would add 54kg + 26kg = 80kg
Then 54kg/80kg = 68% front aero balance. Then multiply wheelbase with 0,68.
0,68 x 2,44m = 1,66m meaning center of forces being this distance away from rear axle.

But I don't know how to calculate if forces are pointed opposite of each other. Obviously aero center will shift? Note those are not static weights. They represent aerodynamic vertical loads.

To represent the pair of forces completely by a single force, it must be equal to the sum of the forces (54-26=28 kg wt upward in this case) and exert the same moment about any given point.
For simplicity, take a moment axis in the line of action of one of the forces, the 26kg wt force, say. The 54 kg wt acts upwards a distance 2.44m away. If the net force acts upwards a distance x away we have (54)(2.44m)=(28)x.

 

[jack action]

If both forces acted in the same direction I would add 54kg + 26kg = 80kg
Then 54kg/80kg = 68% front aero balance. Then multiply wheelbase with 0,68.
0,68 x 2,44m = 1,66m meaning center of forces being this distance away from rear axle.

Taking direction into consideration, if we assume downward is positive, then upward is negative (or vice versa). Try the same thing again with that in mind.

 

[Rok]

OK. So you are saying 54kg-26kg = 28kg
Then 54kg/28kg = 1,93 or 193% of the wheelbase. The result being 4,709m to the left of the right axle.
So a force equal of 28kg is pressing down 4,709m to the left of the right axle. Is this correct?

 

[haruspex]

OK. So you are saying 54kg-26kg = 28kg
Then 54kg/28kg = 1,93 or 193% of the wheelbase. The result being 4,709m to the left of the right axle.
So a force equal of 28kg is pressing down 4,709m to the left of the right axle. Is this correct?

As I wrote, the net force is upwards. Other than that, yes.
Or do you mean the force required to balance the forces shown?

 

[Rok]

Yes I meant to wrote up. OK. So if we take this method of calculating a force there is one problem. What happens if there was equal amount of forces acting in opposite directions like 50kg lift at the front, -50kg rear negative lift a car would have a large understeer in reality. But in a simulation the net force would be 0. So in this case its not possible to simulate proper aero balance of two forces with a single force?

 

[Delta2]

For simplicity, take a moment axis in the line of action of one of the forces, the 26kg wt force, say. The 54 kg wt acts upwards a distance 2.44m away. If the net force acts upwards a distance x away we have (54)(2.44m)=(28)x.

I disagree here, that's not the correct way to find the point where the net force acts. The point where the net force acts splits the distance 2.44 in two parts x and y such that $$\frac{x}{y}=\frac{54}{26}$$ $$x+y=2.44$$.

EDIT:NVM I found out its the same thing...

 

[haruspex]

Yes I meant to wrote up. OK. So if we take this method of calculating a force there is one problem. What happens if there was equal amount of forces acting in opposite directions like 50kg lift at the front, -50kg rear negative lift a car would have a large understeer in reality. But in a simulation the net force would be 0. So in this case its not possible to simulate proper aero balance of two forces with a single force?

When the forces are equal and opposite, but not in the same line, they do not reduce to a single force. Instead, they reduce to a pure torque, known technically as a 'screw'.

 

[jack action]

Yes I meant to wrote up. OK. So if we take this method of calculating a force there is one problem. What happens if there was equal amount of forces acting in opposite directions like 50kg lift at the front, -50kg rear negative lift a car would have a large understeer in reality. But in a simulation the net force would be 0. So in this case its not possible to simulate proper aero balance of two forces with a single force?

If you try with small differences (51/50; 50.1/50; 50.01/50; etc.), you'll notice that as the difference tends to zero, the distance from the axle tends to infinity. But at a difference of zero, you get a moment defined by $0 \times \infty$ which - in this case - gives us zero (no force, thus no moment), which brings us back to @haruspex 's comment in post #10.

 

[haruspex]

at a difference of zero, you get a moment defined by 0×∞ which - in this case - gives us zero (no force, thus no moment)

I am not sure what you intend here. It sounds like you are keeping the offset between the two lines of action constant while reducing the difference in magnitudes to zero.
Why would that lead to 0×∞? The lever arm is the distance between the lines of action. Besides, 0×∞ is simply indeterminate. The moment certainly does not become zero, even though the net force does.

 

[jack action]

Why would that lead to 0×∞?

As the sum of the forces tends to zero, the equivalent lever arm tends to $\infty$. The moment is one multiply by the other.

Besides, 0×∞ is simply indeterminate.

In this case - and this case only - if the sum of forces is exactly 0, then I assume that no matter what the lever arm's length is, the resulting moment is also zero. But I'm open to other points of view here.

My goal was just to point out that, in a simulation, you might be able to always assume a small difference when the sum of forces went to zero and it wouldn't break your mathematical model.

 

[haruspex]

As the sum of the forces tends to zero, the equivalent lever arm tends to ∞. The moment is one multiply by the other.

As I wrote in post #12, it is not clear to me what set up you were discussing in post #11. You were replying to post #8, in which the force magnitudes approached equality while the distance between their lines of action remained fixed. In that case the lever arm is constant. Why do you say it tends to infinity?

 

[jack action]

You said earlier:

If the net force acts upwards a distance x away we have (54)(2.44m)=(28)x.

If you replace 54 and -26 with 50 and -50 you should rewrite this equation to (50)(2.44m)=(0)x. Then x=$\infty$, leading to $0 \times \infty$. Writing this, I realize that I was wrong in saying the moment is zero since it is clearly equal to (54)(2.44m).

 

[haruspex]

You said earlier:

If you replace 54 and -26 with 50 and -50 you should rewrite this equation to (50)(2.44m)=(0)x. Then x=$\infty$, leading to $0 \times \infty$. Writing this, I realize that I was wrong in saying the moment is zero since it is clearly equal to (54)(2.44m).

Ah, you are discussing the moment arm of the resultant force, not the moment arm of the applied forces. Now I understand. Yes, a pure torque is like an infinitesimal force applied at infinity, but expressing it as such renders its magnitude indeterminate.

 

[Rok]

I am bumping this thread to follow up the discussion with a different question. So we have determined center of horizontal forces but what about vertical center? Look at the picture below. The start of the long horizontal line represents center of horizontal aero forces. How does one then determine vertical center from this position? Am I correct that if you would have horizontal center for example somewhere between wheelbase then the center of vertical forces would be at a half point between floor and roof based on that horizontal center? What happens then if you had calculated position which is outside of car body like here?

 

[haruspex]

The start of the long horizontal line

By that do you mean the left end of the line labelled Y?
Why "start"? The centre of the horizontal aero forces is itself a horizontal line indicating a vertical position.
But that makes this meaningless:

if you would have horizontal center for example somewhere between wheelbase

the center of vertical forces would be at a half point between floor and roof

First, which vertical forces are we discussing? If you mean the resultant of all of them then that would be a pure torque. It would have no "centre", horizontal or vertical.
If you mean all except gravity, say, then its centre is a vertical line at some horizontal position.
Since the centre of the aero forces is above ground, and these would be balanced, more or less, by the friction from the road, the horizontal forces produce a net torque tending to lift the leading end of the vehicle. To balance that, the normal force from the ground would be behind the mass centre. The higher or stronger the aero forces, the further back the normal force. If the normal force needs to be behind the rear wheels the car flips over backwards.

 

[Rok]

No I mean the start of the longest line below the car from the left. We are discussing center of aerodynamic forces or aerodynamic pressure. It will be expressed as a position in meters from the rear axle. As you can see I already have a position for a horizontal axis, but don't know how to set position for the vertical axis.

 

[jack action]

The start of the long horizontal line represents center of horizontal aero forces.

Which "horizontal aero forces" are you referring to? Because the OP is asking about finding the center of vertical forces:

Lift front axle: 54kg
Negative lift rear axle: 26kg

[...]

2. Where is the center of those forces relative to the wheelbase?

 

[haruspex]

No I mean the start of the longest line below the car from the left. We are discussing center of aerodynamic forces or aerodynamic pressure. It will be expressed as a position in meters from the rear axle. As you can see I already have a position for a horizontal axis, but don't know how to set position for the vertical axis.

I find your use of the terms horizontal and vertical "axes" ambiguous. The horizontal component of the forces acts at a certain height. So it acts along a horizontal line (is that what you mean by horizontal axis?) and has a vertical coordinate (i.e. a position on the vertical axis).

The point at the left end of the line below the car cannot be either. The horizontal component must act somewhere above the ground and below the roof, while the vertical component must act between the nose and tail.
See the diagrams at http://theracingline.net/2018/race-...ic-efficiency-balance-and-center-of-pressure/ and http://www.formula1-dictionary.net/aerodynamic_balance.html.
According to http://www.landracing.com/docs/CG-CP_Hakansson-Dube_4-2014.pdf, the line of action of the vertical component can be approximated by treating the vehicle as a solid of uniform density (of the same exterior shape) and finding the CG of that.

 

[Rok]

The horizontal component of the forces acts at a certain height. So it acts along a horizontal line (is that what you mean by horizontal axis?) and has a vertical coordinate (i.e. a position on the vertical axis).

Yes. What I meant to say is that the long horizontal line represents aero torque measured from the rear axle. The start of the long horizontal line represents center of vertical aero forces obviously. It doesn't matter that it is outside the car body dimensions. It was calculated from the above data for vertical loads and we had concluded that this is correct. So what I am asking here is at what height measured from the rear axle should this line be then? (I used term vertical center). Sorry for the confusion.

 

[haruspex]

at what height measured from the rear axle should this line be then? (I used term vertical center).

If the purpose of the line is merely to represent where the effective vertical force is it has no specific height. It is merely a horizontal distance from some reference point, such as the rear axle.

If you know the drag force then we can bring that in. In order to preserve the torque balance you already have, we can take the drag as though acting at ground level and add that to the vertical force already established. This gives you a force acting at, say, the point on the ground at the left end of the line and angling back over the top of the car. But you can then take this force as acting at any point along that sloping line. There is no specific point on that line which is the point at which the force acts.

 

[Rok]

I know the drag force is 1108N (113kg). But if I understand you correctly center of vertical forces has no effect on center of horizontal forces? Now that you have net vertical force and drag how do you calculate this? Isn't horizontal forces center just a halfway distance between floor and roof then?

 

[haruspex]

center of vertical forces has no effect on center of horizontal forces?

It is unhelpful to think in terms of a centre of horizontal force and a centre of vertical force. There are only three scalars needed to specify the net aerodynamic force, but many choices of what three parameters to use.

The net force has a magnitude, a direction and a line of action. In your experiment you found the changes the wind made to the normal forces from the ground, and you considered these as resulting from a force acting at a point on the ground at some displacement X in front of the rear wheel. This allowed you to write equations $F_y=\Delta N_l+\Delta N_r$, $F_yX=\Delta N_lL$, where L is the wheelbase. So you found everything except $F_x$ (which you could in principle have found from the change in the frictional forces on the ground).
Having done that, there is no "centre of horizontal force" to be found. You have taken the point of action as being on the ground, displacement X.

Suppose now that you have measured $F_y$. I'll treat the point of contact of the rear wheel as the origin, so the X displacement is technically at (-X,0).
You could now consider the aero force to act at some height Y above the rear wheel. You can find Y from the torque equation $F_xY=\Delta N_lL$. All this means is that the line of action of the aero force runs through the points (-X,0) and (0,Y). There is not a separate displacement of horizontal force and vertical force, they are just two ways of representing the same line of action.

 

[jack action]

Isn't horizontal forces center just a halfway distance between floor and roof then?

It will depend greatly on the frontal area geometry. It should be close to the geometric center (see second moment of area).

I have never seen this calculated per se, as for typical vehicles, drag is always assumed to be acting at the center of gravity's height.

If you look at sideway stability, the shape of the side view area is of more importance because of the lack of symmetry. Taking as an example the shark fin on a Formula One:

You can intuitively see that a sidewind would act more at the rear of the car than at the front. A sidewind will always tend to push the car out of its track. But pushing more at the rear will tend to pivot the car about the front axle and creates a steering effect towards the wind. The more the sidewind pushes on the car, the more the car steers against it, thus the car is self-correcting without any driver input.

If the sidewind would act more at the front end, the opposite would occur, namely the more the sidewind pushes, the more the car would steer in the direction it is pushed, leading to instability and requiring the driver to constantly adjust the steering input according to the sidewind force.