- #1
cloud360
- 212
- 0
?How to know if equilibrium points are stable or not. Is my solution correct
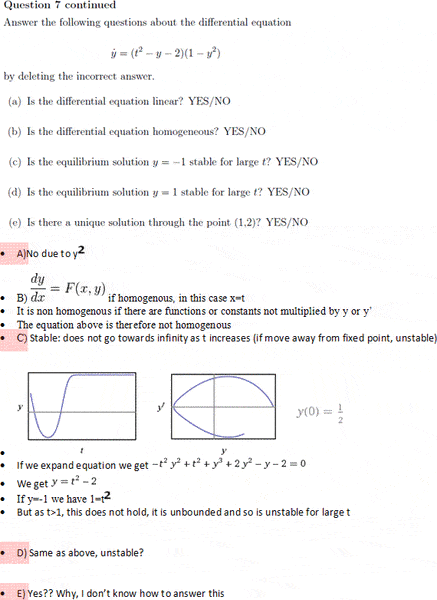
solution above
Homework Statement
Homework Equations
The Attempt at a Solution
solution above