How to relate relativistic kinetic energy and momentum

[greg_rack]

Hi guys,

a special relativity problem requested to choose the right graph representing relativistic momentum $p$ as a function of rel. kinetic energy $K$, from these four:

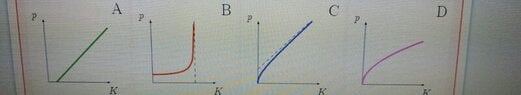
At first, I tried writing $p$ as a function of $K$, in order to then analyze the function's graph and see if it matches one of the four above, being $p=\gamma mv$ and $K=mc^2(\gamma - 1)$, but I couldn't rearrange those two in such a way.
By deduction, I believe the graph should be C or D, since momentum would reasonably tend to infinity in a non-linear way(A) due to the presence of factor $\gamma$, nor as indicated by B...

 

[Gaussian97]

You have an equation for $p(\gamma, v)$ and an equation for $K(\gamma)$, so of course, you cannot use them to find an equation $p(K)$, you need an extra equation, do you know of any equation that relates $\gamma$ and $v$?

 

[greg_rack]

You have an equation for $p(\gamma, v)$ and an equation for $K(\gamma)$, so of course, you cannot use them to find an equation $p(K)$, you need an extra equation, do you know of any equation that relates $\gamma$ and $v$?

Aren't $\gamma$ and $v$ simply related by $\gamma$'s definition $\gamma=\frac{1}{\sqrt{1-\beta ^2}}$, being $\beta$ the velocity in terms of $c$?

 

[Ibix]

Aren't $\gamma$ and $v$ simply related by $\gamma$'s definition $\gamma=\frac{1}{\sqrt{1-\beta ^2}}$, being $\beta$ the velocity in terms of $c$?

Yes. So you should be able to write $p(v)$ and $K(v)$ and hence $K(p)$.

Alternatively, do you know anything about $E^2$ and $p^2c^2$?

 

[greg_rack]

Alternatively, do you know anything about $E^2$ and $p^2c^2$?

Yes, $E^2=p^2c^2+m^2c^{4}$.
Rearranging, it indeed gets $p(E)=\frac{1}{c}\sqrt{E^2-E_0^2}$, hence:
$$p(K)=\frac{1}{c}\sqrt{K(K+2E_0)}$$
which corresponds to graph C :)