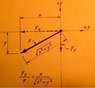
- #1
Vanessa Avila
- 94
- 1
Homework Statement
Each one of the force vectors are surrounded with dimensions. But you're not given any angles. How do you solve for the resultant vector like that?
Homework Equations
Fr = F1 + F2 + F3
The Attempt at a Solution
I tried taking the arctan(200/210) on the 435 N vector to get the angle for that but I don't think that's right.