HP 50g calculator's answer is correct or author's answer is correct?

WMDhamnekar · May 25, 2022

Summary: Evaluate ##\displaystyle\iint\limits_R e^{\frac{x-y}{x+y}} dA ## where ##R {(x,y): x \geq 0, y \geq 0, x+y \leq 1}##

Author has given the answer to this question as ## \frac{e^2 -1}{4e} =0.587600596824 ## But hp 50g pc emulator gave the answer after more than 11 minutes of time 1.11888345561.

Calculator screen showing a double integral from 0 to 1 in X and 0 to 1 in Y of e raised to (X-Y)/(X+Y), with differentials dX dY, and soft-key labels TEXT and OK at the bottom.

HP 50g calculator screen showing a double integral from 0 to 1 of e raised to (X minus Y) over (X plus Y), with dX dY, and the numerical result 1.11888345561.

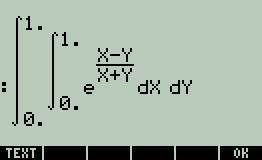
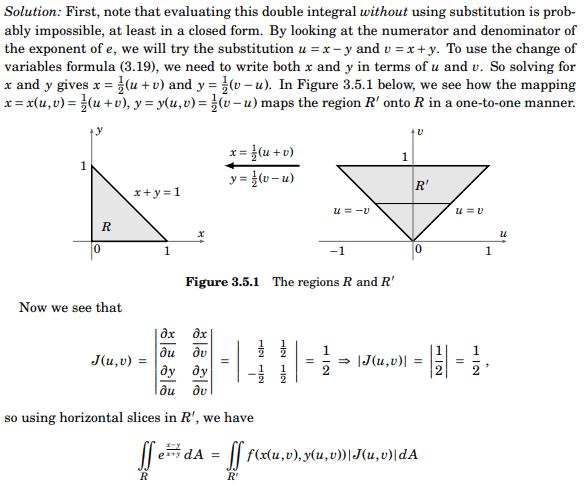
Author's answer

Handwritten-style solution figure: triangular region R with vertices at 0, 1 on the x-axis and y-axis bounded by x + y = 1, next to a transformed region R' bounded by u = -v and u = v with v up to 1, and the Jacobian computed as 1/2.

Handwritten-style worked derivation of a double integral of e^((x-y)/(x+y)) over region R, changed to variables u and v, evaluated step by step to (e^2 - 1)/(4e).

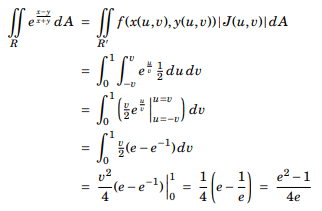Now, How to decide which answer is correct?

Mark44 · May 25, 2022

Your limits of integration are wrong on the inner integral. The integral you entered into the simulator has the region R being ##\{(x, y) | 0 \le x \le 1, 0 \le y \le 1 \}##. IOW, the square bounded by the lines x = 0, y = 0, x = 1, and y = 1. This is incorrect, since the region of integration is a triangle.

Integrating with respect to y first, your integral should look like this:
$$\int_{x=0}^1\int_{y=0}^{1 - x} e^{\frac{x-y}{x+y}}dy dx$$

HP 50g calculator's answer is correct or author's answer is correct?

Discussion

Similar threads