Lambda96
- 233
- 77
- Homework Statement
- show that $$ \Delta S \geqslant 0 $$
- Relevant Equations
- none
Hi,
Unfortunately, I have problems with the task 4
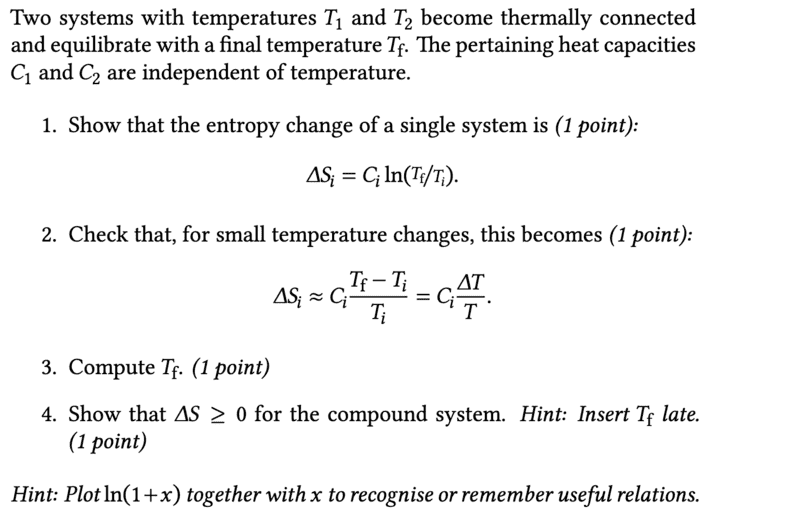
In task 3 I got the following
$$ T_f=T_ie^{\Delta S_i - c_i} $$
Then I proceeded as follows
$$ \Delta S = \Delta S_1 + \Delta S_1 $$
$$ \Delta S =c_1ln(\frac{T_ie^{\Delta S_i - c_i}}{T_1})+c_2ln(\frac{T_f}{T_2})$$
$$ \Delta S =c_1ln(\frac{T_1e^{\Delta S_1 - c_1}}{T_1})+c_2ln(\frac{T_2e^{\Delta S_2 - c_2}}{T_2})$$
$$ \Delta S = c_1ln(e^{\Delta S_1 - c_1}) + c_2*ln(e^{\Delta S_2 -c_2}) $$
$$ \Delta S = c_1*(\Delta S_1 - c_1) + c_2*(\Delta S_2 - c_2) $$
Unfortunately, I can not get any further now
Did I do anything wrong, or am I missing something that I can show that this expression is greater than or equal to 0?
Unfortunately, I have problems with the task 4
In task 3 I got the following
$$ T_f=T_ie^{\Delta S_i - c_i} $$
Then I proceeded as follows
$$ \Delta S = \Delta S_1 + \Delta S_1 $$
$$ \Delta S =c_1ln(\frac{T_ie^{\Delta S_i - c_i}}{T_1})+c_2ln(\frac{T_f}{T_2})$$
$$ \Delta S =c_1ln(\frac{T_1e^{\Delta S_1 - c_1}}{T_1})+c_2ln(\frac{T_2e^{\Delta S_2 - c_2}}{T_2})$$
$$ \Delta S = c_1ln(e^{\Delta S_1 - c_1}) + c_2*ln(e^{\Delta S_2 -c_2}) $$
$$ \Delta S = c_1*(\Delta S_1 - c_1) + c_2*(\Delta S_2 - c_2) $$
Unfortunately, I can not get any further now
Did I do anything wrong, or am I missing something that I can show that this expression is greater than or equal to 0?