I came up with a new theory on adhesive strength

[unscientific]

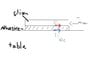
First, I pondered about the concepts of force and pressure, force can't exist without pressure, vice versa. Imagine a an adhesive sandwiched between a tabletop and a silicon wafer. (this is part 1)

Now, when you exert a shearing force F_shear above the adhesive and between the silicon thickness, the adhesive-silicon force exerts an adhesive force opposite in direction to the shearing force. Simultaneously, the adhesive-table force exerts an adhesive force opposite in direction to the shearing force.

This is shown as in diagram 1.

At the point of cohesive failure,

F_shear = F'_si + F'_table

If F'_si > F'_table,
the point of rupture would be the table-adhesive contact

If F'_table > F'_si,
the point of rupture would be the silicon-adhesive contact.

Now, I know that F'_table is less than F_table, where F_table is the TRUE or MAXIMUM adhesive contact force between the adhesive and table.
Furthermore, I know that F'_si is less than F_si, where F_si is the TRUE of MAXIMUM adhesive contact force between the adhesive and silicon.

 

[unscientific]

Now, if the shear force was applied BETWEEN the silicon and the surface of the table, but very close to the surface of the table, the adhesive force between Silicon and adhesive can be IGNORED, as they do not come into play.

Now, looking from the plan view (from Above), I imagine the surface of the adhesive to be composed of tiny segments, each producing a adhesive force to resist the shear force.(diagram2)

Now, here's when i come up with my theory: I imagine the adhesive forces to be composed entirely of springs, where an infinitesimally SMALL and THIN(2d) part of the adhesive surface contains a "spring", that will contribute to part of the adhesive force. Now, when u apply a shear force that is parallel to the surface of the adhesive, the adhesive behaves like springs in parallel, each contributing directly where Fadhesive = kx₁ + kx₂ + kx₃ + kx₄...

Now, i imagine there to be composed of N number of layers of springs, each of 0 thickness, and each layer interacts with each other like springs in parallel. So the first layer in contact with the surface-adhesive will exert a force of nkx where n is the number of springs in a layer. Then the second layer will exert a force of nkx/2, 3rd layer nkx/3, 4th layer is nkx/4 ...until when at the infiniteth layer, that layer does not exert any force opposing F_shear.
This is logical, as u imagine a high TOWER of adhesive, as shown in diagram 3, where the lowest layer(infiniteth) does not contribute any adhesive force.

 

[unscientific]

Now, if the shear force was applied BETWEEN the silicon and the surface of the table, but very close to the surface of the table, the adhesive force between Silicon and adhesive can be IGNORED, as they do not come into play.

Now, looking from the plan view (from Above), I imagine the surface of the adhesive to be composed of tiny segments, each producing a adhesive force to resist the shear force.(diagram2)

Now, here's when i come up with my theory: I imagine the adhesive forces to be composed entirely of springs, where an infinitesimally SMALL and THIN(2d) part of the adhesive surface contains a "spring", that will contribute to part of the adhesive force. Now, when u apply a shear force that is parallel to the surface of the adhesive, the adhesive behaves like springs in parallel, each contributing directly where Fadhesive = kx₁ + kx₂ + kx₃ + kx₄...kx_n (Where n = no. of springs in each layer)

Section 2: "So its all comes down to layers.."

Now, i imagine there to be composed of p number of layers, each of 0 thickness, and each layer interacts with each other like springs in parallel. Let there be N number of springs interacting between 2 layers, each with a "stiffness" constant of k_layer. So the first layer in contact with the surface-adhesive will exert a force of nk_layerx where n is the number of springs within a layer.

So, the TOTAL "spring-adhesive" forces in layer 1 is just nkx, between layer 1 and layer 2 is Nk_layerx_layer (springs between layer 1 and 2 are in series), layer2-3 is Nk_layerx_layer/2, layer 3-4 is Nk_layerx_layer/3, until the infiniteth layer its 0 (the base of an infinitely long series of springs does not contribute any restoring force.

Therefore, the total "adhesive" forces = nkx + Nk_layerx_layer (1 + 1/2 + 1/3 + 1/4 + 1/5 + ...1/(p-1))

 

[Mapes]

Unfortunately for your hypothesis, a simple free-body diagram of the adhesive region in your diagram shows that F'_si = -F'_table. So your inequalities don't make much sense.

We already have a theory describing adhesion; it involves molecular bonding. It describes the mechanical response to shear loading. It describes deadhesion by crack propagation. It even describes the http://arjournals.annualreviews.org/doi/abs/10.1146/annurev.biophys.30.1.105" . I recommend checking it out.

EDIT: I'm assuming both forces, as drawn, act on the adhesive. In any case, their magnitudes (if not their directions) are equal, as shown by a free-body diagram.

 

[unscientific]

they are in the same direction, check out diagram 1.

 

[russ_watters]

Sorry, this isn't a forum for developing new theories.